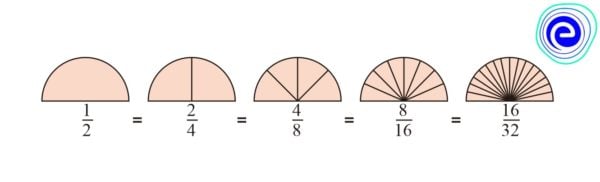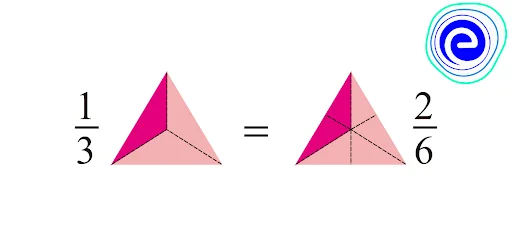Equivalent Fractions: Definition, Methods & Examples

Equivalent Fractions: A fraction represents a part of the whole. Fractions that represent the same value but may have different numerators and denominators are known as equivalent fractions. For example, \(\frac{2}{4}\) and \(\frac{5}{10}\) are equal to the value \(\frac{1}{2}\). So, \(\frac{2}{4}\) and \(\frac{5}{10}\) are called equivalent fractions. In this article, we shall discuss equivalent fractions.

Equivalent Fraction: Definition

The numbers of the form \(\frac{a}{b}\) where \(a\) and \(b\) are non-zero integers, which are called fractions. Equivalent fractions are defined as fractions that have the same value, regardless of their numerators or denominators.

Here, \(a{\mkern 1mu} \to \) Numerator and \(b{\mkern 1mu} \to \) Denominator.

Examples: \(\frac{2}{4},\,\,\frac{5}{{10}},\,\frac{1}{4},\,\frac{3}{{17}}…\) are fractions.

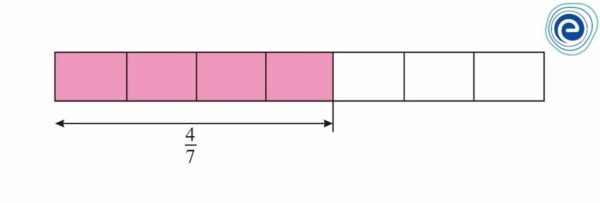
The above figure has been divided into \(7\) equal parts. Out of these \(7\) equal parts, \(4\) parts are shaded. Thus, the shaded portion represents four-sevenths.

Numerically, it is denoted as \(\frac{4}{7}\).

Here, \(\frac{4}{7}\) is a fraction and four – sevenths is a fractional number.

Types of Fractions:

There are \(6\) different types of fractions:

\(1\). Proper Fractions

A fraction whose numerator is less than its denominator is called a proper fraction.
Examples: \(\frac{2}{4},\,\,\frac{5}{{10}},\,\frac{1}{4},\,\frac{3}{{17}}\) etc., are all proper fractions.
Note: Each proper fraction is less than \(1.\)

\(2\). Improper Fractions

A fraction whose numerator is greater than its denominator is called an improper fraction.
Examples: \(\frac{5}{4},\,\,\frac{{13}}{{10}},\,\frac{{11}}{7},\,\frac{{23}}{{17}}\) etc., are all improper fractions.

\(3\). Mixed Fractions

A combination of a whole number and a proper fraction is called a mixed fraction.
Examples:\(1\frac{2}{4},\,\,3\frac{5}{{10}},\,5\frac{1}{4},6\,\frac{3}{{17}}\), etc., are all mixed fractions.

\(4\). Like Fractions

The group of two or more fractions with the same denominators is like fractions.
Examples: \(\frac{1}{2},\frac{2}{2},\frac{3}{2},\frac{4}{2},\frac{5}{2},\) etc., are all like fractions.

\(5\). Unlike Fractions

The group of two or more fractions with different denominators is called Unlike Fractions.
Examples: \(\frac{1}{2},\frac{2}{4},\frac{3}{5},\frac{4}{7},\frac{5}{8},\) etc., are all unlike fractions.

\(6\). Unit Fractions

A unit fraction is any fraction with \(1\) as its numerator and a whole number for the denominator.
Examples: \(\frac{1}{2},\frac{1}{4},\frac{1}{5},\frac{1}{7},\frac{1}{8},\) etc., are all unit fractions.
Note: The number \(1\) which can be written as \(\frac{1}{1}\) has its numerator and denominator equal, is also called a unit fraction.

What are Equivalent Fractions?

Two or more fractions representing the same part of a whole are called equivalent fractions.

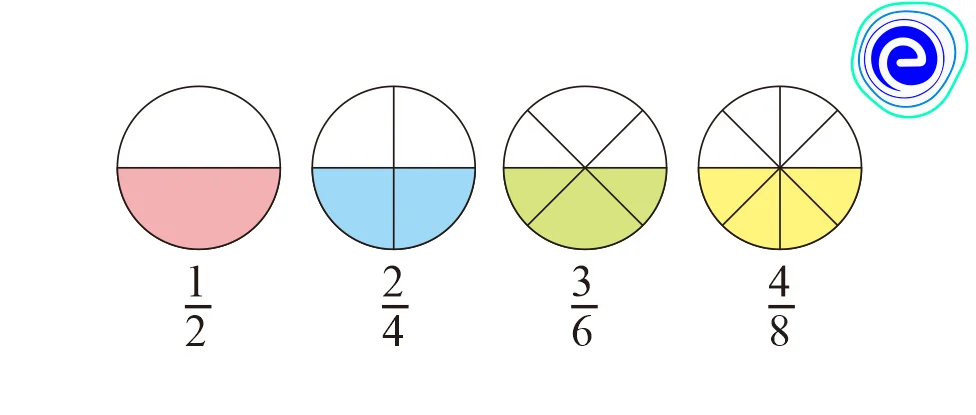
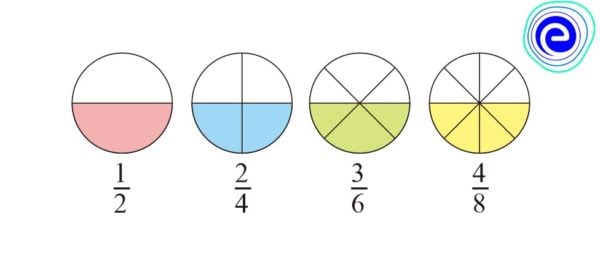
\(\frac{1}{2} = \frac{2}{4} = \frac{3}{6} = \frac{4}{8} = \frac{5}{{10}}\) these fractions are called equivalent fractions.

Note that,\(\frac{1}{2} = \frac{{1 \times 2}}{{2 \times 2}} = \frac{{1 \times 3}}{{2 \times 3}} = \frac{{1 \times 4}}{{2 \times 4}} = \frac{{1 \times 5}}{{2 \times 5}}\)

It shows that multiplying the numerator and the denominator of a fraction by the same non-zero number does not change the value of the fraction.

Similarly,\(\frac{{2 \div 2}}{{4 \div 2}} = \frac{{3 \div 3}}{{6 \div 3}} = \frac{{4 \div 4}}{{8 \div 4}} = \frac{{5 \div 5}}{{10 \div 5}} = \frac{1}{2}\)

A fraction’s value remains unchanged when it is divided by the same non-zero number in both the numerator and the denominator.

Rule of Equivalent Fractions:

To get a fraction equivalent to a given fraction, we multiply or divide the numerator and the denominator of the given fraction by the same non-zero number.

Example: The equivalent fraction for \(\frac{3}{6}\)

\(\frac{{3 \times 2}}{{6 \times 2}} = \frac{6}{{12}}\) is the equivalent fraction for \(\frac{3}{6}\)

\(\frac{{3 \div 3}}{{6 \div 3}} = \frac{1}{2}\) is the equivalent fraction for \(\frac{3}{6}\)

Equivalent Fractions Examples:

Here are few pictorial examples for equivalent fractions.

Numerical examples for equivalent fractions are,

1. \(\frac{1}{3} = \frac{2}{6} = \frac{3}{9} = \frac{4}{{12}} = \frac{5}{{15}}\)
2. \(\frac{1}{2} = \frac{2}{4} = \frac{3}{6} = \frac{4}{8} = \frac{5}{{10}}\)
3. \(\frac{{100}}{{20}} = \frac{{50}}{{10}} = \frac{5}{1} = \frac{{20}}{4} = \frac{{40}}{8}\)

Different Methods to Determine if Two Fractions are Equivalent?

We should simplify the given fractions to find whether they are equivalent fractions or not. Simplification to get equivalent fractions can be done until both the numerator and denominator should still be non-zero integers.

There are various methods to check if the given fractions are equivalent or not:

1. Making the denominators the same
2. Finding the decimal form of both the fractions
3. Cross multiplication method
4. Visual method

\(1\). Making the Denominators the Same:

The denominators of the fractions, \(\frac{3}{9}\) and \(\frac{6}{{18}}\) are \(9\) and \(18.\) The least common multiple \({\rm{LCM}}\) of the denominators \(9\) and \(18\) is \(18.\) Now make the denominators of both fractions \(18\) by multiplying them with suitable numbers.
So, \(\frac{3}{9} = \frac{{3 \times 2}}{{9 \times 2}} = \frac{6}{{18}}\)
And, \(\frac{6}{{18}} = \frac{{6 \times 1}}{{18 \times 1}} = \frac{6}{{18}}\)
Note that both the fractions are equivalent to the same fraction \(\frac{6}{{18}}\) Thus, the given fractions are equivalent.
Note: If the fractions are not equivalent fractions, we can check the greater or smaller fraction by looking at the numerator of both the resultant fractions. Hence, this method can also be used for compairing fractions.

\(2\). Finding the Decimal form of both the Fractions:

Now we can find the decimal form of both the fractions,\(\frac{3}{9}\) and \(\frac{6}{{18}}\) to check if they give the same value.
We see, \(\frac{3}{9} = 0.33333…\)
And, \(\frac{6}{18} = 0.33333…\)
The decimal values of both the fractions are the same, i.e.,\(0.33333…\)
Therefore, \(\frac{3}{9}\) and \(\frac{6}{18}\) are equivalent fractions.

\(3\). Cross Multiplication Method

To test whether two given fractions are equivalent or not,
let \(\frac{a}{b}\) and \(\frac{c}{d}\) be two given fractions.
Cross multiply the given fractions \(\frac{a}{b}\) and \(\frac{c}{d}\) as follows: \(a \times d\) and \(b \times c\)
If \(ad\, = \,bc\) we say that \(\frac{a}{b}\) and \(\frac{c}{d}\) are equivalent fractions, otherwise not.
To check whether \(\frac{3}{9}\) and \(\frac{6}{{18}}\) are equivalent, we cross multiply the given fractions. If both the products are the same, then the fractions are equivalent fractions.
We see, \(3 \times 18 = 54\) and \(6 \times 9 = 54\)
Note that both the obtained products are \(54\) so the given fractions are equivalent fractions.

\(4\). Visual Method

Let us represent each of the fractions \(\frac{1}{3}{\mkern 1mu}\) and \(\frac{2}{6}\) pictorially on identical shapes and can identify if the shaded portions of both are equal or not.

We can see that the shaded portions of both the triangles are equal. Hence, the given fractions are equivalent fractions. 

Simplest Form of a Fraction

A fraction is said to be in the simplest form if the \({\rm{HCF}}\) of its numerator and denominator is \(1.\)
Let the given fraction be \(\frac{a}{b}\) and the \({\rm{HCF}}\) of \(a\) and \(b\)
Then, \(\frac{a}{b} = \frac{{a \div h}}{{b \div h}}\) is the simplest form.
Example: The simplest form of \(\frac{8}{{12}}\)
Now, \(\frac{{8 \div 4}}{{12 \div 4}} = \frac{1}{3}\)
So, the simplest form of \(\frac{8}{{12}}\) is \(\frac{1}{3}\)

Solved Examples – Equivalent Fractions

Q.1. Are the fractions \(\frac{2}{7}\) and \(\frac{4}{{12}}\) equivalent?
Ans: Given fractions are \(\frac{2}{7}\) and \(\frac{4}{12}\)
We see \(2 \times 12 = 7 \times 4\)
\(\Rightarrow 24 \ne 28\)
 Therefore, \(\frac{3}{9}\) and \(\frac{4}{12}\) are not equivalent fractions.

Q.2. Are the fractions, \(\frac{9}{7}\) and \(\frac{6}{4}\) equivalent?
Ans: Given fractions are \(\frac{9}{7}\) and \(\frac{6}{4}\)
We see,  \(9 \times 4 = 6 \times 7\)
\(\Rightarrow 36 \ne 42\)
Therefore, \(\frac{9}{6}\) and \(\frac{6}{4}\) are not equivalent fractions.

Q.3. Write fraction equivalent to \(\frac{3}{4}\) with numerator\(15\)
Ans: The given fraction is \(\frac{3}{4}\) which have the numerator \(15\).
Here, we need to multiply both the numerator and the denominator with \(5\).
So, we have, \(\frac{{3 \times 5}}{{4 \times 5}} = \frac{{15}}{{20}}\)
Therefore, the equivalent fraction for \(\frac{3}{4}\) with numerator \(15\) is \(\frac{{15}}{{20}}\).

Q.4. Write \(4\) equivalent fractions for \(\frac{5}{6}.\) 
Ans: The given fraction is \(\frac{5}{6}.\)The four equivalent fractions are,
\( \Rightarrow \frac{{5 \times 2}}{{6 \times 2}} = \frac{{10}}{{12}}\)
\( \Rightarrow \frac{{5 \times 3}}{{6 \times 3}} = \frac{{15}}{{18}}\)
\( \Rightarrow \frac{{5 \times 4}}{{6 \times 4}} = \frac{{20}}{{24}}\)
\( \Rightarrow \frac{{5 \times 5}}{{6 \times 5}} = \frac{{25}}{{30}}\)
Therefore, \(\frac{{10}}{{12}},\frac{{15}}{{18}},\frac{{20}}{{24}},\frac{{25}}{{30}}\) are four equivalent fractions of \(\frac{5}{6}.\)

Q.5. Write fraction equivalent to \(\frac{{36}}{{63}}\) with numerator \(4\).
Ans: The given fraction is \(\frac{{36}}{{63}}\)
Here, we need to divide both the numerator and the denominator by \(9\).
So, we get, \(\frac{{36 \div 9}}{{63 \div 9}} = \frac{4}{7}\)
Therefore, the equivalent fraction for \(\frac{{36}}{{63}}\) with numerator \(4\) is \(\frac{4}{7}\)

Q.6. Write \(4\) equivalent fractions for \(\frac{3}{7}.\)
Ans: The given fraction is \(\frac{3}{7}.\) The four equivalent fractions are,
\( \Rightarrow \frac{{3 \times 3}}{{7 \times 3}} = \frac{9}{{21}}\)
\( \Rightarrow \frac{{3 \times 7}}{{7 \times 7}} = \frac{{21}}{{49}}\)
\( \Rightarrow \frac{{3 \times 4}}{{7 \times 4}} = \frac{{12}}{{28}}\)
\( \Rightarrow \frac{{3 \times 5}}{{7 \times 5}} = \frac{{15}}{{35}}\)
Therefore, \(\frac{9}{{21}},\frac{{21}}{{49}},\frac{{12}}{{28}},\frac{{15}}{{35}}\) are four equivalent fractions of \(\frac{3}{7}.\)

Summary

Two or more fractions representing the same part of a whole are equivalent fractions. To get a fraction equivalent to a given fraction, we multiply or divide the numerator and the denominator of the given fraction by the same non-zero number. In this article, we have learnt in detail about fractions, types of fractions, equivalent fractions, and how to check the equivalent fractions.

Frequently Asked Questions About Equivalent Fractions

Let’s look at some of the commonly asked questions about Equivalent Fractions:

Q.1. What is an equivalent fraction, with example?
Ans: Fractions that represent the same value but may have different numerators and denominators are known as equivalent fractions.
\(\frac{1}{2} = \frac{2}{4} = \frac{3}{6} = \frac{4}{8} = \frac{5}{{10}}\) these fractions are called equivalent fractions.

Q.2. What are different types of fractions?
Ans: There are six different types of fractions, they are: Proper Fractions, Improper Fractions, Mixed Fractions, Like Fractions, Unlike Fractions and Unit Fractions.

Q.3. How do you simplify equivalent fractions?
Ans: To simplify a fraction equivalent to a given fraction, we divide the numerator and the denominator of the given by the common, same non-zero number.
Example: The simplest form of equivalent fraction for \(\frac{3}{6}\) is \(\frac{{3 \div 3}}{{6 \div 3}} = \frac{1}{2}\) which is the Equivalent Fraction for \(\frac{3}{6}\).
Therefore, the simplest equivalent fraction for \(\frac{3}{6}\) is \(\frac{1}{2}.\)

Q.4. What fraction is \(\frac{3}{8}\) equivalent to?
Ans: Some of the equivalent fractions for \(\frac{3}{8}\) are
\(\frac{{3 \times 4}}{{8 \times 4}} = \frac{{12}}{{32}}\)
\(\frac{{3 \times 3}}{{8 \times 3}} = \frac{9}{{24}}\)
\(\frac{{3 \times 5}}{{8 \times 5}} = \frac{{15}}{{40}}\) and \(\frac{{3 \times 7}}{{8 \times 7}} = \frac{{21}}{{56}}\)
So, \(\frac{{12}}{{32}},\,\frac{9}{{24}},\,\frac{{15}}{{40}},\,\frac{{21}}{{56}}\) are equivalent to a fraction \(\frac{3}{8}.\)

Q.5. What is \(\frac{3}{5}\) equivalent to as a fraction?
Ans: Some of the equivalent fractions for \(\frac{3}{5}\)
\(\frac{{3 \times 2}}{{5 \times 2}} = \frac{6}{{10}},\frac{{3 \times 3}}{{5 \times 3}} = \frac{9}{5},\frac{{3 \times 4}}{{5 \times 4}} = \frac{{12}}{{20}}\) and \(\frac{{3 \times 5}}{{5 \times 4}} = \frac{{15}}{{25}}\)
So, \(\frac{6}{{10}},\frac{9}{{15}},\frac{{12}}{{20}},\frac{{15}}{{25}}\) are equivalent to a fraction \(\frac{3}{5}\)

Q.6. How to find equivalent fractions?
Ans: Multiplying the numerator and the denominator of a fraction by the same non-zero number does not change the value of the fraction.
We have \(\frac{1}{2} = \frac{{1 \times 2}}{{2 \times 2}} = \frac{{1 \times 4}}{{2 \times 4}} = \frac{{1 \times 5}}{{2 \times 5}}.\)
Similarly, \(\frac{{2 \div 2}}{{4 \div 2}} = \frac{{3 \div 3}}{{6 \div 3}} = \frac{{4 \div 4}}{{8 \div 4}} = \frac{{5 \div 5}}{{10 \div 5}} = \frac{1}{2}\)
It shows that dividing the numerator and the denominator of a fraction by the same non-zero number does not change the value of the fraction.

Q.7. How to test whether two given fractions are equivalent or not?
Ans: Let \(\frac{a}{b}\) and \(\frac{c}{d}\) be two given fractions.
Cross multiply the given fractions \(\frac{a}{b} \times \frac{c}{d}\)
If \(ad = bc,\) we say that \(\frac{a}{b}\) and \(\frac{c}{d}\) are Equivalent Fractions, otherwise not.

We hope that this article about Equivalent Fractions is helpful to you. You can also take Mock Tests at Embibe to improve your Math skills.