Lagrange’s Mean Value Theorem: Statement, Proof, Formulas

Lagrange’s Mean Value Theorem: Lagrange’s mean value theorem is also called the first mean value theorem. It is among the most important tools used to prove many other theorems in differential and integral calculus. Sometimes the mean value theorem is also taught with its particular case, i.e., Rolle’s theorem. Rolle’s theorem states that for the curve between two points, there exists a point where the tangent is parallel to the secant line and passes through these two points of the curve.

Lagrange’s Mean Value Theorem is one of the essential theorems in analysis, and therefore, all its applications have major significance. Some of the applications are Leibniz’s rule, L’ Hospital’s rule, strictly increasing and strictly decreasing functions, and the symmetry of second derivatives.

Statement of Lagrange’s Mean Value Theorem 

If a function \(f\left( x \right)\) is defined in the closed interval \(\left[ {a,\,b} \right]\) such that

(i) \(f\left( x \right)\) is continuous on the closed interval \(\left[ {a,\,b} \right]\)
(ii) \(f\left( x \right)\) is derivable on an open interval \(\left( {a,\,b} \right)\) then there exists at least one point \(x = c,\,c \in (a,\,b)\) such that \(\frac{{f(b) – f(a)}}{{b – a}} = {f^\prime }(c)\).

Proof

Let us consider a function, \(F(x) = f(x) + Ax\,…..(i)\)

where a constant \(A\) is to be determined such that

\(F(a) = F(b)\,…..(ii)\)

Now, \(F(a) = f(a) + Aa\) and \(F(b) = f(b) + Ab\)

Using \((ii)\), we have \(f(a) + Aa = f(b) + Ab\)

\(f(b) – f(a) = – A(b-a)\)

\( \Rightarrow – A = \frac{{f(b) – f(a)}}{{b – a}}\,…..(iii)\)

Now, \(f\left( x \right)\) is given to be continuous on \(\left[ {a,\,b} \right]\), and \(Ax\) being a polynomial in \(x\), is always continuous on \(\left[ {a,\,b} \right]\). Thus \(F\left( x \right)\), the sum of two continuous functions \(f\left( x \right)\) and \(Ax\) is also continuous on \(\left[ {a,\,b} \right]\), and also derivable on \(\left( {a,\,b} \right)\).

Thus, we conclude that \(F\left( x \right) = f\left( x \right) + Ax\) is

• Continuous on the closed interval \(\left[ {a,\,b} \right]\) 
• Derivable on the open interval \(\left( {a,\,b} \right)\).
• \(F(a) = F(b)\)

Therefore, \(F\left( x \right)\) satisfies all three conditions of the Rolle’s theorem and hence there must exist at least one value \(‘c’\) of \(x\) in an open interval \(\left( {a,\,b} \right)\) such that \({F^\prime }(c) = 0\).

Now, \(F(x) = f(x) + Ax\)

\(\therefore \,{F^\prime }(x) = {f^\prime }(x) + A \Rightarrow {F^\prime }(c) = {f^\prime }(c) + A\) 

\({F^\prime }(c) = 0 \Rightarrow {f^\prime }(c) + A = 0 \Rightarrow {f^\prime }(c) = – A\,…..(iv)\)

From \((iii)\) and \((iv)\), we have

\(\frac{{f(b) – f(a)}}{{b – a}} = {f^\prime }(c)\) for some \(c \in (a,\,b)\).

Another Form of Lagrange’s Mean Value Theorem

Statement: If function \(f\) is continuous in a closed interval \(\left[ {a,\,a + h} \right]\) and derivable in the open interval \((a,\,a + h)\), then there exist at least one number \(\theta \in (0,\,1)\), such that \(f(a + h) = f(a) + h{f^\prime }(a + \theta h)\).

Proof

We write \(b – a = h\), so that \(h\) denotes the length of the interval \(\left[ {a,\,b} \right]\).

Now, \([a,\,b] = [a,\,a + h]\) and \(a < c < a + h \Rightarrow c = a + \theta h,\,0 < \theta < 1\).

\(\therefore \,\frac{{f(a + h) – f(a)}}{h} = {f^\prime }(a + \theta h)\)

Note: Rolle’s theorem is a special case of Lagrange’s Mean Value Theorem (LMVT).

Hence, by taking \(f(b) = f(a)\), we get \({f^\prime }(c) = 0\).

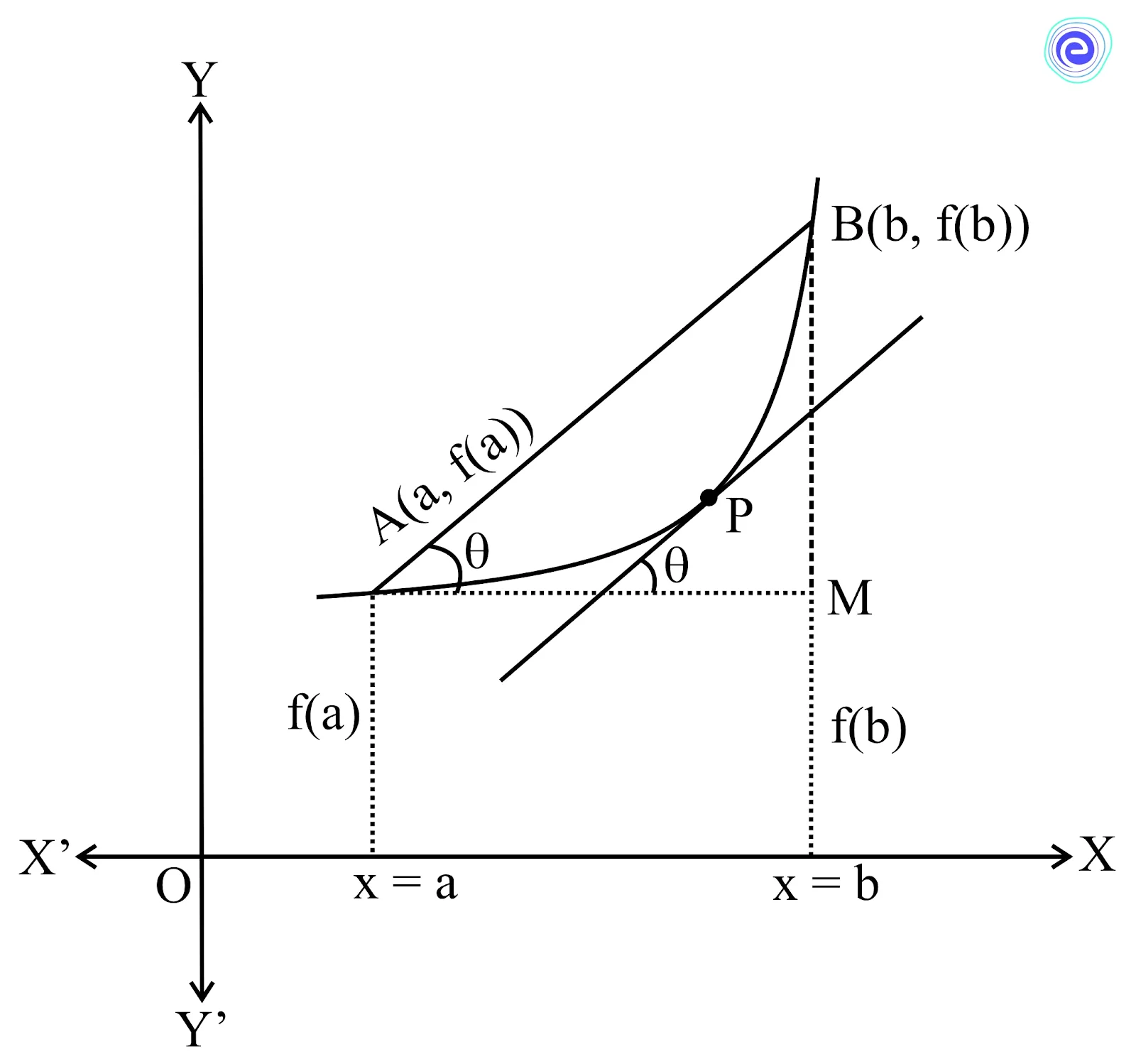
Geometrical Interpretation

Let \(APB\) be the curve represented by the function \(f(x)\) 

The conditions of Lagrange’s mean value theorem imply that:

• \(f(x)\) is continuous on a closed interval \(\left[ {a,\,b} \right]\), i.e., the curve \(y = f(x)\) has no break from the point \(A\left( {a,\,f\left( a \right)} \right)\) to the point \(B\left( {b,\,f\left( b \right)} \right)\).
• \(f(x)\) is derivable on the open interval \(\left( {a,\,b} \right)\), i.e., there exists a non-vertical tangent at every point in \(\left( {a,\,b} \right)\).

Note: Tangent may be vertical at one or both of the endpoints \(A\) and \(B\)

Geometrically, the mean value theorem asserts that there exists at least one point on the curve from \(A\) to \(B\), where the tangent is parallel to the chord through \(A\) and \(B\), because the slope of chord \(AB = \frac{{f(b) – f(a)}}{{b – a}}\).

Therefore, in geometrical form, Lagrange’s mean value theorem can be stated as:

Let \(f(x)\) be a function defined on \([a,\,b]\) such that \(y = f(x)\) is a continuous curve between points \(A(a,\,f(a))\) and \(B(b,\,f(b)\) and at each point on the curve, except at the endpoints, it is possible to draw a unique tangent. A point on the curve exists such that the tangent (at that point) is parallel to the chord joining the endpoints.

Note:

1. Now \(f(b) – f(a)\) is the change in the function \(f\) as \(x\) changes from \(a\) to \(b\), so that \(\frac{{f(b) – f(a)}}{{b – a}}\) is the rate of change of the function over \([a,\,b]\). Also, \({f^\prime }(c)\) is the actual rate of change of the function at \(x = c\). Hence, as per the theorem, the average rate of change of a function over an interval is the same as the actual rate of change of the function for \(x = c\). In particular, for instance, the average velocity of a particle over an interval of time is equal to the velocity at some point instantly belonging to the interval.
2. This interpretation of the theorem justifies the name “Mean Value” for the theorem. The two hypotheses of LMVT are only a set of sufficient conditions and by no means necessary for its conclusion.

Deduction From Mean Value Theorem

1. Let \({f^\prime }(x) = 0\) when \(a \le x \le b\), then
   \(f(x) = f(a)\forall x \in [a,\,b]\)
2. If \(\frac{d}{{dx}}(F(x)) = f(x) = \frac{d}{{dx}}(G(x))\forall x \in [a,\,b]\), then \(F(x)\) and \(G(x)\) differ by a constant.
3. The mean value theorem is used to estimate the size of functions and make numerical approximations.
4. If \({f^\prime }(x)\) exists for \(a < x \le b\) and \(|f(x)| \to \infty \) as \(x \to {a^ + }\) then \(\left| {{f^\prime }(x)} \right| \to \infty \) as \(x \to {a^ + }\).
5. If \(f\) is continuous at \(c\), and if \(\mathop {\lim }\limits_{x \to c} {f^\prime }(x) = 1\), then \(f\) is derivable at \(c\), and \(l\) is the derivative there.

Solved Examples – Lagrange’s Mean Value Theorem

Q.1. Verify the Lagrange’s mean value theorem for the function \(f(x) = 2x – {x^2}\) in \(\left[ {0,\,1} \right]\).
Ans:
Here \(f(x) = 2x – {x^2}\)
\(f(x)\) being a polynomial function of \(x\), is continuous for all the real \(x\).
\(\therefore \,f(x)\) is continuous on \([0,\,1]\)
\({f^\prime }(x) = 2 – 2x\), which exist uniquely for \(x \in (0,\,1)\) Thus \(f(x)\) is derivable on \((0,\,1)\).
Thus, both the conditions of Lagrange’s mean value theorem are satisfied.
Hence, there must exist at least one point \(c \in (a,\,b)\) such that
\(\frac{{f(b) – f(a)}}{{b – a}} = {f^\prime }(c)\) where \(a = 0,\,b = 1\,…..(1)\)
Now, \({f^\prime }(x) = 2 – 2x\)
\( \Rightarrow {f^\prime }(c) = 2 – 2c\)
\(f(a) = f(0) = 0\) and \(f(b) = f(1) = 2 – 1 = 1\)
\(\therefore \) From \((1),\,\frac{{1 – 0}}{{1 – 0}} = 2 – 2c\)
\( \Rightarrow 2 – 2c = 1\)
\(\therefore \,c = \frac{1}{2} \in (0,\,1)\)
Thus, Lagrange’s mean value theorem is verified.

Q.2. Verify the Lagrange’s mean value theorem for the function \(f(x) = \left( {x – 3} \right)(x – 6)(x – 9)\) in \([3,\,5]\).
Ans:
Here, \(f(x) = (x – 3)(x – 6)(x – 9) = {x^3} – 18{x^2} + 99x – 162\)
\(f(x)\) is a polynomial function of \(x\), and it is continuous for all the real numbers.
\(\therefore \,f(x)\) is continuous on \([3,\,5]\)
\({f^\prime }(x) = 3{x^2} – 36x + 99\), which exists uniquely for \(x \in (3,\,5)\) 
Thus, \(f(x)\) is derivable on \((3,\,5)\).
Hence, both the conditions of Lagrange’s mean value theorem are satisfied.
Therefore, there must exist at least one point \(c \in (a,\,b)\) such that
\(\frac{{f(b) – f(a)}}{{b – a}} = {f^\prime }(c)\) where \(a = 3,\,b = 5\,…..(1)\)
Now, \({f^\prime }(x) = 3{x^2} – 36x + 99\)
\( \Rightarrow {f^\prime }(c) = 3{c^2} – 36c + 99\)
\(f(a) = f(3) = (0)( – 3)( – 6)\) and \(f(b) = f(5) = 8\)
\(\therefore \) From \((1),\,\frac{{8 – 0}}{{5 – 3}} = 3{c^2} – 36c + 99\)
\( \Rightarrow 3{c^2} – 36c + 99 = 4\)
\( \Rightarrow 3{c^2} – 36c + 95 = 0\)
\( \Rightarrow c = 6 \pm \sqrt {\frac{{13}}{3}} \)
\( \Rightarrow c = 6 – \sqrt {\frac{{13}}{3}} \)
\(\therefore \,c = 6 – \sqrt {\frac{{13}}{3}} \in (3,\,5)\)
Thus, Lagrange’s mean value theorem is verified.

Q.3. Examine the validity of Lagrange’s mean value theorem for the function \(f(x) = \sin \,x\) in \(\left[ {\frac{\pi }{2},\,\frac{{5\pi }}{2}} \right]\).
Ans:
Here, \(f(x) = \sin \,x\)
The given function is continuous for all \(x\).
\(\therefore \,f(x)\) is continuous on \(\left[ {\frac{\pi }{2},\,\frac{{5\pi }}{2}} \right]\).
\({f^\prime }(x) = \cos \,x\), which is finite, definite and real values of \(x\).
[\(\because \,\cos \,\theta \) is real and lies between \( – 1\) and \(1\)]
\(\therefore \,f(x)\) is derivable on \(\left( {\frac{\pi }{2},\,\frac{{5\pi }}{2}} \right)\).
Thus, both the conditions of Lagrange’s mean value theorem are satisfied. Hence, there must exist at least one point \(c \in (a,\,b)\) such that \(\frac{{f(b) – f(a)}}{{b – a}} = {f^\prime }(c)\) where \(a = \frac{\pi }{2},\,b = \frac{{5\pi }}{2}\,……(1)\)
Now, \({f^\prime }(x) = \cos \,x \Rightarrow {f^\prime }(c) = \cos \,c\) 
\(f(a) = f\left( {\frac{\pi }{2}} \right) = \sin \frac{\pi }{2} = 1\) and \(f(b) = f\left( {\frac{{5\pi }}{2}} \right) = \sin \frac{{5\pi }}{2}\)
\(\therefore \) From \((1),\,\frac{{1 – 1}}{{\frac{5\pi }{2} – \frac{\pi }{2}}} = \cos \,c\)
\( \Rightarrow \cos \,c = 0\)
\( \Rightarrow C = \frac{\pi }{2},\,\frac{{3\pi }}{2},\frac{{5\pi }}{2},\,\frac{{7\pi }}{2} \ldots \)
\(\therefore \,C = \frac{{3\pi }}{2} \in \left( {\frac{\pi }{2},\,\frac{{5\pi }}{2}} \right)\).
Hence, Lagrange’s mean value theorem is verified.

Q.4. If \(f(x)\) and \(g(x)\) are continuous on \([a,\,b]\) and derivable in \((a,\,b)\) then show that
\(\left| {\begin{array}{*{20}{l}}{f(a)}&{f(b)}\\{g(a)}&{g(b)}\end{array}} \right| = (b – a)\left| {\begin{array}{*{20}{l}}{f(a)}&{{f^\prime }(c)}\\{g(a)}&{{g^\prime }(c)}\end{array}} \right|\), where \(a < c < b\).
Ans:
Consider the function
\(F(x) = \left| {\begin{array}{*{20}{l}}{f(a)}&{f(b)}\\{g(a)}&{g(b)}\end{array}} \right|\) which is continuous on \([a,\,b]\) and differentiable in \(\left( {a,\,b} \right)\).
Thus, both the conditions of Lagrange’s mean value theorem are satisfied. Hence there must exist at least one point \(c \in (a,\,b)\) such that
\(\frac{{F(b) – F(a)}}{{b – a}} = {F^\prime }(c)\)
\( \Rightarrow F(b) – F(a) = (b – a){F^\prime }(c)\)
Since, \(F(a) = 0\), we have \(F(b) = (b – a){F^\prime }(c)\).
\(\therefore \,\left| {\begin{array}{*{20}{l}}{f(a)}&{f(b)}\\{g(a)}&{g(b)}\end{array}} \right| = (b – a)\left| {\begin{array}{*{20}{l}}{f(a)}&{{f^\prime }(c)}\\{g(a)}&{{g^\prime }(c)}\end{array}} \right|\)
Hence, proved.

Q.5. Find a point on the curve \(y = {x^3} – 3x\), where the tangent is parallel to the chord joining \((1,\, – 2)\) and \((2,\,2)\).
Ans:
Let \(y = f(x) = {x^3} – 3x\)
\(f(x)\) being a polynomial function of \(x\), is continuous on \([1,\,2]\).
Also, \({f^\prime }(x) = 3{x^2} – 3\), which exist uniquely for \(x \in (1,\,2)\) Thus \(f(x)\) is derivable on \((1,\,2)\). Thus, both the conditions of Lagrange’s mean value theorem are satisfied. Hence there must exist at least one point \(c \in (a,\,b)\) such that
\(\frac{{f(b) – f(a)}}{{b – a}} = {f^\prime }(c)\) where \(a = 1,\,b = 2\,…..(1)\)
Now, \({f^\prime }(x) = 3{x^2} – 3 \Rightarrow {f^\prime }(c) = 3{c^2} – 3\) 
\(f(a) = f(1) = – 2\) and \(f(b) = f(2) = 2\)
\(\therefore \) From \((1),\,\frac{{2 – ( – 2)}}{{2 – 1}} = 3{c^2} – 3\)
\( \Rightarrow 3{c^2} – 3 = 4\)
\( \Rightarrow 3{c^2} = 7\)
\( \Rightarrow c = \pm \sqrt {\frac{7}{3}} \)
Now, \( \Rightarrow c = \sqrt {\frac{7}{3}} \in (1,\,2)\)
\(\therefore \,x = \sqrt {\frac{7}{3}} \)
\( \Rightarrow y = – 1\).
From \({\rm{ (1),}}\,\frac{{f(2) – f(1)}}{{2 – 1}} = {f^\prime }\left( {\sqrt {\frac{7}{3}} } \right)\)
\(\therefore \) Slope of chord joining \((1,\,f(1))\) and \((2,\,f(2))\) i.e. \((1,\, – 2)\) and \((2,\,2) = \) slope of the tangent at \(\left( {\sqrt {\frac{7}{3}} ,\, – 1} \right)\).

Summary

Lagrange’s mean value theorem states that for a curve between two points, there exists a point where the tangent is parallel to the secant line passing through these two points. If a function \(f\left( x \right)\) is defined in the closed interval \(\left[ {a,\,b} \right]\) such that \(f\left( x \right)\) is continuous on the closed interval \(\left[ {a,\,b} \right]\) and derivable on an open interval \(\left( {a,\,b} \right)\), then there exists at least one point \(x = c,\,c \in (a,\,b)\) such that \(\frac{{f(b) – f(a)}}{{b – a}} = {f^\prime }(c)\). Geometrically it is stated as: Let \(f\left( x \right)\) be a function defined on \(f\left( x \right)\) such that \(\left[ {a,\,b} \right]\) is a continuous curve between points \(A(a,\,f(a))\) and \(B(b,\,f(b)\) then there exists a point on the curve such that the tangent at that point is parallel to chord joining the endpoints of the curve.

Frequently Asked Questions (FAQs)

The frequently asked questions about Lagrange’s Mean Value Theorem are given below:

Q.1. What is the mean value theorem formula?
Ans: \(f\left( x \right)\) is continuous on the closed interval \(\left[ {a,\,b} \right]\) and is derivable on an open interval \(\left( {a,\,b} \right)\) then there exists at least one point \(x = c,\,c \in (a,\,b)\) such that
\(\frac{{f(b) – f(a)}}{{b – a}} = {f^\prime }(c)\)

Q.2. Is the mean value theorem and Lagrange mean value theorem the same?
Ans: Yes, Lagrange’s mean value theorem is the mean value theorem, also called the first mean value theorem.

Q.3. What is \(C\) in Lagrange’s mean value theorem?
Ans: If \(f(x)\) is a function defined on \([a,\,b]\) then \(c\) can be any value of \(x\) such that \(c \in \left( {a,\,b} \right)\).

Q.4. At which condition Lagrange’s mean value theorem becomes Rolle’s theorem?
Ans: If \(f(x)\) is a function defined on \([a,\,b]\) and when the value of \(f\left( a \right) = f\left( b \right)\), then Lagrange’s mean value theorem becomes Rolle’s theorem.

Q.5. How do you find the value of \(c\) that satisfies the Mean Value Theorem?
Ans: If a function \(f(x)\) is defined in the closed interval \([a,\,b]\) such that \(f(x)\) is continuous on the closed interval \([a,\,b]\) and derivable on an open interval \(\left( {a,\,b} \right)\), then there exists at least one point \(x = c,\,c \in (a,\,b)\) such that \(\frac{{f(b) – f(a)}}{{b – a}} = {f^\prime }(c)\).
Hence, we find the value of \(c\) using the equation \(\frac{{f(b) – f(a)}}{{b – a}} = {f^\prime }(c)\).

ATTEMPT MOCK TESTS ON EMBIBE

We hope this article has provided you with the necessary information on Lagrange’s Mean Value Theorem. If you have any doubts feel to drop a comment in the comment box below and we will get back to you. For more such articles, stay tuned to Embibe.