Exams CBSE Prime Numbers- Know Types, Methods, and Properties

Written By Vaibhav_Raj_Asthana
Last Modified 10-03-2023

Prime Numbers- Know Types, Methods, and Properties

What are Prime Numbers? There are lots of definitions of Prime Numbers and each one may be correct in its own way. However, here is the definition given in NCERT books is this – “The numbers other than 1 whose only factors are 1 and the number itself are called Prime Numbers“. A natural number greater than 1 that is not prime is called a composite number. We can check whether a number is prime or composite using the prime factorisation. Read this article to learn how to check whether a number is prime or composite and get the list of prime numbers from 1 to 1000.

What is a Prime Number?

Going by the easiest and most effective definition, prime numbers can be defined as the “Numbers which are only divisible by 1 and the number itself”. For instance, 5 is prime because it has only 2 factors, 1 × 5 or 5 × 1, including 5 itself. However, 4 is composite because it is a product of 1 × 4 or 4 × 1 and 2 × 2. To understand this better, take a look at the table below:

Numbers	Factors	Number Of Factors
1 2 3 4 5 6 7 8 9 10 11 12	1 1, 2 1, 2 1, 2, 4 1, 5 1, 2, 3, 6 1, 7 1, 2, 4, 8 1, 3, 9 1, 2, 5, 10 1, 11 1, 2, 3, 4, 6, 12	1 2 2 3 2 4 2 4 3 4 2 6

In the above table, 2,3,5,7 and 11 are primes because they have only 2 factors – 1 and the number itself.

So from the above table, we can devise another definition of Prime Numbers – “Numbers having exactly 2 factors are known as Prime Numbers“.

Fun Exercise For You: Try to find the list of Prime Numbers between 1 to 50. You can also play it as a competition with your friends and once done you can check the answers from the table in the next section.

List of Prime Numbers from 1 to 1000

We have provided the list of primes between 1 to 1000 below:

Prime Number Between 1 To 500

Prime Numbers Between 500 To 1000

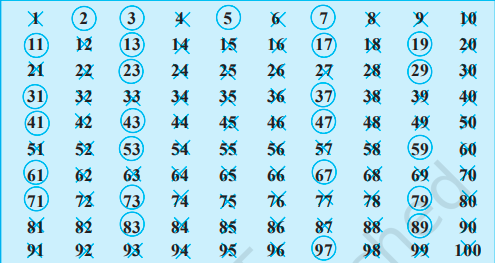
2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37, 41, 43, 47, 53, 59, 61, 67, 71, 73, 79, 83, 89, 97, 101, 103, 107, 109, 113, 127, 131, 137, 139, 149, 151, 157, 163, 167, 173, 179, 181, 191, 193, 197, 199, 211, 223, 227, 229, 233, 239, 241, 251, 257, 263, 269, 271, 277, 281, 283, 293, 307, 311, 313, 317, 331, 337, 347, 349, 353, 359, 367, 373, 379, 383, 389, 397, 401, 409, 419, 421, 431, 433, 439, 443, 449, 457, 461, 463, 467, 479, 487, 491, 499.

503, 509, 521, 523, 541, 547, 557, 563, 569, 571, 577, 587, 593, 599, 601, 607, 613, 617, 619, 631, 641, 643, 647, 653, 659, 661, 673, 677, 683, 691, 701, 709, 719, 727, 733, 739, 743, 751, 757, 761, 769, 773, 787, 797, 809, 811, 821, 823, 827, 829, 839, 853, 857, 859, 863, 877, 881, 883, 887, 907, 911, 919, 929, 937, 941, 947, 953, 967, 971, 977, 983, 991, 997.

Quick Tip: 1 is not a prime number as it has only 1 factor.

Properties of Prime Numbers

Some properties through which you can decide whether a number is prime or not are listed below:

A. From the above table, you can see that all prime numbers except 2 are odd. Therefore, 2 is the only even number that is prime.
B. Any number >1 can be divided by at least one Prime Number.
C. Coprime or relatively prime or mutually prime numbers are the ones that have 1 as HCF.
D. All the even positive integers >2 can be expressed as a sum of 2 prime numbers ( 4 = 2+2 or 8 = 5+3).

Types of Prime Numbers

Often students have difficulty in finding the types of prime numbers. So, we have tabulated the types below:

Even Prime Numbers	Odd Prime Numbers	Coprime Numbers	Twin Prime Numbers
Prime Numbers divisible by 2.	Prime Numbers not divisible by 2.	Numbers having only 1 as their common factor or HCF = 1.	Prime Numbers with a gap of 2 between them.
Eg: 2.	Eg: 3, 5, 7, 9, 11, etc.	Eg: 18 and 35.	Eg: 3 & 5 or 821 & 823.

Eratosthenes Method of Finding Prime Numbers: Prime Numbers Chart

Eratosthenes was a Greek Mathematician from the 3rd century BC who devised a method to find the Prime nos. using the below chart.

-1st Step: Cross 1 as it is not a prime no.
-2nd Step: Encircle 2 and cross all other even numbers as they are not prime.
-3rd Step: You will see that the next no. is 3 (uncrossed). Encircle 3 and cross out all its multiples.
-4th Step: Follow the 3rd Step with other odd/uncrossed numbers.
-5th Step: Continue the process till all nos. are either encircled or crossed out.

Prime Numbers Chart — Source: NCERT Book

Difference between Composite & Prime Nos.

Now that you are well aware of the steps to know Prime nos. you can easily differentiate them from composite numbers.

Composite Numbers	Prime Numbers
It has more than 2 factors.	It has only 2 factors.
These numbers are divisible by all their factors.	These are divisible by themselves and 1 only.
Eg: 6, 12, 15, 50, 124, 188, etc.	Eg: 5, 11, 61, 157, 457, etc.

Prime Numbers Questions and Solutions

So, now you have all the information on Prime Numbers, their properties, list of prime numbers from 1 to 1000, the method to find prime numbers. Let us provide you with some questions along with solutions. Since this topic belongs to CBSE NCERT Solutions For Class 6 Maths Chapter 3 Playing With Numbers, you can click the link to download the solutions for the exercise questions as well.

Example 1: Is 15 a Prime Number?
Solution 1: No, 15 is not a Prime Number because it is divisible by 1, 3, 5, and 15.

Example 2: Is 19 Prime or not?
Solution 2: Yes, since 19 has only two factors i.e. 1 and 19, it is prime.

Example 3: Write 3 pairs of Prime Numbers less than 20 whose sum is divisible by 5?
Solution 3: 3+2 = 5, 3+7 = 10, 2+13 = 15.

Example 4: Find the Prime Numbers from the following:
34, 27, 29, 41, 67, 83
Solution 4: 34 – Not prime.
27 – Divisible by 3, 9; hence, not prime.
29, 41, 67, 83 – All are prime (divisible by 1 and themselves only).

Frequently Asked Questions: FAQs

Here are some questions that students look for:

Que. What are the Prime Numbers from 1 to 1000?
Ans. We have listed all the Prime Nos. in this article – 2 being the smallest and 997 being the largest between 1 to 1000.

Que. Which is the only even Prime Number/ Which is the smallest prime number?
Ans. 2.

Que. What are the Prime Numbers between 1 to 200?
Ans. The Prime Nos between 1 to 200 are:
2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37, 41, 43, 47, 53, 59, 61, 67, 71, 73, 79, 83, 89, 97, 101, 103, 107, 109, 113, 127, 131, 137, 139, 149, 151, 157, 163, 167, 173, 179, 181, 191, 193, 197, 199.

Que. How many factors does a Prime Number have?
Ans. A Prime No. has only 2 factors.

That was all the information on Prime Numbers. We hope the information provided here helps you. However, if you have any questions, feel to use the comments section below to reach out to us and we will get back to you at the earliest.