Thermodynamic Principles of Metallurgy

Thermodynamic Principles of Metallurgy: Metallurgy is an important process of extraction of metals from their ores, and it is based upon various principles of chemistry. Several steps are followed to make the processes efficient and effective at the end of each process. Not only are the purity of the metals extracted at the end of each process important, but it is equally essential to ensure the extraction process by itself does not take more than the necessary time, effort, or any other chemical incompatibilities. Hence, the principles are very significant, and while there are many, the upmost amongst these principles is the thermodynamic principles which are applied to the metallurgical processes to make them much more efficient. The idea is to apply the thermodynamic principles of metallurgy and the basic concepts of thermodynamics, such as Gibb’s energy, in the metallurgical transformations.

Application of Thermodynamic Principles in Metallurgical Process

The concept of thermodynamics suggests that the Gibbs energy change for a particular process at a particular temperature is given by:

\({\rm{\Delta G}} = {\rm{\Delta H}} – {\rm{T\Delta S}}\)

Here, the \({\rm{\Delta H}}\) is enthalpy change, \({\rm{\Delta S}}\) is entropy change and \({\rm{\Delta G}}\) is the energy change.

The change for any reaction could be explained with the help of the reaction:

\({\rm{\Delta }}{{\rm{G}}^{\rm{0}}}{\rm{ =\, – RT}}\) In \({\rm{K}}\)

Where, \({\rm{K}}\) is the equilibrium constant of that particular reaction at temperature, \({\rm{T}}\). When the \({\rm{\Delta G}}\) is negative, it implies that the \({\rm{K}}\) is positive. This means the reaction is proceeding in the forward direction. 

Hence, accordingly, when:

1. \({\rm{\Delta G}}\) value is negative in a metallurgical process; the reaction will only proceed in the forward direction. At this time, the \({\rm{\Delta H}}\) is always positive and \({\rm{\Delta S}}\) is also positive. And, at a very high temperature, \({\rm{T\Delta S}} > {\rm{\Delta H}}\).
2. When the \({\rm{\Delta G}}\) of a reaction is positive, it can be made spontaneous by coupling it with another reaction that has a large \({\rm{\Delta G}}\) negative value. That way, the sum of two \({\rm{\Delta G}}\) of the coupled reactions becomes negative.

For example, the reduction of metal oxides to metal using a reducing agent is the subtraction of two half equations. The decomposition of \({\rm{F}}{{\rm{e}}_2}{{\rm{O}}_3}\) to \({\rm{Fe}}\) is not a spontaneous reaction and has a \(({\rm{\Delta }}{{\rm{G}}^ \circ }\) value of \( + 1487{\rm{KJ}}/{\rm{mol}}\). However, the burning of \({\rm{CO}}\) in the presence of oxygen is spontaneous \(\left( {{\rm{\Delta }}{{\rm{G}}^ \circ } = \,- 514.4\,{\rm{KJ}}/{\rm{mol}}} \right)\).

\(2{\text{F}}{{\text{e}}_2}{{\text{O}}_3}\left( {\text{s}} \right) \to 4{\text{Fe}} + 3{{\text{O}}_2}……\left( 1 \right)\)

\(2{\text{CO}}\left( {\text{g}} \right) + {{\text{O}}_2}\left( {\text{g}} \right) \to 2{\text{C}}{{\text{O}}_2}\left( {\text{g}} \right)…\left( 2 \right)\)

\(\left( {\Delta {{\rm{G}}^ \circ } =\, – 514.4\,{\rm{KJ}}/{\rm{mol}}} \right)\)

On multiplying \((2)\) by \(3\)

\(6{\rm{CO}} + 3{{\rm{O}}_2} \to 6{\rm{C}}{{\rm{O}}_2} \ldots \ldots (3)\)

\({\rm{\Delta }}{{\rm{G}}^{\rm{^\circ }}}{\rm{ = \,- 1543}}\,{\rm{.2KJ/mol}}\)

Adding equation \((3)\) with \((1)\):

\(2{\rm{F}}{{\rm{e}}_2}{{\rm{O}}_3} + 6{\rm{CO}} \to 4{\rm{Fe}} + 6{\rm{C}}{{\rm{O}}_2}\)

\({\rm{\Delta }}{{\rm{G}}^{\rm{^\circ }}}{\rm{ = \,- 56}}\,{\rm{.2KJ/mol}}\)

With this, the \({\rm{\Delta }}{{\rm{G}}^{\rm{^\circ }}}\) value has become negative, making the reaction feasible as well as spontaneous. The feasibility of the thermal reduction of ores can be predicted by drawing a plot of Gibbs energy and temperature for the reactions.

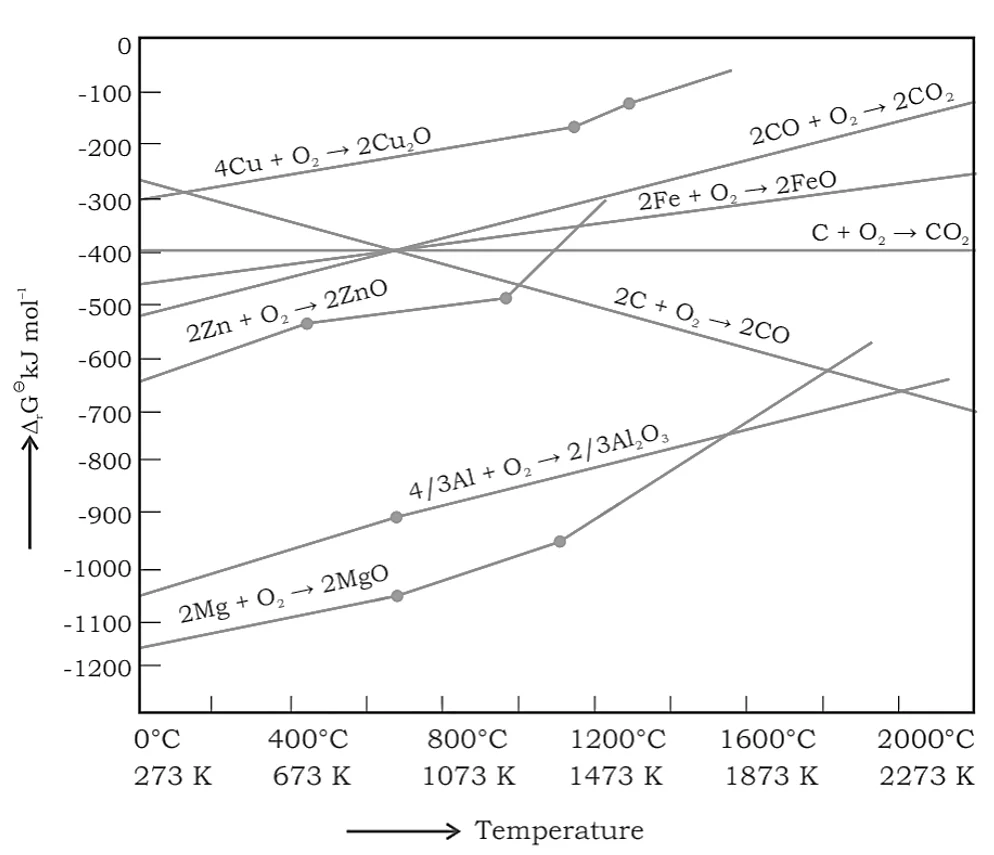
Ellingham Diagrams

Ellingham diagrams is the graphical representation of Gibb’s energy change of a reaction and the absolute temperature for the process. It can be represented as the graph below:

Below are some salient features or predictions that can be made using the Ellingham diagram:

1. The diagram generally shows a plot of \({\rm{\Delta }}{{\rm{G}}^{\rm{^\circ }}}\) versus temperature for oxide formation of elements.
   For the below reaction, the amount of gas decreases, thereby decreasing the randomness of the reaction.
   \(2{\text{xM}}\left( {\text{s}} \right) + {{\text{O}}_2}\left( {\text{g}} \right) \to 2{{\text{M}}_{\text{x}}}{\text{O}}\left( {\text{s}} \right)\)
   The entropy changes for the reaction, therefore, is negative. The term \({\rm{T}}\Delta {\rm{S}}\) becomes positive in the equation:
   \(\Delta {\rm{G}} = \Delta {\rm{H}} – {\rm{T}}\Delta {\rm{S}}\)
   Therefore, the energy change increases with increases in temperature for the formation of oxides of type: \({{\rm{M}}_{\rm{x}}}{\rm{O}}\), and the slope is also positive.

2. Every plot on the diagram is a straight line, except for those instances when phase changes occur. The slope of the peak increases to the positive side at the temperature at which the change occurs. For example, the \({\rm{Zn}}\) and \({\rm{ZnO}}\) plot shows an abrupt change in the curve at the melting point.
   
3. The Ellingham diagram gives the plots of \({\rm{\Delta }}{{\rm{G}}^{\rm{^\circ }}}\) for the oxidation and the reduction of corresponding species of common metals as well as some reducing agents too.

Applications of Ellingham Diagram

1. The diagram predicts the feasibility of thermal reduction of the ore.
2. It helps in the selection of a reducing agent for the reduction of a metal oxide.
3. By looking at the diagram, one can predict how the reduction of oxides of elements in the upper line is possible with the elements represented in the lower line.

Limitations of Ellingham Diagram

1. The reactants and the products need to be in equilibrium for the interpretation of energy from the diagram.
2. The kinetics of the reduction process is not indicated by the diagram. It only indicates the thermodynamic feasibility of the reduction process.

Applications of Thermodynamics in Metallurgy

Thermodynamic principles can be applied in various processes of metallurgy for the extraction of metals from their ores. For example, thermodynamic principles are used in the below processes:

1. Extraction of Iron from oxide ore
2. Extraction of copper from Copper (I) oxide and
3. Extraction of Zinc from Zinc Oxide.

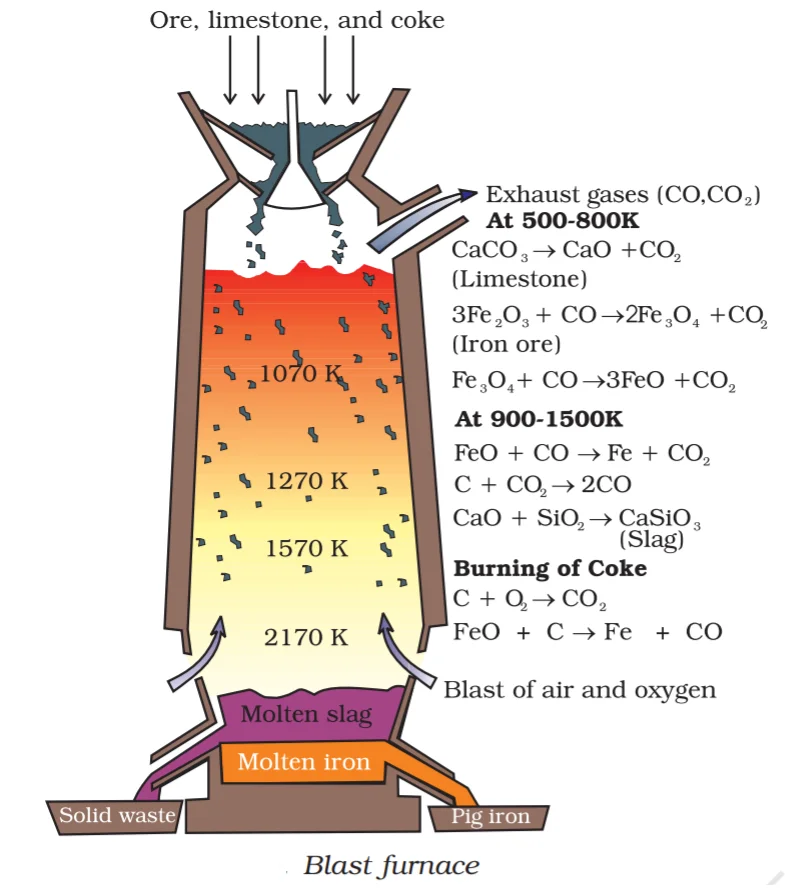
1. Extraction of Iron from Iron Oxide
Iron is extracted from the oxide ore by the step-by-step process in the blast furnace. The roasted or calcinated ore is mixed with limestone and coke and then fed into the blast furnace from the top. The oxide ore melts to give metal inside the furnace. The reaction involved are as follows:
\({\rm{FeO(s) + C(s)}} \to {\rm{Fe(solid}}\,{\rm{or}}\,{\rm{liquid) + CO(g)}}\)

The reaction is a combination of two of the following reactions:
\({\text{FeO}}\left( {\text{s}} \right) \to {\text{Fe}}\left( {\text{s}} \right) + \frac{1}{2}{{\text{O}}_2}\left[ {\Delta {{\text{G}}_{\left( {{\text{FeO,}}\,{\text{Fe}}} \right)}}} \right]\)
\({\text{C}}\left( {\text{s}} \right) + \frac{1}{2}{{\text{O}}_2}\left( {\text{g}} \right) \to {\text{CO}}\left( {\text{g}} \right);\left[ {\Delta {{\text{G}}_{\left( {{\text{C,}}\,{\text{CO}}} \right)}}} \right]\)

The net Gibb’s energy for the reaction becomes:
\(\Delta {{\text{G}}_{\left( {{\text{C,}}\,{\text{CO}}} \right)}} + \Delta {{\text{G}}_{\left( {{\text{FeO,}}\,{\text{Fe}}} \right)}} = {\Delta _{\text{r}}}{\text{G}}\)

In the Ellingham diagram, the like for \(\Delta {\text{G}}_{\left( {{\text{FeO,}}\,{\text{Fe}}} \right)}^{\text{o}}\) goes above the line for \({\Delta {{\text{G}}_{\left( {{\text{C,}}\,{\text{CO}}} \right)}}}\). Since the line for \({\Delta {{\text{G}}_{\left( {{\text{C,}}\,{\text{CO}}} \right)}}}\) at \({\rm{1073}}\,{\rm{K}}\) is much lower, coke will reduce \({\rm{FeO}}\) to Fe at this temperature and gets oxidized to \({\rm{CO}}\). The following steps are involved in the extraction of Iron from Iron Oxide:

Step 1:  Concentration of the ore by hydraulic washing method.
Step 2: Calcination of the concentrated ore by heating it in the absence of air to remove moisture and volatile impurities.
Step 3: Oxidation of the concentrated, calcinated ore by heating it in excess of air to convert ferrous oxide to ferric oxide by the following reaction:
\(4{\rm{FeO}} + {{\rm{O}}_2} \to 2{\rm{F}}{{\rm{e}}_2}{{\rm{O}}_3}\)
Step 4: Smelting process wherein the ore undergoes reduction with carbon in the blast furnace.
Ore, at this stage, is mixed with limestone and coke in a ratio of \(8:1:4\) (called as charge) and placed in the furnace. A blast of hot air is blown from the bottom, through the tuyeres or narrow pipes.
Step 5: The reduction of iron oxides takes place at different temperatures in the blast furnace. The zones in the blast furnace are divided into reduction zone, combustion zone, and melting zone, and each zone has a particular reaction going on to complete the process.

2. Extraction of Copper

Although copper occurs mostly as sulphides, the Ellingham diagram suggests that reduction of oxides of copper would be easier. Hence, instead of \({\rm{C}}{{\rm{u}}_2}\;{\rm{S}},{\rm{C}}{{\rm{u}}_2}{\rm{O}}\) reduction is made. For this, the sulphide is first converted into oxide, and the reduction of oxide is carried out to obtain copper. The following steps are involved in the extraction of copper from its ore:

Step 1: Concentration of copper pyrite ore is done by powdering it and using the froth flotation process.
Step 2: Roasting the concentrated ore by strongly heating it in excess of air. As, \({\rm{S}}\) and \({\rm{Sb}}\) are removed in the process as oxides, and copper pyrite gets converted to copper sulphide.
Step 3: Smelting process wherein the roasted ore is mixed with silica and coke and heated in the reverberatory furnace in the presence of hot air.
Step 4:  Bessemerisation, in which the copper sulphide is oxidized after all the Iron sulphide is oxidised. Molten copper is collected, which is called blister copper. This way, most extraction processes of metals involve the use of the Ellingham diagram to predict the feasibility and determine the best approach for performing the extraction reactions.

Summary

Thermodynamic principles such as the use of energy change are widely employed in metal extraction or metallurgical processes to make it feasible and spontaneous. For a reaction to be feasible, the energy change needs to be negative. When some reactions have positive energy change and are not spontaneous, they are made so by coupling it with other reactions which have large negative energy change. Ellingham diagram predicts such feasibilities by plotting Gibbs energy change and the absolute temperature. The plots determine the feasibility of the thermal reduction of ore. The oxides of elements on the upper line can be reduced by the elements on the lower line in the Ellingham diagram. The Ellingham diagram and its principles are used for the extraction of several metals such as Iron, Copper and Zinc.

FAQs

Q.1. What is the use of thermodynamics in metallurgy?
Ans: Thermodynamic principles and values such as energy and entropy change are used to predict the feasibility and spontaneity of a reaction, such as oxidation for extraction of metals from their ores.

Q.2. What are the applications of thermodynamics in metallurgy?
Ans: Thermodynamics is used in the extraction of metals from their ores. For example, thermodynamic principles are used for the extraction of iron, zinc and copper from their oxides.

Q.3. What are Ellingham Diagrams?
Ans: Ellingham diagram is a graphical representation of Gibb’s energy change and the absolute temperature for any process or reaction.

Q.4. What are the salient features of Ellingham Diagrams?
Ans: Here are some of the salient features of the Ellingham diagram:
● Ellingham diagram plots the energy change of the reaction with temperature for the formation of oxides of elements.
● Each plot in an Ellingham diagram is a straight line, except for when phase change happens, where it curves or the slope increases to the positive side.
● There is a point in the curve below which \({\rm{\Delta G}}\) is negative, and the oxide is stable, while above this point, the oxide decomposes on its own.

Q.5. What are the stages of Iron extraction?
Ans: The extraction of Iron from Iron oxide ore takes place in the blast furnace. The stages of Iron extraction are as given below:
● Concentration of the ore.
● Calcination of the concentrated ore in the absence of air.
● Oxidation of the concentrated ore in the presence of air.
● Smelting or the ore.
● Reduction of Iron Oxide to Iron.

Learn About State Functions Here

We hope this detailed article on the Thermodynamic Principle of Metallurgy is helpful. If you have any queries, please ping us through the comment box below and we will get back to you as soon as possible.