Variations: Definition, Example, Types

Variations: The term ‘variation’ refers to a mathematical concept dealing with variability. Any change in one quantity due to a change in another is referred to as variation. In mathematics, we frequently encounter various types of variation problems. Variation problems involve relatively simple relationships or formulas in which one variable equals one term. The most common types of variation are direct and inverse variations.

Variations assist some organisms in their quest for survival. It assists in adapting to new circumstances. The organisms develop new traits as a result of discontinuous variations. Variation is necessary for growth and occurs during sexual reproduction. Variations give each creature its personality.

LEARN EXAM CONCEPTS ON EMBIBE

What is a Variation?

In problems involving two or more variables, the value of one variable changes as the value (s) of the related variable changes (or variables). The variation is a relationship between a set of values of one variable and the values of other variables.

Example:

Variation is illustrated by the simple equation \(y = kx\), where \(k\) is a constant. When \(k = 5\), the equation becomes \(y = 5x\).
When \(x = 1,\,y = 1 \times 5 = 5\)
When \(x = 2,\,y = 2 \times 5 = 10\)
When \(x = 3,\,y = 3 \times 5 = 15\)
Simply put, the value of \(y\) varies with the value of \(x\).
We can see the variation of \(y\) with various values of \(x\). Similarly, the value of \(x\) changes with different values of \(y\).

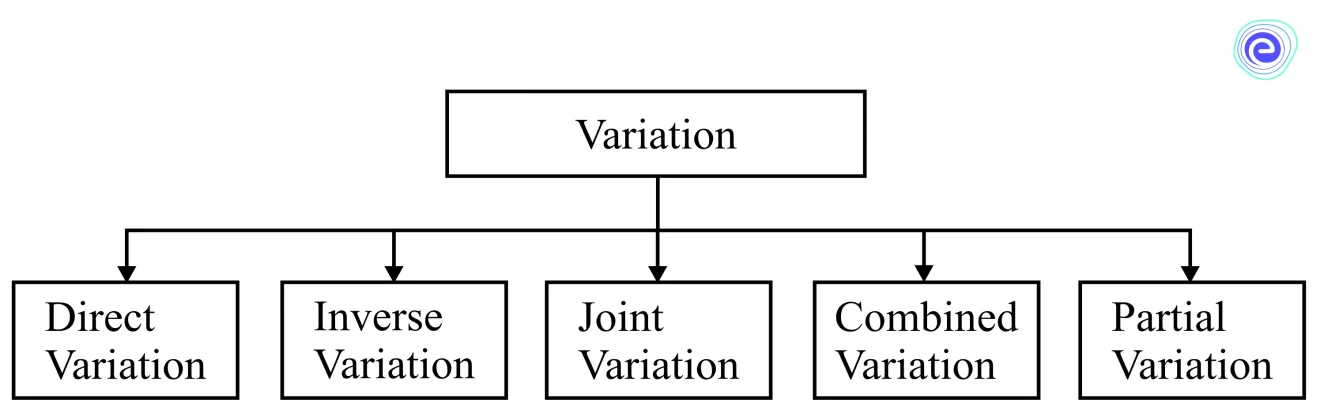
Types of Variations

Variation can be classified into several types based on the pattern of change or the relationships between variables.

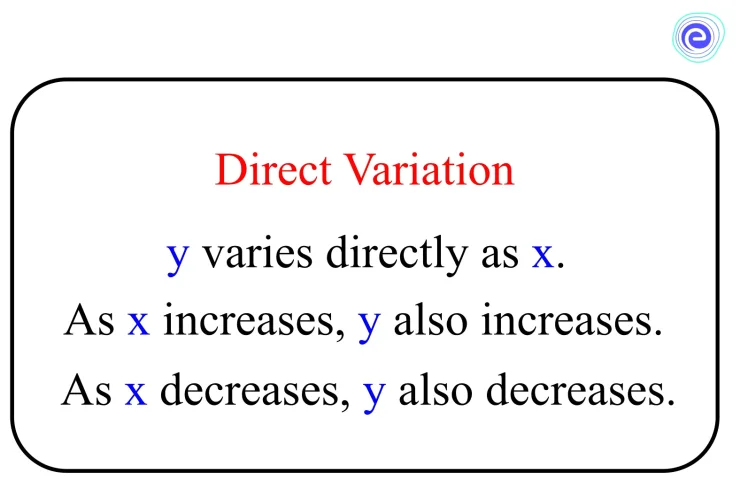
Direct Variation

The relationship between two variables in which one is a multiple of the other is direct variation.

For instance, if \(b\) is directly proportional to \(a\), the equation is \(b = ka\) (where \(k\) is a constant). When the ratio of two variables remains the same, they are in direct variation.

Direct variation can be expressed as \({\text{A}} \propto {\text{B}}\). It is read as ‘\({\text{A}}\) varies as \({\text{B}}\)’ or ‘A is proportional to B’.

Examples:

1. If we have to buy more pens, we need more money
2. The cost of vegetables directly varies with their weight.

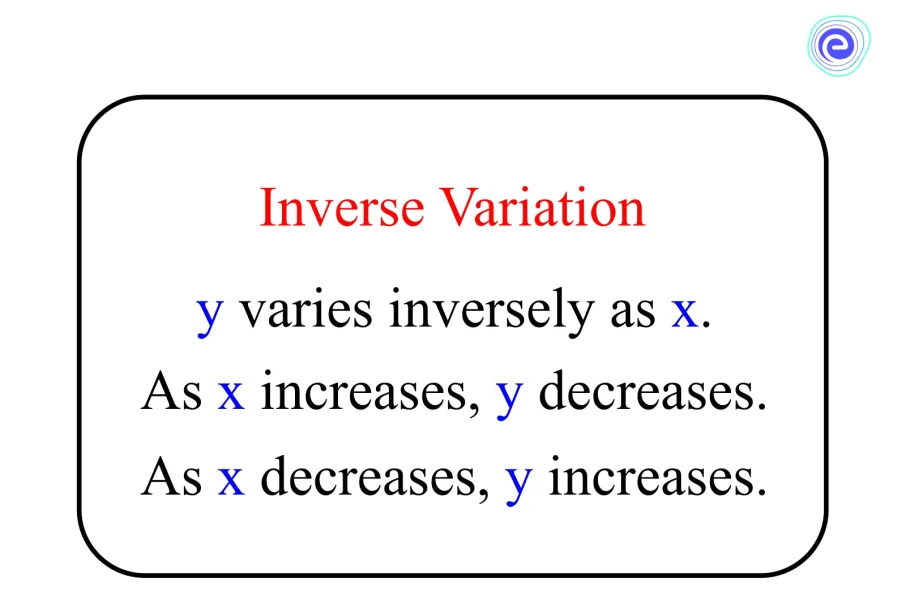
Indirect Variation

Indirect variation occurs when two variables change in opposite directions. In the indirect variation, one variable is equal to the inverse of the other variable, which is multiplied by a constant. When one variable increases, the other decreases.
The general equation for an inverse variation is

\(y = k \cdot \frac{1}{x} \Rightarrow xy = k\)

Hence, we can say that for inverse variation, the product of two variables is a constant.

Thus, if \({\text{A}}\) varies inversely as \({\text{B}}\), we write \({\text{A}} \propto \frac{1}{{\text{B}}}\) or, \({\text{A}} = {\text{m}}\frac{1}{{\text{B}}} \Rightarrow {\text{AB}} = m\), where \(m\) is the variation constant.

As a result, if the product of the corresponding values of two variables is constant, then one quantity varies in the opposite direction as another.

Examples:

1. More men will take less time to complete the work
2. The bank balance is inversely varies with the expenditure

Joint Variation

Joint variation occurs when more than two variables are directly related or when one variable changes due to the change in the product of two or more variables.
If \(X\) has a joint variation with \(Y\) and \(Z\), it can be written symbolically as
\(X \propto YZ\)
If \(Y\) is also constant, then \(X\) is directly proportional to \(Z\). In simpler words, joint variation is similar to direct variation with more than two variables.
The joint variation equation is \(X = kYZ\), where \(k\) is constant.

When one variable varies directly with the product of several other variable quantities, it is said to be joint variation.
If \(\rm{A}\) varies directly as the product of \(\rm{B, C}\) and \(\rm{D}\), that is, if \({\rm{A}} \propto {\rm{BCD}}\) or \({\rm{A}} = k{\rm{BCD}}\), then \({\rm{A}}\) varies jointly as \({\rm{B, C}}\) and \({\rm{D}}\).

Examples:

1. Area of the rectangle varies with changes in its length and breadth.
2. Cost per person while travelling is increasing with the number of persons and distance travelled.

Combined Variation

The term refers to a situation where a variable is dependent on two (or more) variables and varies directly with some of them while varying inversely with the rest (while the other variables are constant).

If \(z\) varies directly as \(x\;\left( {z \propto x} \right)\) and inversely as \(y\;\left( {z \propto \frac{1}{y}} \right),\) the combined variation equation is represented as
\(z \propto \frac{x}{y}\)
\(\Rightarrow z = k\frac{x}{y}\)
Where, \(k\) is a constant

Examples:

1. Speed of a car varies directly with the distance and inversely with the time taken.
2. Bank balance directly varies with the savings and inversely with the expenditure.

PRACTICE EXAM QUESTIONS AT EMBIBE

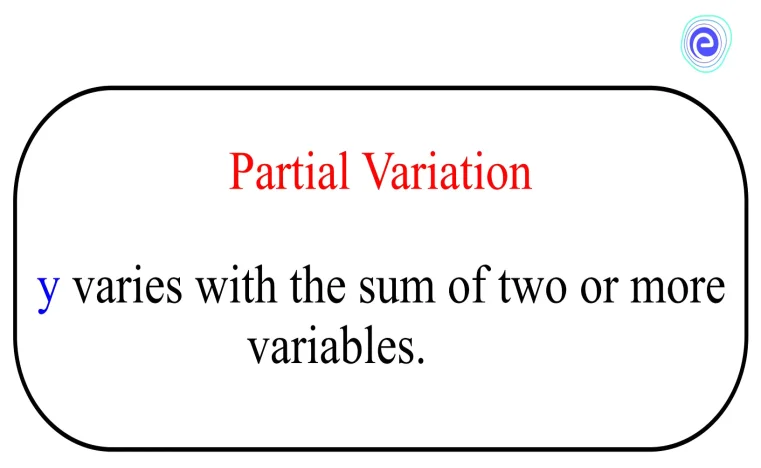
Partial Variation

A partial variation is when a formula relates two variables or when a variable varies with the sum of two or more variables.

Examples:

1. Equation of a straight line, \(y = mx + C\)
2. Cost of making a dress depends on: the cost of material (a constant) and time taken to stitch a dress (partly variable).

Solved Examples – Variations

Below are a few solved examples that can help in getting a better idea.

Q.1. If \(A\) varies directly as \(B\), the value of \(A\) is \(60\), and \(B\) is \(40\). Find the value of the proportionality constant by writing the equation that describes the direct variation of \(A\) and \(B\).
Ans:
Given: \({\text{A}} \propto {\text{B}}\)
Then, the ratio of \({\text{A}}\) and \({\text{B}}\) is a constant for any value of\({\text{A}}\) and \({\text{B}}\).
\( \Rightarrow k = \frac{{\text{A}}}{{\text{B}}} = \frac{{60}}{{40}} = \frac{3}{2}\)
\(\therefore \,{\text{A}} = \frac{3}{2}{\text{B}}\)
Hence, the value of the proportionality constant, \(k = \frac{3}{2}\) or \(1.5\).

Q.2. If a car runs at a speed of \(40\,kmph\) and it takes \(3\) hours to run a given distance. What time will it take to run the same distance with a speed of \(60\,kmph\)?
Ans:
Let \({\text{S}}\) be the distance, \({\text{V}}\) be the speed, and \({\text{T}}\) be the time taken.
As distance is constant, we know that speed and time are inversely proportional.
So, \({\text{V}} \propto \frac{1}{{\text{T}}}\) or \({\text{V}} = \frac{{\text{S}}}{{\text{T}}}\)
Distance, \({\text{S}} = {\text{VT}}\)
\(= 40 \times 3\)
\(\therefore {\rm{S}} = 120\,{\rm{km}}\).
When \(V = 60\,{\rm{kmph}}\),
\(120 = 60 \times {\text{T}}\)
\( \Rightarrow {\text{T}} = \frac{{12}}{{60}}\)
\(\therefore {\rm{T}} = 2\) hours.

Q.3. If \(x\) is in inverse variation with the square of \(y\), and when \(x = 4\), \(y = 3\). Find the value of \(x\), when \(y = 6\).
Ans:
Given: \(x \propto \frac{1}{{{y^2}}}\)
\(\therefore \,x = \frac{k}{{{y^2}}}\)
Given: when \(x = 4\), \(y = 3\)
\( \Rightarrow 4 = {\frac{k}{{{3^2}}}}\)
\(\Rightarrow k = 4 \times {3^2}\)
\(= 4 \times 9\)
\(\therefore \,k = 36\)
When \(y = 6\),
\(x = \frac{{36}}{{{6^2}}}\)
\(= \frac{{36}}{{{36}}}\)
\(\therefore \,x = 1\).

Q.4. The variable \(x\) is in joint variation with the variables \(y\) and \(z\). The value of \(x\) is \(16\), when the values of \(y\) and \(z\) are \(4\) and \(6\). Find the value of \(x\) when \(y = 8\) and \(z = 12\)?
Ans:
Given: \(x\) is in joint variation with the variables \(y\) and \(z\).
\(\Rightarrow x \propto yz\) or \(x = kyz\)
Given: \(x\) is \(16\), when the values of \(y\) and \(z\) are \(4\) and \(6\).
\(16 = k \times 4 \times 6\)
\( \Rightarrow k = \frac{{16}}{{24}}\)
\(\therefore k = \frac{2}{3}\)
Now for the values of \(y = 8\) and \(z = 12\),
\(x = \frac{2}{3} \times 8 \times 12\)
\(\therefore \,x = 64\).

Q.5. A rectangle has a length of \(6\) meters and a width of \(4\) meters. How much would the perimeter increase or decrease if the length is doubled and the breadth is halved?
Ans:
Perimeter of a rectangle, \(P = 2(l + b)\)
Here, \(2\) is a constant.
If length is doubled, then the new length \(= 2l\).
Breadth is halved, then the new breadth \(= \frac {w}{2}\).
So, the new perimeter, \(P = 2\left( {2l + \frac{w}{2}} \right)\)
\( = 2\left( {2\;x\;6 + \frac{4}{2}} \right)\)
\(\therefore P = 28\,{\rm{m}}\)
Hence, the perimeter will increase by \((28 – 20) = 8\,{\rm{m}}\).

Summary

Variation tells that a change in one variable will cause a change in one or two or more variables. There are several types of variations, such as direct variation, indirect or inverse variation, joint variation, partial variation and combined variation. The concept of variations is used in many fields such as Mathematics, Science, Medicine and Engineering.

Variations help determine real-life situations like men and their work, speed and time of travelling etc. In a direct variation, variables are directly proportional, while in an indirect variation, they are inversely proportional. A joint variation is when a variable is directly proportional to two or more variables. Whereas a combined variation, some variables are in direct variation while others are indirectly proportional.

FAQs on Variations

Students might be having many questions with respect to the Variations. Here are a few commonly asked questions and answers.

Q.1. What are variations in Math?
Ans: Variation is a term used to describe a relationship between the change in the value of a variable and the changes in the values of related variables. An example of a basic equation \(y = mx\), where \(m\) is a constant, can be used to show this.

Q.2. What are the four types of variations?
Ans: The four types of variations are

• Direct variation
• Inverse variation
• Joint variation
• Combined variation

Q.3. What is the difference between joint and combined variations?
Ans: In joint variation, one variable is directly proportional to two or more variables. Whereas, in combined variation, a variable varies directly with some variables and inversely with others.

Q.4. Give an example of direct variation.
Ans: As the radius increases, the circumference also increases. Hence, the circumference and radius of a circle are in direct variation.

Q.5. What is a partial variation?
Ans: Partial variation is defined when a variable is related to the sum of two or more variables. The equation of straight line, \(y = mx + C\), where \(m\) and \(C\) are constants, shows the partial variation.

ATTEMPT MOCK TESTS ON EMBIBE

We hope this information about the Variations has been helpful. If you have any doubts, comment in the section below, and we will get back to you.