Chittaranjan Dasgupta and Asok Kumar Das Solutions for Chapter: Motion of System of Particles and Rigid Body, Exercise 1: EXERCISE

Two particles $A$ and $B$, of mass $M$ each are attached at the ends of a rigid massless rod of length $L$. A particle $\mathrm{P}$ of the same mass moving with a speed of $V$ at right angle to$\mathrm{AB}$  strikes $A$ and sticks to it. Then

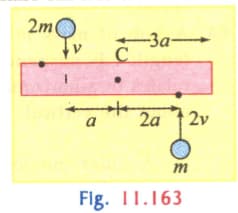
A uniform bar of length $6$ and mass $8\mathrm{m}$ lies on a smooth horizontal table. Two point masses $\mathrm{m}$ and $2 \mathrm{m}$ moving in the same horizontal with speed $2\mathrm{v}$ and $\mathrm{v}$ respectively, strike the bar as shown in the figure, and stick to the bar after the collision. Denoting angular velocity (about the centre of mass), total energy and centre of mass velocity by $\omega , E$ and $v_{\mathit{c}}$ respectively, we have after the collision:

A sphere is rolling without slipping on a fixed horizontal plane surface. In the figure, $A$ is the point of contact, $B$ is the centre of the sphere and $C$ is the topmost point. Then

The density of a rod increases linearly with distance from the end $A$ to the other end $B$. Its mid-point is at $C$ and centre of mass is at $G$. Moment of inertia of the rod about the axes passing through $A\mathit{,}\mathit{ }B\mathit{,}\mathit{ }C$ and $G$ and perpendicular to the length of the rod are $I_{\mathit{A}}\mathit{,}\mathit{ }{I}_{\mathit{B}}\mathit{,}\mathit{ }{I}_{\mathit{C}}$ and $I_G$  respectively. Then

A block with length, breadth and height respectively as $a$, $a$ and $h$ is placed on a rough inclined plane. The inclination of plane is gradually increased. If $\mu$ is the coefficient of friction, the block will

In a figure, ladder of mass $\mathrm{m}$ is shown leaning against a wall. It is in static equilibrium making an angle $\theta$ with the horizontal floor. The coefficient of friction between the wall and the ladder is ${\mu }_1$ and that between the floor and the ladder is ${\mu }_2$. The normal reaction of the wall on the ladder is $N_1$ and that of the floor is $N_2$. If the ladder is about to slip, then:

A circular disc rolls on a horizontal floor without slipping and the centre of disc moves with a uniform velocity $v$. Which of the following values the velocity at a point on the ring of the disc can have?

A large number of particles are placed around the origin, each at a distance $R$ from the origin. The distance of the centre of mass from the origin is: