Embibe Experts Solutions for Chapter: Rotational Mechanics, Exercise 1: Exercise - 1

The moment of inertia of an elliptical disc of uniform mass distribution of mass $m,$ semi major axis $r,$ semi minor axis $d$ about its axis is:

In case of torque of a couple if the axis is changed by displacing it parallel to itself, torque will :

A particle moves with a constant velocity parallel to the $y$-axis. Its angular momentum about the origin

A sphere is released on a smooth inclined plane from the top. When it moves down its angular momentum is:

A circular wooden loop of mass $m$ and radius $R$ rests flat on a horizontal frictionless surface. A bullet, also of mass $m$, and moving with a velocity $v$, strike the loop and gets embedded in it. The thickness of the loop is much smaller than $R$. The angular velocity with which the system rotates just after the bullet strikes the loop is

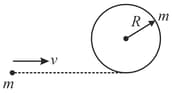
A uniform cube of side $a$ and mass $m$ rests on a rough horizontal table. A horizontal force $F$ is applied normally to one of the faces at a point that is directly above the centre of the face, at a height $\frac{3a}{4}$ above the base. The minimum value of $F$ for which the cube begins to tilt about the edge is (Assume that the cube does not slide).

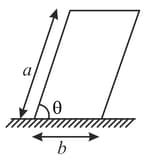
A homogenous block having its cross-section to be a parallelogram of sides $a$ and $b$ (as shown) is lying at rest and is in equilibrium on a smooth horizontal surface. Then for acute angle $\mathrm{\theta }$:

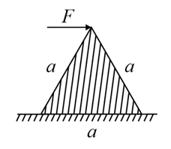
An equilateral uniform prism of mass $m$ rests on a rough horizontal surface with a coefficient of friction $\mu$. A horizontal force $F$ is applied to the prism as shown in the figure. If the coefficient of friction is sufficiently high so that the prism does not slide before toppling, then the minimum force required to topple the prism is: