Andhra Pradesh Board Solutions for Chapter: Quadrilaterals, Exercise 5: EXERCISE - 8.3
Andhra Pradesh Board Mathematics Solutions for Exercise - Andhra Pradesh Board Solutions for Chapter: Quadrilaterals, Exercise 5: EXERCISE - 8.3
Attempt the practice questions on Chapter 8: Quadrilaterals, Exercise 5: EXERCISE - 8.3 with hints and solutions to strengthen your understanding. Mathematics Class 9 solutions are prepared by Experienced Embibe Experts.
Questions from Andhra Pradesh Board Solutions for Chapter: Quadrilaterals, Exercise 5: EXERCISE - 8.3 with Hints & Solutions
is a parallelogram and are perpendiculars drawn from vertices and on diagonal , show that
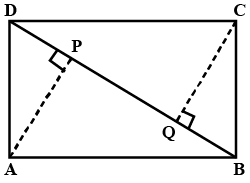
is a parallelogram and are perpendiculars drawn from vertices and on diagonal , show that
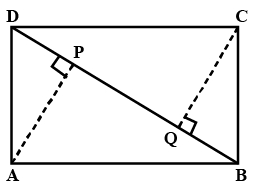
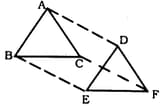
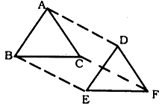
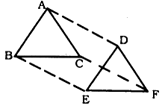
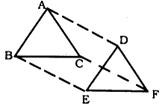
In and , and ; and . Vertices and are joined to vertices and respectively. Show that is a parallelogram.
In and , and ; and . Vertices and are joined to vertices and respectively. Show that is a parallelogram.
In and , and ; and . Vertices and are joined to vertices and respectively. Show that .
In and , and ; and . Vertices and are joined to vertices and respectively. Show that .
is a parallelogram. and are the diagonals intersect at . and are the points of trisection of the diagonal . Prove that and also bisects
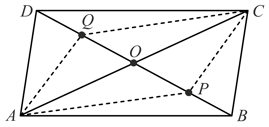
is a square. and are the midpoints of and respectively. Such that . Prove that is a square.