D. C. Pandey Solutions for Chapter: Geometric Optics, Exercise 15: Objective Problems [Level 2]

A biconvex lens of focal length $15 \mathrm{cm}$ is in front of a plane mirror. The distance between the lens and the mirror is $10 \mathrm{cm}$. A small object is kept at a distance of $30 \mathrm{cm}$ from the lens. The final image is,

The focal length of a thin biconvex lens is $20 \mathrm{cm}$. When an object is moved from a distance of $25 \mathrm{cm}$ in front of it to $50 \mathrm{cm}$, the magnification of its image changes from $m_{25}$ to $m_{50}$. The ratio $\frac{m_{25}}{m_{50}}$ is,

A square wire of side $1 \mathrm{cm}$ is placed perpendicular to the principle axis of a concave mirror of focal length $15 \mathrm{cm}$ at a distance of $20 \mathrm{cm}$. The area enclosed by the image of the wire is,

A ray of light is incident on a plane mirror at an angle of $60°$. The angle of deviation produced by the mirror is,

A point object $O$ is placed in front of a glass rod having spherical end of radius of curvature $30 \mathrm{cm}$. The image would be formed,

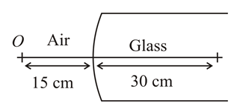
$A\text{,} B$ and $C$ are the parallel sided transparent media of refractive indices $n_1\text{,} n_2$ and $n_3$, respectively. They are arranged as shown in the figure. A ray is incident at an angle $i$ on the surface of separation of $A$ and $B$ as shown in the figure. After refraction in the medium $B$, the ray grazes the surface of separation of the media $B$ and $C$. Then, $\sin i$ is equal to,

A boat has green light of wavelength, $\lambda =500\mathrm{nm}$ on the mast. What wavelength would be measured and what colour would be observed for this light as seen by a diver submerged in water by the side of the boat? Given, $n_{\mathrm{w}}=\frac{4}{3}$.

Two beams of red and violet colours are made to pass separately through a prism of $A=60°$. In the minimum deviation position, the angle of refraction inside the prism will be,