David Sang and Graham Jones Solutions for Chapter: Uniform Electric Fields, Exercise 4: Questions

In Figure, two parallel plates are shown, separated by $25 \mathrm{~cm}$.

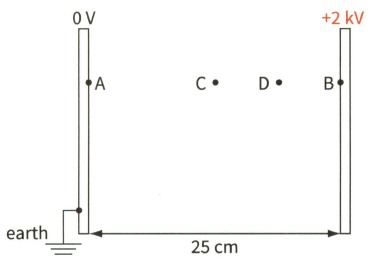
Copy the diagram and draw field lines to represent the field between the plates.

In Figure, two parallel plates are shown, separated by $25 \mathrm{cm}$.  What is the potential difference between points A and B?

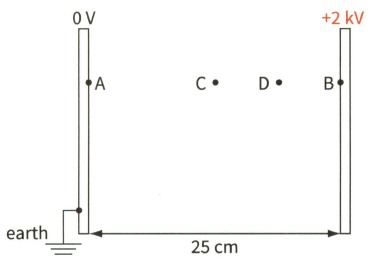
In Figure, two parallel plates are shown, separated by $25 \mathrm{~cm}$.

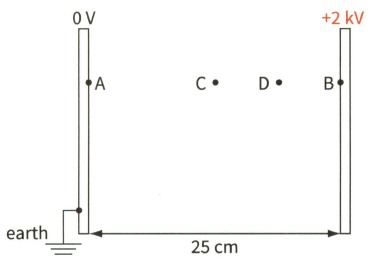
What is the electric field strength at $C$, and at $\mathrm{D}$ ?

In Figure, two parallel plates are shown, separated by $25 \mathrm{~cm}$.

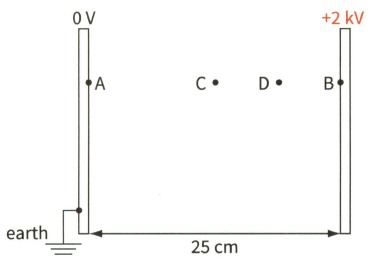
Calculate the electric force on a charge of $+5 \mu \mathrm{C}$ placed at $\mathrm{C}$. In which direction does the force act?

A particle of charge $+2 \mu \mathrm{C}$ is placed between two parallel plates, $10 \mathrm{~cm}$ apart and with a potential difference of $5 \mathrm{kV}$ between them. Calculate the field strength between the plates, and the force exerted on the charge.

We are used to experiencing accelerations that are usually less than $10 \mathrm{~m} \mathrm{~s}^{-2}$. For example, when we fall, our acceleration is about $9.81 \mathrm{~m} \mathrm{~s}^{-2}$. When a car turns a corner sharply at speed, its acceleration is unlikely to be more than $5 \mathrm{~m} \mathrm{~s}^{-2} .$ However, if you were an electron, you would be used to experiencing much greater accelerations than this. Calculate the acceleration of an electron in a television tube where the electric field strength is $50000 \mathrm{~V} \mathrm{~cm}^{-1}$. (Electron charge $-e=-1.6 \times 10^{-19} \mathrm{C} ;$ electron mass $m_{\mathrm{e}}=9.11 \times 10^{-31} \mathrm{~kg} .$ )

Use a diagram to explain how the electric force on a charged particle could be used to separate a beam of electrons $\left(\mathrm{e}^{-}\right)$ and positrons $\left(\mathrm{e}^{+}\right)$ into two separate beams. (A positron is a positively charged particle that has the same mass as an electron but opposite charge. Positron-electron pairs are often produced in collisions in a particle accelerator.)

Use a diagram to explain how the electric force on a charged particle could be used to separate a beam of electrons $\left(\mathrm{e}^{-}\right)$ and positrons $\left(\mathrm{e}^{+}\right)$ into two separate beams. (A positron is a positively charged particle that has the same mass as an electron but opposite charge. Positron-electron pairs are often produced in collisions in a particle accelerator.)