Embibe Experts Solutions for Chapter: Oscillations, Exercise 1: JEE Advanced Paper 2 - 2019

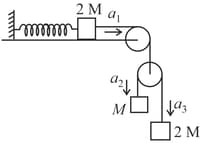
A block of mass $2\mathrm{M}$ is attached to a massless spring with spring-constant $\mathrm{k}.$ This block is connected to two other blocks of masses $\mathrm{M}$ and $2\mathrm{M}$ using two massless pulleys and strings. The accelerations of the blocks are ${\mathrm{a}}_1,{\mathrm{a}}_2$ and ${\mathrm{a}}_3$ as shown in figure. The system is released from rest with the spring in its unstretched state. The maximum extension of the spring is ${\mathrm{x}}_0.$ Which of the following option(s) is/are correct?
[ $\mathrm{g}$ is the acceleration due to gravity. Neglect friction]

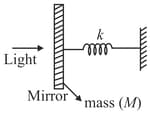
A perfectly reflecting mirror of mass $\mathrm{M}$ mounted on a spring constitutes a spring-mass system of angular frequency $\mathrm{\Omega }$ such that $\frac{4\mathrm{\pi }\mathrm{M}\mathrm{\Omega }}{\mathrm{h}}={10}^{24}{\mathrm{m}}^{-2}$ with $\mathrm{h}$ as Planck's constant. $\mathrm{N}$ photons of wavelength $\mathrm{\lambda }=8\mathrm{\pi }\times {10}^{-6}\mathrm{m}$ strike the mirror simultaneously at normal incidence such that the mirror gets displaced by $1\mathrm{\mu }\mathrm{m}.$ If the value of $\mathrm{N}$ is $\mathrm{x}\times {10}^{12},$ then the value of $\mathrm{x}$ is________.
[Consider the spring as massless]

A block with mass $M$ is connected by a massless spring with stiffness constant $k$ to a rigid wall and moves without friction on a horizontal surface. The block oscillates with small amplitude $A$ about an equilibrium position $x_0$. Consider two cases : (i) when the block is at $x_0$ and (ii) when the block is at $x=x_0+A$. In both the cases, a particle with mass $m\left(<M\right)$ is softly placed on the block after which they stick to each other. Which of the following statement(s) is(are) true about the motion after the mass $m$ is placed on the mass $M$?

A particle of mass $m$ is attached to one end of a massless spring of force constant $k$, lying on a frictionless horizontal plane. The other end of the spring is fixed. The particle starts moving horizontally from its equilibrium position at time $t=0$ with an initial velocity $u_0$. When the speed of the particle is $0.5u_0$, it collides elastically with a rigid wall. After this collision :

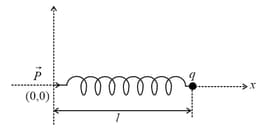
One end of a spring of negligible unstretched length and spring constant $k$ is fixed at the origin $(0, 0)$. A point particle of mass $m$ carrying a positive charge $q$ is attached at its other end. The entire system is kept on a smooth horizontal surface. When a point dipole $\overrightarrow{p}$ pointing towards the charge $q$ is fixed at the origin, the spring gets stretched to a length $l$ and attains a new equilibrium position (see figure below). If the point mass is now displaced slightly by $\mathrm{\Delta }l\ll l$ from its equilibrium position and released, it is found to oscillate at frequency $\frac{1}{\delta }\sqrt{\frac{k}{m}},$ The value of $\delta$ is _______.

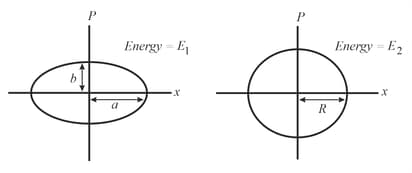
Two independent harmonic oscillators of equal mass are oscillating about the origin with angular frequencies ${\mathrm{\omega }}_1$ and ${\mathrm{\omega }}_2$ and have total energies $E_1$ and $E_2$ , respectively. The variations of their momenta $p$ with positions $x$ are shown in the figures. If $\frac{a}{b}=n^2$ and $\frac{a}{R}=n$ , then the correct equation(s) is (are)