Embibe Experts Solutions for Chapter: Ray Optics, Exercise 1: KCET (UG) 2018

An object is placed at the focus of a convex mirror. The image will be at

An object is placed at a distance of $20 \mathrm{cm}$ from the pole of a concave mirror of focal length $10 \mathrm{cm}$. The distance of the image formed is

A candle placed $25 \mathrm{cm}$ from a lens forms an image on the screen placed $75 \mathrm{cm}$ on the other side of the lens. The focal length and type of the lens should be

In refraction, light waves are bent on passing from one medium to second medium because, in the second medium.

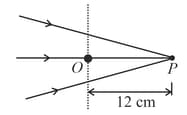
The following figure shows a beam of light converging at point $P$. When a concave lens of focal length $16 \mathrm{cm}$ is introduced in the path of the beam at a place shown by dotted line such that $OP$ becomes the axis of the lens, the beam converges at a distance $x$ from the lens. The value of $x$ will be equal to

If the refractive index from air to glass is $\frac{3}{2}$ and that from air to water is $\frac{4}{3}$, then the ratio of focal lengths of a glass lens in water and in air is

Two thin biconvex lenses have focal lengths $f_1$ and $f_2$. A third thin biconcave lens has focal length of $f_3.$ If the two biconvex lenses are in contact, the total power of the lenses is $P_1$. If the first convex lens is in contact with the third lens, the total power is $P_2$. If the second lens is in contact with the third lens, the total power is $P_3$ then

The size of the image of an object, which is at infinity, as formed by a convex lens of focal length $30 \mathrm{cm}$ is $2 \mathrm{cm}$. If a concave lens of focal length $20 \mathrm{cm}$ is placed between the convex lens and the image at a distance of $26 \mathrm{~cm}$ from the lens, the new size of the image is: