Embibe Experts Solutions for Chapter: Surface Areas and Volumes, Exercise 1: Exercise
Embibe Experts Mathematics Solutions for Exercise - Embibe Experts Solutions for Chapter: Surface Areas and Volumes, Exercise 1: Exercise
Attempt the practice questions on Chapter 13: Surface Areas and Volumes, Exercise 1: Exercise with hints and solutions to strengthen your understanding. Mathematics Crash Course (Based on Revised Syllabus-2023) solutions are prepared by Experienced Embibe Experts.
Questions from Embibe Experts Solutions for Chapter: Surface Areas and Volumes, Exercise 1: Exercise with Hints & Solutions
A cone of base radius is divided into two parts by drawing a plane through the mid-point of its height and parallel to its base. Compare the volume of the two parts.
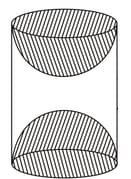
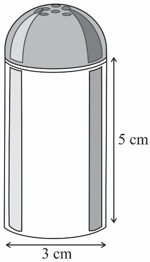
A wooden article was made by scooping out a hemisphere from each end of a solid cylinder, as shown in figure. If the height of the cylinder is , and its base is of radius . Find the total surface area of the article.

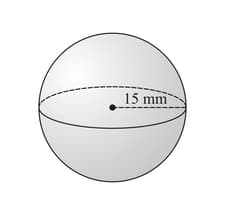
Find the volume and surface area of the following sphere. Round your answers to the nearest tenth .
Find the volume of the following shape.
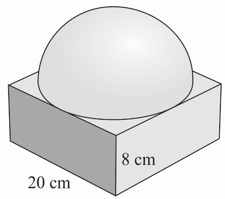
Find the volume of the following shape.
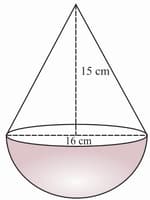
Find the volume of the following shape.
Jumbo circus team is a very famous circus company in south India. They roam around in the different parts of the counts and host the circus treat for the people. When they are settling at a place, they will erect their huge circus tent for performing the show and small tents for them to stay in those days. The stage tent consists of a cylinder capped by a cone.

The height and radius of the base of a conical portion is metre and metre. To make this conical part, find the length of the cloth in metres that is required if the given cloth has a breadth of metres.
Akhil runs a toy manufacturing company. He produces a wide variety of toys suitable for kids of different ages. A wooden toy was made by scooping out a hemisphere from each end of a solid cylinder, as shown in the figure. If the height of the cylinder is , and its base is of radius . Find the total surface area of the toy.