G Tewani Solutions for Chapter: Circles, Exercise 1: DPP

$P$ and $Q$ are any two points on the circle $x^2+y^2=4$, such that $PQ$ is the diameter. If $\alpha$ and $\beta$ are the lengths of the perpendicular from $P$ and $Q$ on $x+y=1$, then the maximum value of $\alpha \beta$ is

Let $A\left(-4,0\right)$ and $B\left(4,0\right)$. Then, the number of points $C$ on the circle $x^2+y^2=16$ lying in the first quadrant, such that, the area of the triangle whose vertices are $A, B$ and $C$ is an integer, is

A triangle is inscribed in a circle of radius $1$. The distance between the orthocenter and the circumcenter of the triangle cannot be

The circle with equation $x^2+y^2=1$ intersects the line $y=7x+5$ at two distinct points $A$ and $B$. Let $C$ be the point at which the positive $x$-axis intersects the circle. The angle $ACB$ is

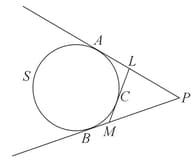
$PA$ and $PB$ are tangents to a circle $S$ touching it at points $A$ and $B$. $C$ is a point on $S$ in between $A$ and $B$ as shown in the figure. $LCM$ is a tangent to $S$ intersecting $PA$ and $PB$ on points $L$ and $M$, respectively. Then the perimeter of the triangle $PLM$ depends on

Two equal chords $AB$ and $AC$ of the circle $x^2+y^2-6x-8y-24=0$ are drawn from the point $A\left(\sqrt{33}+3, 0\right)$. Another chord $PQ$ is drawn intersecting $AB$ and $AC$ at points $R$ and $S$, respectively, given that $AR=SC=7$ and $RB=AS=3.$ The value of $\frac{PR}{QS}$ is

From a point $P$ outside a circle with center at $C,$ tangents $PA$ and $PB$ are drawn such that $\frac{1}{{\left(CA\right)}^2}+\frac{1}{{\left(PA\right)}^2}=\frac{1}{16}$, then the length of chord $AB$ is

$\left(1,2\sqrt{2}\right)$ is a point on circle $x^2+y^2=9$. Which of the following is not a point on the circle at $2$ units distance from $\left(1,2\sqrt{2}\right)$?