Embibe Experts Solutions for Exercise 7: EXERCISE 6.7
Author:Embibe Experts
Embibe Experts Physics Solutions for Exercise - Embibe Experts Solutions for Exercise 7: EXERCISE 6.7
Attempt the free practice questions from Exercise 7: EXERCISE 6.7 with hints and solutions to strengthen your understanding. Gamma Question Bank for Engineering Physics solutions are prepared by Experienced Embibe Experts.
Questions from Embibe Experts Solutions for Exercise 7: EXERCISE 6.7 with Hints & Solutions
HARD
JEE Main/Advance
IMPORTANT
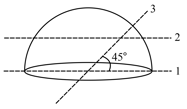
A solid hemisphere has the moment of inertia about axis (Diameter of hemisphere)
MEDIUM
JEE Main/Advance
IMPORTANT
of hemisphere about axis , which is parallel to diameter but passing through centre of mass, will be
HARD
JEE Main/Advance
IMPORTANT
of hemisphere about axis , will be
MEDIUM
JEE Main/Advance
IMPORTANT
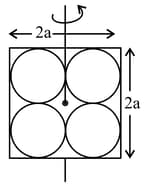
A square plate is of mass and length of edge equal to . Its about its centre of mass axis, perpendicular to its plane is equal to . Four identical disks are cut from the plane. The of leftover square about same axis is