K A Tsokos Solutions for Chapter: Circular Motion and Gravitation, Exercise 1: Test yourself

A cylinder of radius $5.0\mathrm{m}$ rotates about its vertical axis. A girl stands inside the cylinder with her back touching the side of the cylinder. The floor is suddenly lowered but the girl stays 'glued' to the wall. The coefficient of friction between the girl and the wall is $0.60$.

b. Determine the minimum number of revolutions per minute for which the girl does not slip down the wall.

A loop-the-loop machine has a radius of $18\mathrm{m}$.

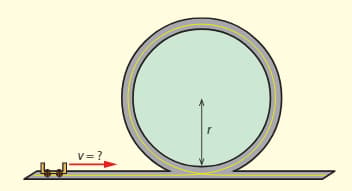
a. Calculate the minimum speed with which a cart must enter the loop so that it does not fall off at the highest point.

A loop-the-loop machine has a radius of $18\mathrm{m}$.

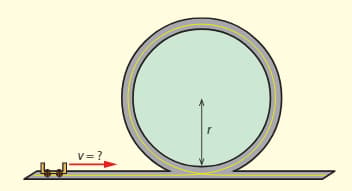
b. Predict the speed at the top in this case.

The diagram shows a horizontal disc with a hole through its centre. A string passes through the hole and connects a mass $m$ on top of the disc to a bigger mass $M$ that hangs below the disc. Initially, the smaller mass is rotating on the disc in a circle of radius $r$. Determine the speed of $m$  be such that the big mass stands still.

,

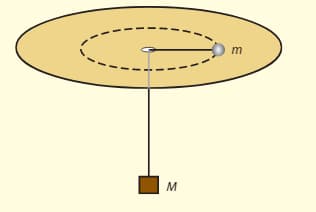
The ball shown in the diagram is attached to a rotating pole with two strings. The ball has a mass of $0.250\mathrm{kg}$ and rotates in a horizontal circle at a speed of $8.0 \mathrm{m}/\mathrm{s}$.

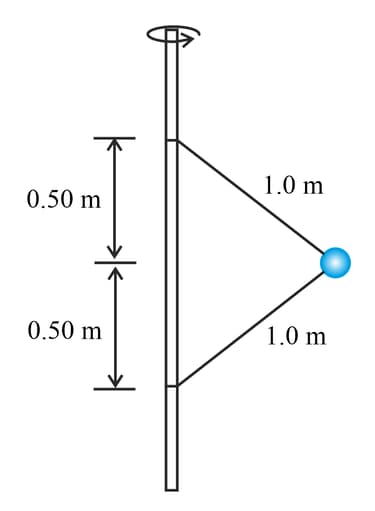
Determine the tension in each string.

In an amusement park ride a cart of mass $300\mathrm{kg}$ and carrying four passengers each of mass $60\mathrm{kg}$ is dropped from a vertical height of $120\mathrm{m}$ along a frictionless path that leads into a loop-the-loop machine of radius $30\mathrm{m}$. The cart then enters a straight stretch from A to C, where friction brings it to rest after a distance of $40\mathrm{m}$.

a. Determine the velocity of the cart at A.

In an amusement park ride a cart of mass $300\mathrm{kg}$ and carrying four passengers each of mass $60\mathrm{kg}$ is dropped from a vertical height of $120\mathrm{m}$ along a frictionless path that leads into a loop-the-loop machine of radius $30\mathrm{m}$. The cart then enters a straight stretch from A to C, where friction brings it to rest after a distance of $40\mathrm{m}$.

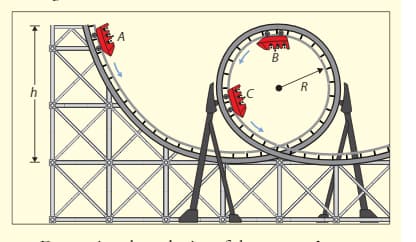
b. Calculate the reaction force from the seat the cart onto the passenger at B.

In an amusement park ride a cart of mass $300\mathrm{kg}$ and carrying four passengers each of mass $60\mathrm{kg}$ is dropped from a vertical height of $120\mathrm{m}$ along a frictionless path that leads into a loop-the-loop machine of radius $30\mathrm{m}$. The cart then enters a straight stretch from A to C, where friction brings it to rest after a distance of $40\mathrm{m}$.

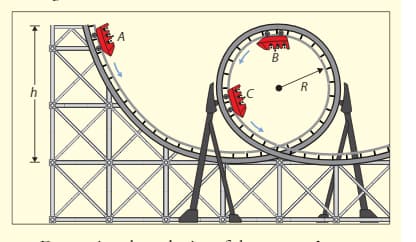
c. Determine the acceleration experienced by the cart from A to C.