Andhra Pradesh Board Solutions for Chapter: Tangents and Secants to a Circle, Exercise 9: EXERCISE - 9.2

Two concentric circles are of radii $5 \mathrm{cm}$ and $3 \mathrm{cm}$ are drawn. Find the length (in $\mathrm{cm}$) of the chord of the larger circle which touches the smaller circle.

Prove that the parallelogram circumscribing a circle is a rhombus.

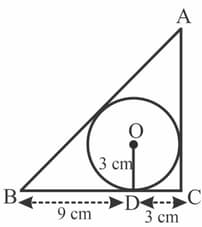
A triangle $\mathrm{ABC}$ is drawn to circumscribe a circle of radius $3 \mathrm{cm}$ such that $\mathrm{BD}=9 \mathrm{cm}$ and $\mathrm{DC}=3 \mathrm{cm}$. Find the sides $\mathrm{AB}$ and $\mathrm{AC}$.

Draw a circle of radius $6 \mathrm{cm}$. From a point $10 \mathrm{cm}$ away from its centre, construct the pair of tangents to the circle and measure their lengths. Verify by using Pythagoras Theorem.

Construct a tangent to a circle of radius $4 \mathrm{cm}$ from a point on the concentric circle of radius $6 \mathrm{cm}$ and measure its length. Also verify the measurement by actual calculation.

Draw a circle with the help of a bangle, take a point outside the circle. Construct the pair of tangents from this point to the circle.

In a right-angled triangle $ABC$, a circle with a side $AB$ as diameter is drawn to intersect the hypotenuse $AC$ in $P$. Prove that the tangent to the circle at $P$ bisects the side $BC$.

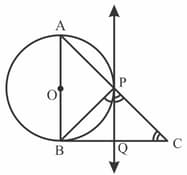
Draw a tangent to a given circle with centre $O$ from a point $R$ outside the circle. How many tangents can be drawn to the circle from that point?