Embibe Experts Solutions for Chapter: Electrostatics, Exercise 1: Exercise 1

Two charges $q$ and $-q$ are placed at $(a, 0)$ and $(-a, 0)$ on the $x-$axis. Another charge $2q$ is taken from $(0, 0)$ to $(0, a)$, then the work done to do so

If a solid and a hollow conducting sphere have same radius then

Two spheres of radii $2 \mathrm{cm}$ and $4 \mathrm{cm}$ are charged equally, then the ratio of charge density on the surfaces of the spheres will be -

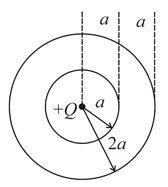
A solid spherical conducting shell has inner radius $a$ and outer radius $2a$. At the center of the shell is located a point charge $+Q$. What must the excess charge of the shell be in order for the charge density on the inner and outer surfaces of the shell to be exactly equal?

Two isolated metallic spheres of radii $2 \mathrm{cm}$ and $4 \mathrm{cm}$ are given equal charge, then the ratio of charge density on the surfaces of the spheres will be

The dimensional formula of linear charge density $\lambda$ is

Assertion: No two electric lines of force can intersect each other.

Reason: Tangent at any point of electric line of force gives the direction of electric field.

The plates of a parallel plate condenser are being moved away with a constant speed $v$. If the plate separation at any instant of time is $d$, then the rate of change of capacitance with time is proportional to,