Resnick & Halliday Solutions for Chapter: Magnetic Fields Due to Currents, Exercise 1: Problems

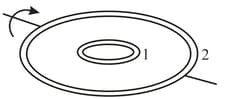
In the figure, two concentric circular loops of wire carrying current in the same direction lie in the same plane. Loop $1$ has radius $1.50 \mathrm{cm}$ and carries $4.00 \mathrm{m}$$\mathrm{A}$ . Loop $2$ has radius $2.50 \mathrm{cm}$ and carries $6.00 \mathrm{mA}$. Loop $2$ is to be rotated about a diameter while the net magnetic field $\overrightarrow{B}$ set up by the two loops at their common center is measured. (a) Through what angle must loop $2$ be rotated so that the magnitude of that net field is $200 \mathrm{nT}?$ (b) What is the least possible magnitude of the net field?

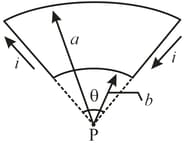
In the given figure, two circular arcs have radii $a=18.9 \mathrm{cm}$ and $b=10.7 \mathrm{cm}$, subtend angle $\theta =74.0°$, carry current $i=0.411 \mathrm{A}$ and share the same center of curvature $P .$ What are the $\left(\mathrm{a}\right)$ magnitude and $\left(\mathrm{b}\right)$ direction (into or out of the page) of the net magnetic field at $P ?$

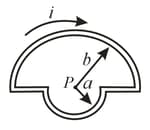
In the figure, current $i=56.2 \mathrm{mA}$ is set up in a loop having two radial lengths and two semicircles of radii $a=5.72 \mathrm{cm}$ and $b=8.57\mathrm{cm}$ with a common center $P .$ What are the (a) magnitude and (b) direction (into or out of the page) of the magnetic field at $P$ and the (c) magnitude and (d) direction of the loop's magnetic dipole moment? 

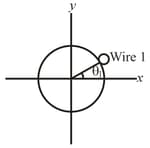
A wire with current $i=9.50 \mathrm{A}$ is shown in the figure. Two semi-infinite straight sections, both tangent to the same circle with radius is $1.65 \mathrm{cm}$, are connected by a circular arc that has a central angle $\mathrm{\theta }$ and runs along the circumference of the circle. The arc and the two straight sections all lie in the same plane. If the magnetic field is $B=0$ at the circle's centre, what is $\mathrm{\theta }?$ 

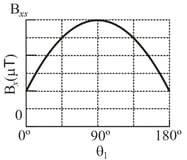
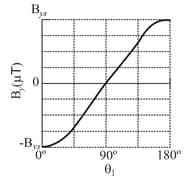
Two long straight thin wires with current lie against an equally long plastic cylinder, at radius $R=4.0 \mathrm{cm}$ from the cylinder's central axis. Figure a shows, in cross-section, the cylinder and wire $1$ but not wire $2 .$ With wire $2$ fixed in place, wire$1$ is moved around the cylinder, from angle ${\theta }_1=0°$ to angle ${\theta }_1=180°,$ through the first and second quadrants of the $xy$ coordinate system. The net magnetic field $\overrightarrow{B}$ at the center of the cylinder is measured as a function of ${\theta }_1$. Figure b gives the $x$ component $B_{\mathrm{x}}$ of that field as a function of ${\theta }_1$ (the vertical scale is set by $B_{\mathrm{xs}}=6.0 \mu \mathrm{T}$), and Fig. c gives the $y$ component $B_{\mathrm{y}}$ (the vertical scale is set by ${\mathrm{B}}_{\mathrm{ys}}=7.2\times {10}^{-6} \mathrm{T}$). (a) At what angle ${\theta }_2$ is wire $2$ located? What are the (b) size and (c) direction (into or out of the page) of the current in wire $1$ and the (d) size and (e) direction of the current in wire $2 ?$ 

$\left(\mathrm{a}\right)$ 

$\left(\mathrm{b}\right)$ 

$\left(\mathrm{c}\right)$ 

Two long straight wires are parallel and are $16 \mathrm{cm}$ apart. They are to carry equal currents such that the magnetic field at a point halfway between them has the magnitude of $450 \mathrm{\mu T}$.  

$\left(\mathrm{a}\right)$ Should the currents be in the same or opposite directions?
$\left(\mathrm{b}\right)$ How much current is needed?

The figure shows, in cross-section, four thin wires that are parallel, straight, and very long. They carry identical currents in the directions indicated. Initially, all four wires are at distance $d=15.0 \mathrm{cm}$ from the origin of the coordinate system, where they create a net magnetic field $\overrightarrow{B}$. $\left(\mathrm{a}\right)$ To what value of $x$ must you move wire $1$ along the $x$ axis in order to rotate $\overrightarrow{B}$ counterclockwise by $50°?$ $\left(\mathrm{b}\right)$ With wire $1$in that new position, to what value of $x$ must you move wire $3$ along the$x$ axis to rotate $\overrightarrow{\mathit{B}}$ by $50°$ back to its initial orientation? [Use $\tan \left(50°\right)=1.192$]

 At a certain location in the Philippines, Earth's magnetic field of  $39 \mu \mathrm{T}$ is horizontal and directed due north. Suppose the net field is zero exactly $2.0 \mathrm{cm}$ above a long, straight, horizontal wire that carries a constant current. What are the $\left(\mathrm{a}\right)$ magnitude and $\left(\mathrm{b}\right)$ direction of the current?