Rose Harrison, Clara Huizink, Aidan Sproat Clements and, Marlene Torres Skoumal Solutions for Exercise 4: Practice 1
Rose Harrison Mathematics Solutions for Exercise - Rose Harrison, Clara Huizink, Aidan Sproat Clements and, Marlene Torres Skoumal Solutions for Exercise 4: Practice 1
Attempt the free practice questions from Exercise 4: Practice 1 with hints and solutions to strengthen your understanding. Extended MYP Mathematics A Concept based approach Years 4 & 5 solutions are prepared by Experienced Embibe Experts.
Questions from Rose Harrison, Clara Huizink, Aidan Sproat Clements and, Marlene Torres Skoumal Solutions for Exercise 4: Practice 1 with Hints & Solutions
Here is a standard set of dominos.

One domino is selected at random. It is then replaced and a second domino is drawn at random. If is the set of outcomes of selecting dominos, write down .
Here is a standard set of dominos.

One domino is selected at random. It is then replaced and a second domino is drawn at random. If is the set of outcomes of selecting dominos, write down . Let be the event 'at least one of the values on the first domino is a six' and be the event 'the second domino is a double' (both values are the same). Explain whether or not these events are independent.
Here is a standard set of dominos.
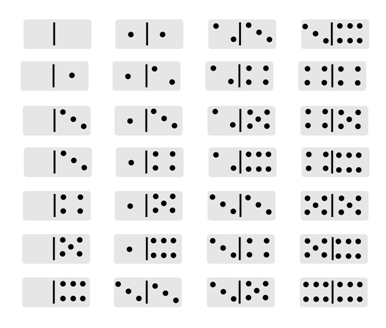
One domino is selected at random. It is then replaced and a second domino is drawn at random. If is the set of outcomes of selecting dominos, write down . Let be the event 'at least one of the values on the first domino is a six' and be the event 'the second domino is a double' (both values are the same). Explain whether or not these events are independent. Verify your answer using the multiplication rule.
In a different class, four students take Mathematics only, two take both Mathematics and Science, six take Science only and take neither subject. Determine whether the choice of taking Mathematics and taking Science ar independent events for this class.
Students in an after-school activity programme register for either trampolining or table tennis. The table shows students' choices by gender:
| Trampolining | Table tennis | Total | |
| Male | 39 | 16 | 55 |
| Female | 21 | 14 | 35 |
A student is selected at random from the group. Find:
Students in an after-school activity programme register for either trampolining or table tennis. The table shows students' choices by gender:
| Trampolining | Table tennis | Total | |
| Male | 39 | 16 | 55 |
| Female | 21 | 14 | 35 |
A student is selected at random from the group. Find:
Students in an after-school activity programme register for either trampolining or table tennis. The table shows students' choices by gender:
| Trampolining | Table tennis | Total | |
| Male | 39 | 16 | 55 |
| Female | 21 | 14 | 35 |
A student is selected at random from the group. Find:
Students in an after-school activity programme register for either trampolining or table tennis. The table shows students' choices by gender:
| Trampolining | Table tennis | Total | |
| Male | 39 | 16 | 55 |
| Female | 21 | 14 | 35 |
A student is selected at random from the group. Determine whether or not the events trampolining and table tennis are independent events.
