Question

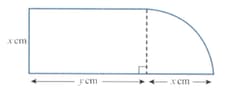
The diagram shows a metal plate consisting of a rectangle with sides $x$ cm and $y$ cm and a quarter-circle of radius $x$ cm. The perimeter of the plate is $60$ cm.Given that $x$ can vary, find this stationary value of $A$ and determine whether it is a maximum or a minimum value.

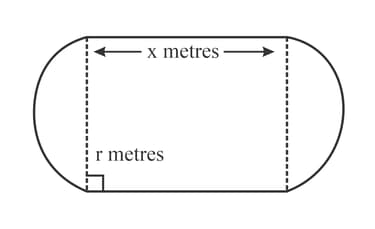
Question Image

Accepted Answer

Given that, we have:

Perimeter is the total length of the boundary of a closed figure.
We know that the total length of the boundary of a plane closed figure is called its perimeter.

Now, total perimeter of metal plate $P = 2 x + 2 y + \frac{π x}{2}$ i.e., $P = 2 x + 2 y + \frac{π x}{2} = 60$

$\Rightarrow 2 y = 60 - 2 x - \frac{π x}{2}$

$\Rightarrow y = 30 - x - \frac{π x}{4}$

Now, the area of the plate is $A = x y + \frac{1}{4} π x^{2}$

$\Rightarrow A = x (30 - x - \frac{π x}{4}) + \frac{1}{4} π x^{2}$ [Using $y = 30 - x - \frac{π x}{4}$ ]

$\Rightarrow A = 30 x - x^{2} - \frac{π x^{2}}{4} + \frac{1}{4} π x^{2}$

$\Rightarrow A = 30 x - x^{2}$

Now, differentiate $A$ with respect to $x$ , then we get: $\frac{d A}{d x} = \frac{d}{d x} (30 x - x^{2})$

$\Rightarrow \frac{d A}{d x} = 30 \frac{d}{d x} (x) - \frac{d}{d x} (x^{2})$

$\Rightarrow \frac{d A}{d x} = 30 - 2 x \to (1)$

For stationary points: we have, $\frac{d A}{d x} = 0$

Therefore, $30 - 2 x = 0$

$\Rightarrow 30 = 2 x$

$\Rightarrow x = 15$

Again differentiate $(1)$ with respect to $x$ , then $\frac{d^{2} A}{d x^{2}} = \frac{d}{d x} (30 - 2 x)$

$\Rightarrow \frac{d^{2} A}{d x^{2}} = \frac{d}{d x} (30) - 2 \frac{d}{d x} (x)$

$\Rightarrow \frac{d^{2} A}{d x^{2}} = - 2$

When $x = 15$ , then ${\frac{d^{2} A}{d x^{2}}}_{x = 15} = - 2 < 0$

Hence, we have maximum value at $x = 15$ .

Sue Pemberton Solutions for Chapter: Further Differentiation, Exercise 9: END-OF-CHAPTER REVIEW EXERCISE 8

Sue Pemberton Mathematics Solutions for Exercise - Sue Pemberton Solutions for Chapter: Further Differentiation, Exercise 9: END-OF-CHAPTER REVIEW EXERCISE 8

Questions from Sue Pemberton Solutions for Chapter: Further Differentiation, Exercise 9: END-OF-CHAPTER REVIEW EXERCISE 8 with Hints & Solutions

Learn and Prepare for any exam you want !