The position vector a is such that a = 4 i - 8 j + k . Find a · j and hence find the angle that a makes with the positive y -axis.

Given that: The position vector a is such that a = 4 i - 8 j + k . We have to find a · j and hence find the angle that a makes with the positive y -axis. Let the angle between them be θ As we know that, a → · b → = a b cos θ ⇒ cos θ = a → · b → a b So, cos θ = 4 × 0 - 8 × 1 + 1 × 0 4 2 + - 8 2 + 1 2 0 2 + 1 2 + 0 2 ⇒ cos θ = - 8 81 1 = - 8 9 ⇒ θ = cos - 1 - 8 9 = 152 . 733 ° ≈ 152 . 7 ° So, the required angle is 152 . 7 ° .

Explain why a · b · c has no meaning.

We have to explain why a · b · c has no meaning. a · b is a scalar and the dot product is a product of two vectors a · b · c is dot product is a product of scalar and vector has no meaning.

The diagram shows a cube O A B C D E F G with side length 4 units. The unit vectors i , j and k are parallel to O A → , O C → and O D → , respectively. The point M is the midpoint of G F and A N : N B is 1 : 3 . Find each of the vectors O M → and N G → .

Given that: The diagram shows a cube O A B C D E F G with side length 4 units. We have to find each of the vectors O M → and N G → . From the given figure, M = 2 i + 4 j + 4 k The point M is the midpoint of G F and A N : N B is 1 : 3 . N = 4 i + j G = 4 j + 4 k O M → = O M → - O O → = 2 i + 4 j + 4 k N G → = 0 - 4 i + 4 - 1 j + 4 - 0 k = - 4 i + 3 j + 4 k So, O M → = 2 i + 4 j + 4 k and N G → = - 4 i + 3 j + 4 k .

The diagram shows a cube O A B C D E F G with side length 4 units. The unit vectors i , j and k are parallel to O A → , O C → and O D → , respectively. The point M is the midpoint of G F and A N : N B is 1 : 3 . Find the angle between the directions O M → and N G → .

Given that: The diagram shows a cube O A B C D E F G with side length 4 units. We have to find the angle between the directions O M → and N G → . From the given figure, M = 2 i + 4 j + 4 k The point M is the midpoint of G F and A N : N B is 1 : 3 . N = 4 i + j G = 4 j + 4 k So, O M → = 2 i + 4 j + 4 k and N G → = - 4 i + 3 j + 4 k Let angle between them is θ Now, cos θ = O M → N G → O M → · N G → So, cos θ = 2 × - 4 + 4 × 3 + 4 × 4 2 2 + 4 2 + 4 2 - 4 2 + 3 2 + 4 2 ⇒ cos θ = 20 6 41 ⇒ θ = cos - 1 20 6 41 Hence, the required angle between them is 58 . 6 ° .

The diagram shows a pyramid V O A B C . The base of the pyramid, O A B C , is a rectangle. The unit vectors i , j and k are parallel to O A → , O C → and O V → , respectively. The position vectors of the points A , B , C and V are given by O A → = 6 i , O C → = 3 j and O V → → = 9 k . The points M and N are the midpoints of A B and B V , respectively. Find the vector M N → .

Given that: the position vectors of the points A , B , C and V are given by O A → = 6 i , O C → = 3 j and O V → → = 9 k . We have to find the vector M N → From the given figure, The points M and N are the midpoints of A B and B V O N → = 3 1 . 5 4 . 5 and O M → = 6 1 . 5 0 Now, M N → = O N → - O M → So, M N → = 3 1 . 5 4 . 5 - 6 1 . 5 0 = - 3 0 4 . 5 Hence, the required vector M N → = - 3 0 4 . 5 .

The diagram shows a pyramid V O A B C . The base of the pyramid, O A B C , is a rectangle. The unit vectors i , j and k are parallel to O A → , O C → and O V → , respectively. The position vectors of the points A , B , C and V are given by O A → = 6 i , O C → = 3 j and O V → → = 9 k . The points M and N are the midpoints of A B and B V , respectively. The point P lies on O V and is such that P N M is a right angle. Find the position vector of the point P .

Given that: the position vectors of the points A , B , C and V are given by O A → = 6 i , O C → = 3 j and O V → → = 9 k . We have to find the position vector of the point P . From the given figure, The points M and N are the midpoints of A B and B V O N → = 3 1 . 5 4 . 5 and O M → = 6 1 . 5 0 Now, M N → = O N → - O M → So, M N → = 3 1 . 5 4 . 5 - 6 1 . 5 0 = - 3 0 4 . 5 Therefore, the required vector M N → = - 3 0 4 . 5 . Since,the point P lies on O V and is such that P N M is a right angle. P N → · M N → = 0 , P N → = 3 1 . 5 4 . 5 - p So, 3 - 3 + 4 . 5 4 . 5 - p = 0 ⇒ p = 2 . 5 Hence, the position vector of O P → = 0 0 2 . 5 .

Sue Pemberton, Julianne Hughes and, Julian Gilbey Solutions for Chapter: Vectors, Exercise 7: EXERCISE 9C

Author:Sue Pemberton, Julianne Hughes & Julian Gilbey

Sue Pemberton Mathematics Solutions for Exercise - Sue Pemberton, Julianne Hughes and, Julian Gilbey Solutions for Chapter: Vectors, Exercise 7: EXERCISE 9C

Attempt the practice questions on Chapter 9: Vectors, Exercise 7: EXERCISE 9C with hints and solutions to strengthen your understanding. Cambridge International AS & A Level Mathematics : Pure Mathematics 2 & 3 Course Book solutions are prepared by Experienced Embibe Experts.

Questions from Sue Pemberton, Julianne Hughes and, Julian Gilbey Solutions for Chapter: Vectors, Exercise 7: EXERCISE 9C with Hints & Solutions

HARD

AS and A Level

IMPORTANT

Cambridge International AS & A Level Mathematics : Pure Mathematics 2 & 3 Course Book>Chapter 9 - Vectors>EXERCISE 9C>Q 6

The position vector $a$ is such that $a = 4 i - 8 j + k .$ Find $a \cdot j$ and hence find the angle that a makes with the positive $y$ -axis.

EASY

AS and A Level

IMPORTANT

Cambridge International AS & A Level Mathematics : Pure Mathematics 2 & 3 Course Book>Chapter 9 - Vectors>EXERCISE 9C>Q 7

Explain why $a \cdot b \cdot c$ has no meaning.

MEDIUM

AS and A Level

IMPORTANT

Cambridge International AS & A Level Mathematics : Pure Mathematics 2 & 3 Course Book>Chapter 9 - Vectors>EXERCISE 9C>Q 8

The diagram shows a cube $O A B C D E F G$ with side length $4$ units. The unit vectors $i, j$ and $k$ are parallel to $\vec{O A}, \vec{O C}$ and $\vec{O D},$ respectively. The point $M$ is the midpoint of $G F$ and $A N : N B$ is $1 : 3 .$

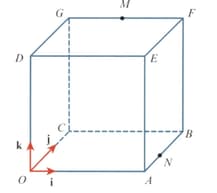
Question Image

Find each of the vectors $\vec{O M}$ and $\vec{N G} .$

HARD

AS and A Level

IMPORTANT

Cambridge International AS & A Level Mathematics : Pure Mathematics 2 & 3 Course Book>Chapter 9 - Vectors>EXERCISE 9C>Q 8

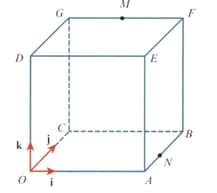
Question Image

Find the angle between the directions $\vec{O M}$ and $\vec{N G} .$

HARD

AS and A Level

IMPORTANT

Cambridge International AS & A Level Mathematics : Pure Mathematics 2 & 3 Course Book>Chapter 9 - Vectors>EXERCISE 9C>Q 9

The diagram shows a triangular prism, $O A B C D E .$ The uniform cross-section of the prism, $O A E,$ is a right-angled triangle with base $77 cm$ and height $36 cm .$ The length, $A B,$ of the prism is $60 cm .$ The unit vectors $i, j$ and $k$ are parallel to $\vec{O A}, \vec{O C}$ and $\vec{O E},$ respectively. The point $N$ divides the length of the line $D B$ in the ratio $4 : 1$ and $M$ is the midpoint of $D E .$

Question Image

Use a scalar product to find angle $M A N .$

HARD

AS and A Level

IMPORTANT

Cambridge International AS & A Level Mathematics : Pure Mathematics 2 & 3 Course Book>Chapter 9 - Vectors>EXERCISE 9C>Q 10

The diagram shows a pyramid $V O A B C .$ The base of the pyramid, $O A B C,$ is a rectangle. The unit vectors $i, j$ and $k$ are parallel to $\vec{O A}, \vec{O C}$ and $\vec{O V},$ respectively. The position vectors of the points $A, B, C$ and $V$ are given by
$\vec{O A} = 6 i, \vec{O C} = 3 j and \vec{O \vec{V}} = 9 k .$
The points $M$ and $N$ are the midpoints of $A B$ and $B V,$ respectively.

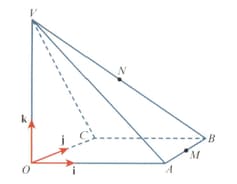
Question Image

Find the angle between the directions of $\vec{A N}$ and $\vec{O C} .$

MEDIUM

AS and A Level

IMPORTANT

Cambridge International AS & A Level Mathematics : Pure Mathematics 2 & 3 Course Book>Chapter 9 - Vectors>EXERCISE 9C>Q 10

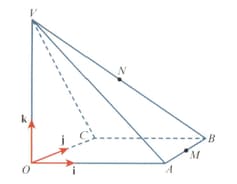
Question Image

Find the vector $\vec{M N} .$

HARD

AS and A Level

IMPORTANT

Cambridge International AS & A Level Mathematics : Pure Mathematics 2 & 3 Course Book>Chapter 9 - Vectors>EXERCISE 9C>Q 10

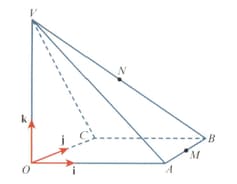
Question Image

The point $P$ lies on $O V$ and is such that $P N M$ is a right angle. Find the position vector of the point $P .$

Sue Pemberton, Julianne Hughes and, Julian Gilbey Solutions for Chapter: Vectors, Exercise 7: EXERCISE 9C

Sue Pemberton Mathematics Solutions for Exercise - Sue Pemberton, Julianne Hughes and, Julian Gilbey Solutions for Chapter: Vectors, Exercise 7: EXERCISE 9C

Questions from Sue Pemberton, Julianne Hughes and, Julian Gilbey Solutions for Chapter: Vectors, Exercise 7: EXERCISE 9C with Hints & Solutions

Learn and Prepare for any exam you want !