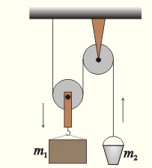
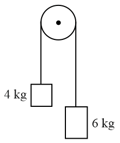
A bucket with mass${\mathrm{m}}_2$ and a block with mass ${\mathrm{m}}_1$ are hung on a pulley system. Find the magnitude of the acceleration with which the bucket and the block are moving and the magnitude of the tension force $\mathrm{T}$ by which the rope is stressed. Ignore the masses of the pulley system and the rope. The bucket moves up and the block moves down.

Two weights of the mass $m_1$ and $m_2\left(>m_1\right)$ are joined by an inextensible string of negligible mass passing over a fixed frictionless pulley. The magnitude of the acceleration of the loads is

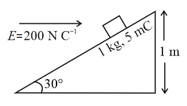
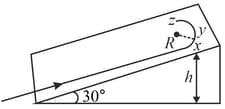
An inclined plane making an angle of $30°$ with the horizontal is placed in a uniform horizontal electric field $200\frac{\mathrm{N}}{\mathrm{C}}$ as shown in the figure. A body of mass $1 \mathrm{kg}$ and charge $5 \mathrm{mC}$ is allowed to slide down from rest at a height of $1 \mathrm{m}$. If the coefficient of friction is $0.2,$ find the time taken by the body to reach the bottom.
$\left[g=9.8 \mathrm{m} {\mathrm{s}}^{-2}; \sin 30°=\frac{1}{2}; \cos 30°=\frac{\sqrt{3}}{2}\right]$

Two blocks $A$ and $B$ of masses $1.5 \mathrm{kg}$ and $0.5 \mathrm{kg}$ respectively are connected by a massless inextensible string passing over a frictionless pulley as shown in the figure. Block A is lifted until block B touches the ground and then block A is released. The initial height of block A is $80 \mathrm{cm}$ when block B just touches the ground. The maximum height reached by block $\mathrm{B}$ from the ground after the block A falls on the ground is

A student skates up a ramp that makes an angle $30°$ with the horizontal. He/she starts (as shown in the figure) at the bottom of the ramp with speed $v_0$ and wants to turn around over a semicircular path $xyz$ of radius $R$ during which he/she reaches a maximum height $h$ (at point $y$) from the ground as shown in the figure. Assume that the energy loss is negligible and the force required for this turn at the highest point is provided by his/her weight only. Then ($g$ is the acceleration due to gravity)

A crate of mass $20 \mathrm{kg}$ is pulled vertically upwards using a rope that passes over a first pulley, under a second pulley and over a third pulley. At the other end of the rope there is a ball of mass $30 \mathrm{kg}.$ Each pulley has mass $m \mathrm{kg}.$ The first and third pulleys are fixed at the same horizontal level and are $4 \mathrm{m}$ apart. The second pulley is an equal distance from the first and third pulleys and hangs at a distance $2 \mathrm{m}$ below them. It is not fixed, but it does not move.

What modelling assumptions need to be made? Which of these assumptions is unlikely to affect the equilibrium of the second pulley?

Represent the union of two sets by Venn diagram for each of the following.

$X=\{x | x$ is a prime number between $80$ and $100\}$

$Y=\{y | y$ is an odd number between $90$ and $100\}$