A circle touches all the four sides of a quadrilateral . Prove that
Important Questions on Circle
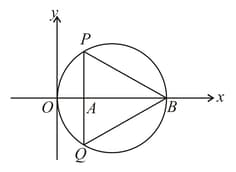
On the circle with center , points are such that . A point is located on the tangent at to the circle such that and are on the opposite sides of the line and . The line segment intersects the circle again at . Then the ratio is equal to -
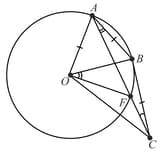
Suppose are two unequal circles; and are the direct common tangents to these circles. A transverse common tangent cuts in and in . If units, then is -
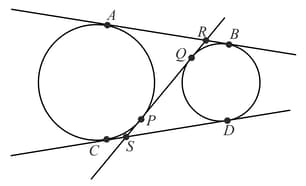
In the given figure, is the centre of the circle and then, the measure of _____.
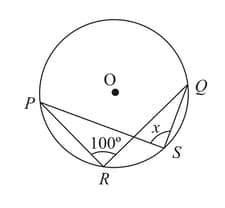
In the given figure, the measure of _____ degree.
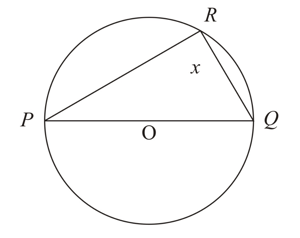
The angle between the tangent at a point on a circle and the radius through the point is _____.
Prove that if a point is marked on each side of a triangle , then the three Miquel circles (each through a vertex and the two marked points on the adjacent sides) are concurrent at a point called the Miquel point.

is the centre of the circle and then the measure of _____
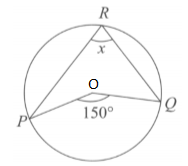
In the given figure, _____ degree.
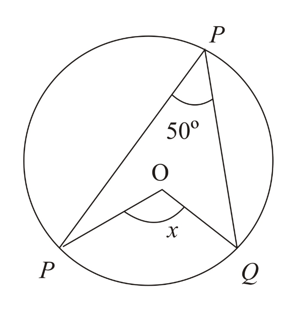
In the given figure, find the measurement of the unknown angle in the circle with centre .
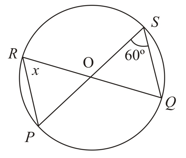
If , then _____.
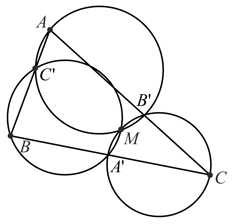
Prove that if be the points on the sides of , respectively, then the circles and pass through a common point. (called the Miquel point)

