A coin is biased such that the probability that three tosses all result in heads is $\frac{125}{512}$. Find the probability of obtaining no heads with three tosses of the coin.

In a group of five men and four women, there are three pairs of male and female business partners and three teachers, where no teacher is in a business partnership. One man and one woman are selected at random. Find the probability that they are:

both teachers

In a group of five men and four women, there are three pairs of male and female business partners and three teachers, where no teacher is in a business partnership. One man and one woman are selected at random. Find the probability that they are:

in a business partnership with each other

In a group of five men and four women, there are three pairs of male and female business partners and three teachers, where no teacher is in a business partnership. One man and one woman are selected at random. Find the probability that they are:

each in a business partnership but not with each other.

A biased die in the shape of a pyramid has five faces marked $1, 2, 3, 4$ and $5$ . The possible scores are $1, 2, 3, 4$ and $5$ and $\mathrm{P}\left(x\right)=\frac{k-x}{25}$, where $k$ is a constant.

Find, in terms of $k$, the probability of scoring $5$.

A biased die in the shape of a pyramid has five faces marked $1, 2, 3, 4$ and $5$ . The possible scores are $1, 2, 3, 4$ and $5$ and $\mathrm{P}\left(\mathrm{x}\right)=\frac{k-x}{25}$, where $k$ is a constant.

Find, in terms of $k$, the probability of scoring less than $3$ .

A biased die in the shape of a pyramid has five faces marked $1, 2, 3, 4$ and $5$ . The possible scores are $1, 2, 3, 4$ and $5$ and $\mathrm{P}\left(\mathrm{x}\right)=\frac{k-x}{25}$, where $k$ is a constant.

The die is rolled three times and the scores are added together. Evaluate $k$ and find the probability that the sum of the three scores is less than $5$ .

A game board is shown in the diagram.

Players take turns to roll an ordinary fair die, then move their counters forward from 'start' a number of squares equal to the number rolled with the die. If a player's counter ends its move on a coloured square, then it is moved back to the start.

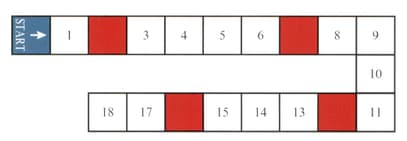
Find the probability that a player's counter is on 'start' after rolling the die once.

A game board is shown in the diagram.

Players take turns to roll an ordinary fair die, then move their counters forward from 'start' a number of squares equal to the number rolled with the die. If a player's counter ends its move on a coloured square, then it is moved back to the start.

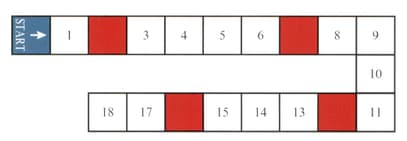
Find the probability that a player's counter is on 'start' after rolling the die twice.