MEDIUM
10th ICSE
IMPORTANT
Earn 100
A cone is filled of water poured into the hemisphere, which is also filled. The radius and height of a cone are and , respectively. Find the radius of the hemisphere (in ).
50% studentsanswered this correctly

Important Questions on Surface Area and Volume
HARD
10th ICSE
IMPORTANT
HARD
10th ICSE
IMPORTANT
HARD
10th ICSE
IMPORTANT
MEDIUM
10th ICSE
IMPORTANT
MEDIUM
10th ICSE
IMPORTANT
The water for a factory is stored in a hemispherical tank, whose internal diameter is . The tank contains of water. Water is pumped into the tank to fill to its capacity. If the volume of water pumped into the tank is .
Then find the value of correct to two decimal places.
[Take ]
MEDIUM
10th ICSE
IMPORTANT
A wooden article was made by scooping out a hemisphere from each end of a solid cylinder, as shown in the figure. If the height of the cylinder is and its base is of radius , then if the total surface area of the article is , find the value of . [Take ]
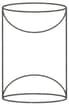
MEDIUM
10th ICSE
IMPORTANT
MEDIUM
10th ICSE
IMPORTANT
