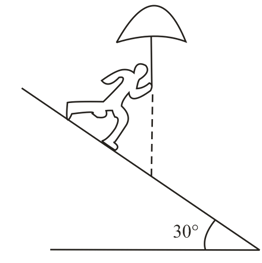
A man is coming down an incline of angle $30°$. When he walks with speed $2\sqrt{3} \mathrm{m}{ \mathrm{s}}^{-1}$, he has to keep his umbrella vertical to protect himself from rain. The actual speed of rain is $5{ \mathrm{m} \mathrm{s}}^{-1}$. At what angle with vertical should he keep his umbrella when he is at rest so that he does not get drenched?

A helicopter flies horizontally with constant velocity in a direction $\mathrm{\theta }$ east of north between two points $A$ and $B$, at distance $d$ apart. Wind is blowing from south with constant speed $u$, the speed of helicopter relative to air is $nu$, where $n>1$. Find the speed of the helicopter along $AB$. The helicopter returns from $B$ to $A$ with same speed $nu$ relative to air in same wind. Find the total time for the journeys.

$A, B, C,$ and $D$ are four trees, located at the vertices of a square. Wind blows from $A$ to $B$ with uniform speed. The ratio of times of flight of a bird from $A$ to $B$ and from $B$ to $A$ is $n$. At what angle should the bird fly from the direction of wind flow, in order that it starts from $A$ and

(a) reaches $C$,

(b) reaches $D$?

Two particles are located on a horizontal plane at a distance $60 \mathrm{m}$. At $t=0$ both the particles are simultaneously projected at angle $45°$ with velocities $2{ \mathrm{m} \mathrm{s}}^{-1}$ and $14{ \mathrm{m} \mathrm{s}}^{-1}$, respectively.

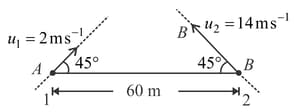
$\left(\mathrm{a}\right)$ Find the minimum separation between them during motion.
$\left(\mathrm{b}\right)$ At what time is the separation between them minimum?

Two towers $AB$ and $CD$ are situated at distance $d$ apart as shown in figure. $AB$ is $20 \mathrm{m}$ high and $CD$ is $30 \mathrm{m}$ high from the ground. A particle is thrown from the top of $AB$ horizontally with a velocity of $10{ \mathrm{m} \mathrm{s}}^{-1}$ towards $CD$. Simultaneously, another particle is thrown from the top of $CD$ at an angle $60°$ to the horizontal towards $AB$ with the same magnitude of initial velocity as that of the first object. The two particles moving in the same vertical plane collide in mid-air. Calculate the distance $d$ between the towers.

Two cars $A$ and $B$ are moving west to east and south to north, respectively, along crossroads. A moves with a speed of $20 \mathrm{m} {\mathrm{s}}^{-1}$ and is $500 \mathrm{m}$ away from the point of intersection of cross roads and $B$ moves with a speed of $15{ \mathrm{m} \mathrm{s}}^{-1}$ and is $400 \mathrm{m}$ away from the point of intersection of cross roads. Find the shortest distance between them.

Two particles $A$ and $B$ are moving with uniform velocity as shown in figure given below at $t=0$.

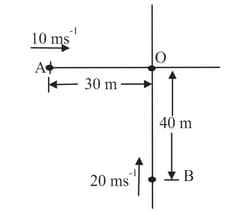
(a) Will the two particles collide?
(b) Find out the shortest distance between two particles.