A metal sphere of radius $30 \mathrm{cm}$ has a net charge of $3.0\times {10}^{-8} \mathrm{C}$. $\left(\mathrm{a}\right)$ What is the electric field at the sphere's surface? $\left(\mathrm{b}\right)$ If $V=0$ at infinity, what is the electric potential at the sphere's surface? $\left(\mathrm{c}\right)$ At what distance from the sphere's surface has the electric potential decreased by $500 \mathrm{V}$?

Two metal spheres, each of radius $3.0 \mathrm{cm}$, have a centre-to-centre separation of $2.0 \mathrm{m}$. Sphere $1$has charge $+1.0\times {10}^{-8} \mathrm{C}$ and sphere $2$ has charge $-8.0\times {10}^{-8} \mathrm{C}$. Assume that the separation is large enough for us to say that the charge on each sphere is uniformly distributed (the spheres do not affect each other). With $V=0$ at infinity, calculate $\left(a\right)$ the potential at the point halfway between the centres and the potential on the surface of $\left(b\right)$ sphere $1$and $\left(c\right)$ sphere $2$. 

A graph of $x$-component of electric field as a function of $x$, in a region of space, is shown in the figure. The scale of the vertical axis is set by $E_{\mathrm{xs}}=10.0 \mathrm{N} {\mathrm{C}}^{-1}$. The $y$ and $z$ components of electric field are zero in this region. If the electric potential at the origin is $10 \mathrm{V}$, $\left(a\right)$ what is the electric potential at $x=2.0 \mathrm{m}$, $\left(b\right)$ what is the greatest positive value of the electric potential for points on the $x$-axis, for which  $0\le x\le 6.0 \mathrm{m}$, and $\left(c\right)$ for what value of $x$ is the electric potential zero?

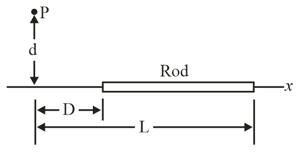
The figure shows a thin rod with a uniform charge density of $1.00 \text{μ}\mathrm{C} {\mathrm{m}}^{-1}$. Evaluate the electric potential at the point $P$ if $d=D=\frac{L}{4.00}$. Assume that the potential is zero at infinity.