HARD

Earn 100

A monatomic ideal gas sample is given heat $Q$ . One half of this heat is used as work done by the gas and rest is used for increasing its internal energy. The equation of process in terms of volume and temperature is

50% studentsanswered this correctly

Important Questions on Thermodynamics

MEDIUM

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

Under an adiabatic process, the volume of an ideal gas gets doubled. Consequently, the mean collision time between the gas molecule changes from

τ_{1}

τ_{2}

. If

\frac{C_{P}}{C_{v}} = γ

for this gas then a good estimate for

\frac{τ_{2}}{τ_{1}}

is given by

HARD

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

A gas is enclosed in a cylinder with a movable frictionless piston. Its initial thermodynamic state at pressure

P_{i} = 10^{5} Pa and volume V_{i} = 10^{- 3} m^{3}

changes to a final state at

P_{f} = (\frac{1}{32}) \times 10^{5} Pa and V_{f} = 8 \times 10^{- 3} m^{3}

in an adiabatic quasi-static process, such that

P^{3} V^{5} =

constant. Consider another thermodynamic process that brings the system from the same initial state to the same final state in two steps: an isobaric expansion at

P_{i}

followed by an isochoric (isovolumetric) process at volumes

V_{f}

. The amount of heat supplied to the system in the two step process is approximately

MEDIUM

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

A sample of an ideal gas is taken through the cyclic process

a b c a

as shown in the figure. The change in the internal energy of the gas along the path

c a

- 180 J .

The gas absorbs

250 J

of heat along the path

a b

and

60 J

along the path

b c

. The work done by the gas along the path

a b c

is:

EASY

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

Work done on (or) by a thermodynamic system is zero in which of the following processes?

HARD

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

The equation of state of $n$ moles of a non-ideal gas can be approximated by the equation $(p + \frac{n^{2} a}{V^{2}}) (V - n b) = n R T$ where, $a$ and, $b$ are constant characteristics of the gas. Which of the following can represent the equation of a quasi-static adiabatic for this gas (assume that, $C_{V}$ is the molar heat capacity at constant volume is independent of temperature)?

EASY

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

When a system is taken from state $i$ to state $f$ along the path $i a f$ , it is found that $Q = 50 cal$ and $W = 20 cal$ . Along the path $i b f$ , $Q' = 36 cal$ . $W$ along the path $i b f$ is,

Question Image

EASY

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

n

moles of an ideal gas with constant volume heat capacity

C_{v}

undergo an isobaric expansion by certain volume. The ratio of the work done in the process, to the heat supplied is:

HARD

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

Certain quantity of heat is supplied to a monatomic ideal gas which expands at constant pressure. The percentage of heat that is used to do work by the gas is

EASY

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

The ratio of work done by an ideal monoatomic gas to the heat supplied to it in an isobaric process is

HARD

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

An ideal gas is undergoing a cyclic thermodynamic process in different ways as shown in the corresponding P-V diagrams in column 3 of the table. Consider only the path from state 1 to state 2. W denotes the corresponding work done on the system. The equations and plots in the table have standard notations as used in thermodynamic process. Here

γ

is the ratio of heat capacities at constant pressure and constant volume. The number of moles in the gas is n.

Column – 1	Column – 2	Column – 3
(I) $W_{1 \to 2} = \frac{1}{γ - 1} (P_{2} V_{2} - P_{1} V_{1})$	(i) Isothermal	(P)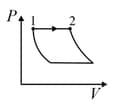
(II) $W_{1 \to 2} = - P V_{2} + P V_{1}$	(ii) Isochoric	(Q)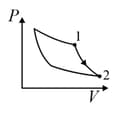
(III) $W_{1 \to 2} = 0$	(iii) Isobaric	(R)
(IV) $W_{1 \to 2} = - n R T \ln (\frac{V_{2}}{V_{1}})$	(iv) Adiabatic	(S)

Which of the following options is the only correct representation of the process

∆ U = ∆ Q - P ∆ V

MEDIUM

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

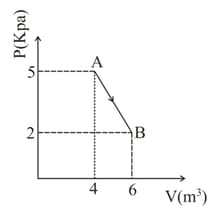
One mole of an ideal diatomic gas undergoes a transition from $A$ to $B$ along a path $A B$ as shown in figure. The change in internal energy of the gas during the transition is

Question Image

MEDIUM

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

In a thermodynamic system,

Q

represents the energy transferred to or from a system by heat and

W

represents the energy transferred to or from a system by work

(I)

Q > 0

and

W = 0

(II)

Q < 0

and

W = 0

(III)

W > 0

and

Q = 0

(IV)

W < 0

and

Q = 0

Which of the above will lead to an increase in the internal energy of the system?

MEDIUM

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

The $P - V$ diagram shown below indicates two paths along which a sample of gas can be taken from state $A$ to state $B$ . The energy equal to $5 P V$ in the form of heat is required to be transferred if the Path- $1$ is chosen. How much energy in the form of heat should be transferred if Path- $2$ is chosen?

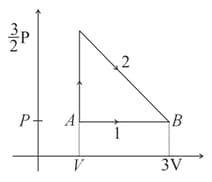
Question Image

MEDIUM

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

Match the thermodynamics processes taking place in a system with the correct conditions. In the table : $∆ Q$ is the heat supplied, $∆ W$ is the work done and $∆ U$ is change in internal energy of the system.

	Process		Condition
(I)	Adiabatic	(A)	$∆ W = 0$
(II)	Isothermal	(B)	$∆ Q = 0$
(III)	Isochoric	(C)	$∆ U \neq 0, ∆ W \neq 0, ∆ Q \neq 0$
(IV)	Isobaric	(D)	$∆ U = 0$

EASY

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

Which of the following does not follow first law of thermodynamics?

(w =

work

; q =

heat

; ΔU =

change in internal energy

)

MEDIUM

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

An air bubble rises from the bottom of a water tank of height $5 m$ . If the initial volume of the bubble is $3 {mm}^{3}$ . What will be its volume as it reaches the surface. Assume that its temperature does not change.

[ $g = 9.8 m s^{- 2}, 1 atm = 10^{5} Pa$ , density of water $= 1 gm {cc}^{- 1}$ ]

MEDIUM

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

The internal energy of the air, in a room of volume

V

, at temperature

T

and with outside pressure

P

increasing linearly with time, varies as

EASY

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

A diatomic gas with rigid molecules does

10 J

of work when expanded at constant pressure. What would be the heat energy absorbed by the gas, in this process?

EASY

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

Five moles of an ideal monoatomic gas with an initial temperature of

150 ° C

expand and in the process absorb

1500 J

of heat and does

2500 J

of work. The final temperature of the gas in

° C

is (ideal gas constant,

R = 8.314 J K^{- 1} {mol}^{- 1}

EASY

Physics>Heat and Thermodynamics>Thermodynamics>First Law of Thermodynamics

Figure below shows two paths that may be taken by a gas to go from a state A to a state C.
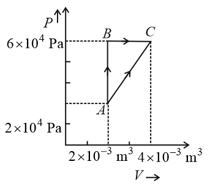
Question Image

In process AB,

400 J

of heat is added to the system and in process BC,

100 J

of heat is added to the system. The heat absorbed by the system in the process AC will be:

A monatomic ideal gas sample is given heat Q. One half of this heat is used as work done by the gas and rest is used for increasing its internal energy. The equation of process in terms of volume and temperature is

Important Questions on Thermodynamics

Learn and Prepare for any exam you want !

A monatomic ideal gas sample is given heat $Q$ . One half of this heat is used as work done by the gas and rest is used for increasing its internal energy. The equation of process in terms of volume and temperature is