Two harmonic waves are represented in SI units by y 1 ( x , t ) = 0 . 2 sin ( x - 3 . 0 t ) and y 2 ( x , t ) = 0 . 2 sin ( x - 3 . 0 t + ϕ ) (a) Write the expression for the sum y = y 1 + y 2 for ϕ = π 2 rad . (b) Suppose the phase difference ϕ between the waves is unknown and the amplitude of their sum is 0 . 32 m , what is ϕ ?

Given that, two harmonic waves are represented by, y 1 ( x , t ) = 0 . 2 sin ( x - 3 . 0 t ) and y 2 ( x , t ) = 0 . 2 sin ( x - 3 . 0 t + ϕ ) (a) Given that, the phase difference between two harmonic waves is, ϕ = π 2 . y = y 1 + y 2 = 0 . 2 sin x - 3 . 0 t + 0 . 2 sin x - 3 . 0 t + π 2 y = A eff sin x - 3 . 0 t + θ θ = phase difference between the resultant amplitude and amplitude of an individual wave. Effective amplitude of superposed waves is, A eff = A 2 + A 2 + 2 A A cos ϕ A eff = 2 A cos ϕ 2 ∵ A 1 = A 2 = A As both waves are perpendicular to each other, effective amplitude of the resultant wave, A eff = 2 A cos π 2 2 = 2 A cos π 4 = 2 A 1 2 A eff = 2 A = 2 0 . 2 = 1 . 41 0 . 2 = 0 . 28 m θ = ϕ 2 = π 2 2 = π 4 Hence, the resultant wave equation is, y = 0 . 28 sin x - 3 . 0 t + π 4 (b) If the phase difference is unknown, then A eff = 0 . 32 m . Effective amplitude of superposed waves is, A eff = 2 A cos ϕ 2 ⇒ 0 . 32 = 2 0 . 2 cos ϕ 2 cos ϕ 2 = 0 . 8 ⇒ ϕ 2 = cos - 1 0 . 8 ϕ 2 = 0 . 643 rad ⇒ ϕ ≅ 1 . 29 rad

Figure shows different standing wave patterns on a string of linear mass density 4 . 0 × 10 - 2 kg m - 1 under a tension of 100 N . The amplitude of antinodes is indicated in each figure. The length of the string is 2 . 0 m . (i) Obtain the frequencies of the modes shown in figures ( a ) and ( b ) . (ii) Write down the transverse displacement y as a function of x and t for each mode. (Take the initial configuration of the wire in each mode to be as shown by the dark lines in the figure).

Given that, linear density of string is, μ = 4 . 0 × 10 - 2 kg m - 1 , length of the string is, L = 2 m . Tension developed in the string, T = 100 N . (i) In figure (a), string vibrates with one loop, i.e., string vibrates with fundamental frequency. Hence, the fundamental frequency of the string is, n a = 1 2 L T μ = 1 2 2 100 4 × 10 - 2 = 1 4 10 0 . 2 = 12 . 5 Hz In figure (b), string vibrates with three loops, hence, the frequency of vibrations in string is, n b = 3 n a = 3 12 . 5 = 37 . 5 Hz . (ii) From figures (a) and (b), amplitudes of waves are, A a = 0 . 1 m an d A b = 0 . 04 m Angular frequencies of both the waves are, ω a = 2 π n a = 2 π 12 . 5 = 25 π rad s - 1 ω b = 2 π n b = 2 π 37 . 5 = 75 π rad s - 1 Speed of transverse wave is, v = T μ = 100 4 × 10 - 2 = 10 0 . 2 = 50 m s - 1 Propagation constants of both waves are, v = ω k ⇒ k = ω v k a = ω a v = 25 π 50 = π 2 rad m - 1 k b = ω b v = 75 π 50 = 3 π 2 rad m - 1 Equations of the waves (standing waves in stretching strings) in figures (a) and (b) are, y a = A a sin k a x sin ω a t and y b = A b sin k b x sin ω b t y a = 0 . 1 sin π 2 x sin 25 π t m and y b = 0 . 04 sin 3 π 2 x sin 75 π t m .

EASY

JEE Advanced

IMPORTANT

Earn 100

A string vibrates in $4$ segments to a frequency of $400 Hz$ .
(a) What is its fundamental frequency?
(b) What frequency will cause it to vibrate into $7$ segments?

Important Questions on Superposition of Waves

EASY

JEE Advanced

IMPORTANT

Understanding Physics JEE Main & Advanced WAVES AND THERMODYNAMICS>Chapter 2 - Superposition of Waves>Exercises>Q 15

A sonometer wire has a total length of

1 m

between the fixed ends. Where should the two bridges be placed below the wire so that the three segments of the wire have their fundamental frequencies in the ratio

1 : 2 : 3

EASY

JEE Advanced

IMPORTANT

Understanding Physics JEE Main & Advanced WAVES AND THERMODYNAMICS>Chapter 2 - Superposition of Waves>Exercises>Q 16

A guitar string is

90 cm

long and has a fundamental frequency of

124 Hz

. Where should it be pressed to produce a fundamental frequency of

186 Hz

HARD

JEE Advanced

IMPORTANT

Understanding Physics JEE Main & Advanced WAVES AND THERMODYNAMICS>Chapter 2 - Superposition of Waves>Exercises>Q 17

Adjacent antinodes of a standing wave on a string are

15.0 cm

apart. A particle at an antinode oscillates in simple harmonic motion with amplitude

0.850 cm

and period

0.0750 s

. The string lies along the

+ x

-axis and is fixed at

x = 0

.
(a) Find the displacement of a point on the string as a function of position and time.
(b) Find the speed of propagation of a transverse wave in the string.
(c) Find the amplitude at a point

3.0 cm

to the right of an antinode.

MEDIUM

JEE Advanced

IMPORTANT

Understanding Physics JEE Main & Advanced WAVES AND THERMODYNAMICS>Chapter 2 - Superposition of Waves>Exercises>Q 18

1.50 m

long rope is stretched between two supports with a tension that makes the speed of transverse waves

48.0 m s^{- 1}

. What are the wavelength and frequency of
(a) the fundamental?
(b) the second overtone?
(c) the fourth harmonic?

HARD

JEE Advanced

IMPORTANT

Understanding Physics JEE Main & Advanced WAVES AND THERMODYNAMICS>Chapter 2 - Superposition of Waves>Exercises>Q 19

A thin taut string tied at both ends and oscillating in its third harmonic has its shape described by the equation

y (x, t) = (5.60 cm) \sin [(0.0340 rad {cm}^{- 1}) x] \sin [(50.0 rad s^{- 1}) t]

, where the origin is at the left end of the string, the

x

-axis is along the string and the

y

-axis is perpendicular to the string.
(a) Draw a sketch that shows the standing wave pattern.
(b) Find the amplitude of the two travelling waves that make up this standing wave.
(c) What is the length of the string?
(d) Find the wavelength, frequency, period and speed of the travelling wave.
(e) Find the maximum transverse speed of a point on the string.
(f) What would be the equation

y (x, t)

for this string if it were vibrating in its eighth harmonic?

HARD

JEE Advanced

IMPORTANT

Understanding Physics JEE Main & Advanced WAVES AND THERMODYNAMICS>Chapter 2 - Superposition of Waves>Exercises>Q 20

A wire with mass

40.0 g

is stretched so that its ends are tied down at points

80.0 cm

apart. The wire vibrates in its fundamental mode with frequency

60.0 Hz

and with an amplitude at the antinodes of

0.300 cm

.
(a) What is the speed of propagation of transverse wave in the wire?
(b) Compute the tension in the wire.
(c) Find the maximum transverse velocity and acceleration of particles in the wire.

MEDIUM

JEE Advanced

IMPORTANT

Understanding Physics JEE Main & Advanced WAVES AND THERMODYNAMICS>Chapter 2 - Superposition of Waves>Exercises>Q 21

Two harmonic waves are represented in SI units by

y_{1} (x, t) = 0.2 \sin (x - 3.0 t)

and

y_{2} (x, t) = 0.2 \sin (x - 3.0 t + ϕ)

(a) Write the expression for the sum

y = y_{1} + y_{2}

for

ϕ = \frac{π}{2} rad

.
(b) Suppose the phase difference

ϕ

between the waves is unknown and the amplitude of their sum is

0.32 m

, what is

ϕ

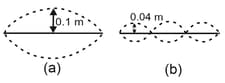
EASY

JEE Advanced

IMPORTANT

Understanding Physics JEE Main & Advanced WAVES AND THERMODYNAMICS>Chapter 2 - Superposition of Waves>Exercises>Q 22

Figure shows different standing wave patterns on a string of linear mass density $4.0 \times 10^{- 2} kg m^{- 1}$ under a tension of $100 N$ . The amplitude of antinodes is indicated in each figure. The length of the string is $2.0 m$ .

Question Image

(i) Obtain the frequencies of the modes shown in figures $(a)$ and $(b)$ .
(ii) Write down the transverse displacement $y$ as a function of $x$ and $t$ for each mode. (Take the initial configuration of the wire in each mode to be as shown by the dark lines in the figure).

A string vibrates in 4 segments to a frequency of 400 Hz. (a) What is its fundamental frequency? (b) What frequency will cause it to vibrate into 7 segments?

Important Questions on Superposition of Waves

Learn and Prepare for any exam you want !

A string vibrates in $4$ segments to a frequency of $400 Hz$ .
(a) What is its fundamental frequency?
(b) What frequency will cause it to vibrate into $7$ segments?