A weightless piston divides a thermally insulated cylinder into two parts of volumes and of an ideal gas at pressure atmosphere are confined to the part with volume . The remainder of the cylinder is evacuated. The piston is now released and the gas expands to fill the entire space of the cylinder. The piston is then pressed back to the initial position. Find the increase of internal energy in the process and final temperature of the gas. The ratio of the specific heats of the gas,

Important Questions on Thermodynamics
In given figure, an adiabatic cylindrical tube of volume is divided in two equal parts by a frictionless adiabatic separator. An ideal gas in left side of a tube having pressure and temperature , where as on the right side having pressure and temperature is the same for both the gases. The separator is slid slowly and is released at a position where it can stay in equilibrium. Find (a) the final volumes of the two parts, (b) the heat given to the gas in the left part and (c) the final common pressure of the gases.

A cylindrical tube with adiabatic walls having volume contains an ideal monoatomic gas as shown in figure. The tube is divided into two equal parts by a fixed super conducting wall. Initially, the pressure and the temperature are on the left and on the right. When system is left for sufficient amount of time the temperature on both sides becomes equal (a) Find work done by the gas on the right part? (b) Find the final pressures on the two sides. (c) Find the final equilibrium temperature. (d) How much heat has flown from the gas on the right to the gas on the left?
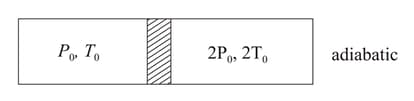
An ideal gas having initial pressure and volume .
(a) The gas is taken isothermally to a pressure and then adiabatically to a pressure . Find the final volume.
(b) The gas is brought back to its initial state. It is adiabatically taken to a pressure and then isothermally to a pressure . Find the final volume.
A Carnot engine cycle is shown in the figure (2). The cycle runs between temperatures Minimum and maximum volume at state and state are and , respectively. The cycle uses one mole of an ideal gas with . Here and are the specific heats at constant pressure and volume respectively. You must express all answers in terms of the given parameters and universal gas constant .
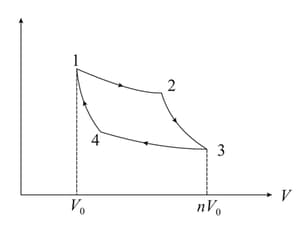
(a) Find for all the states
(b) Calculate the work done by the engine in each process
(c) Calculate , the heat absorbed in the cycle.
Cloud formation condition
Consider a simplified model of cloud formation. Hot air in contact with the earth’s surface contains water vapour. This air rises connectively till the water vapour content reaches its saturation pressure. When this happens, the water vapour starts condensing and droplets are formed. We shall estimate the height at which this happens. We assume that the atmosphere consists of the diatomic gases oxygen and nitrogen in the mass proportion respectively. We further assume that the atmosphere is an ideal gas, the acceleration due to gravity is constant and air processes are adiabatic. Under these assumptions one can show that the pressure is given by
Here and is the pressure and temperature respectively at sea level is the lapse rate (magnitude of the change in temperature with height above the earth’s surface, i.e. ).
(a) Obtain an expression for the lapse rate in terms of and . Here is the ratio of specific heat at constant pressure to specific heat at constant volume; , the gas constant; and , the relevant molar mass.
(b) Estimate the change in temperature when we ascend a height of one kilometre ?
(c) Show that pressure will depend on height as given by Eq. (1). Find an explicit expression for exponent in terms of .
(d) According to this model what is the height to which the atmosphere extends? Take and
