Consider two particle mass $m$ and $16m$ separated by a distance $d$.

Deduce that a point $P$, a distance $\frac{d}{5}$ from the particle with mass $m$, the gravitational strength is zero.

Consider two particle mass $m$ and $16m$ separated by a distance $d$. There is a point $P$, a distance $\frac{d}{5}$ from the particle with mass $m$.

Determine the value of the gravitational potential at $P$.

The diagram shows the variation with distance from the centre of the planet of the gravitational potential due to the planet and its Moon. The planet's centre is at $r=0$ and the centre of the Moon is at $r=1$. The units of separation are arbitrary. At the point where $r=0.75$ the gravitational field is zero.

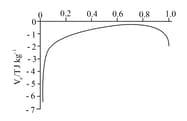
With what speed must a probe be launched from the surface of the planet in order to arrive on the surface of the Moon $?$

The diagram shows a planet orbiting the Sun counter-clockwise, at two positions-$A$ and $B$. Also shown is the gravitational force acting on the planet at each position. By decomposing the force into components normal and tangential to the path (dotted lines), explain why it is only the tangential component that does work. Hence, explain why the planet will accelerate from $A$ to $P$ but will slow down from $P$ to $B$.

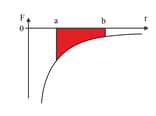
The diagram shows the variation of the gravitational force with distance between two masses. What does the shaded area represent $?$