Energy of 1000 J is spent to increase the angular speed of a wheel from 20 rad / s to 30 rad / s . Calculate the moment of inertia of the wheel.

Given Initial angular speed, ω 1 = 20 rad / s Final angular speed, ω 2 = 30 rad / s It is a case of conservation of energy in rotational kinematics. The change in kinetic energy is equal to the work done or the energy spent. Expression for change in rotational kinetic energy is 1 2 I ω 2 2 - ω 1 2 where I is moment of inertia. 1000 = 1 2 × I × 30 2 - 20 2 I = 4 kg m 2

HARD

Earn 100

Derive an expression for kinetic energy of a rotating body with uniform angular velocity.

Important Questions on Systems of Particles and Rotational Motion

MEDIUM

Physics>Mechanics>Systems of Particles and Rotational Motion>Energy in Rotation

Kinetic energy of rotation of a flywheel of radius

2 m,

mass

8 kg

and angular speed

4 rad s^{- 1}

about an axis perpendicular to its plane and passing through its center is

MEDIUM

Physics>Mechanics>Systems of Particles and Rotational Motion>Energy in Rotation

A stationary horizontal disc is free to rotate about its axis. When a torque is applied on it, its kinetic energy as a function of

θ

, where

θ

is the angle by which it has rotated, is given as

k θ^{2}

. If its moment of inertia is

I

then the angular acceleration of the disc is:

MEDIUM

Physics>Mechanics>Systems of Particles and Rotational Motion>Energy in Rotation

A wheel is rotating freely with an angular speed

ω

on a shaft. The moment of inertia of the wheel is

I

and the moment of inertia of the shaft is negligible. Another wheel of moment of inertia

3 I

initially at rest is suddenly coupled to the same shaft. The resultant fractional loss in the kinetic energy of the system is:

EASY

Physics>Mechanics>Systems of Particles and Rotational Motion>Energy in Rotation

Two bodies have their moments of inertia

I

and

2 I

respectively about their axes of rotation. If their kinetic energies of rotation are equal, their angular momenta will be in the ratio__

MEDIUM

Physics>Mechanics>Systems of Particles and Rotational Motion>Energy in Rotation

A rod of length $50 c m$ is pivoted at one end. It is raised such that it makes an angle of $30^{o}$ from the horizontal as shown and released from rest. Its angular speed when it passes through the horizontal (in $r a d s^{- 1}$ ) will be $(g = 10 m s^{- 2})$

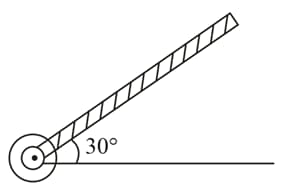
Question Image

HARD

Physics>Mechanics>Systems of Particles and Rotational Motion>Energy in Rotation

A wheel of mass

10 kg

and radius

0.8 m

is rolling on a road with an angular speed

20 {rads}^{- 1}

without sliding. The moment of inertia of the wheel about the axis of rotation is

1.2 {kgm}^{2},

then the percentage of rotational kinetic energy in the total kinetic energy of the wheel is ____________(approximately)

MEDIUM

Physics>Mechanics>Systems of Particles and Rotational Motion>Energy in Rotation

Moment of inertia of a body about a given axis is

1.5 k g m^{2} .

Initially the body is at rest. In order to produce a rotational kinetic energy of

1200 J,

the angular acceleration of

20 r a d / s^{2}

must be applied about the axis for a duration of:

MEDIUM

Physics>Mechanics>Systems of Particles and Rotational Motion>Energy in Rotation

The moment of inertia of a ring about an axis passing through the centre and perpendicular to its plane is

I

. It is rotating with angular velocity

ω.

Another identical ring is gently placed on it so that their centres coincide. If both the rings are rotating about the same axis then loss in kinetic energy is

EASY

Physics>Mechanics>Systems of Particles and Rotational Motion>Energy in Rotation

A solid sphere of mass,

m

and radius,

R

is rotating about its diameter. A solid cylinder of the same mass and same radius is also rotating about its geometrical axis with an angular speed twice that of the sphere. The ratio of their kinetic energies of rotation

(\frac{E_{sphere}}{E_{cylinder}})

will be

EASY

Physics>Mechanics>Systems of Particles and Rotational Motion>Energy in Rotation

A solid sphere of mass

2 kg

is rolling on a frictionless horizontal surface with velocity

6 m s^{- 1}

. It collides on the free end of an ideal spring whose other end is fixed. The maximum compression produced in the spring will be (Force constant of the spring =

36 N m^{- 1}

EASY

Physics>Mechanics>Systems of Particles and Rotational Motion>Energy in Rotation

The ring of radius

1 m

and mass

15 kg

is rotating about its diameter with angular velocity of

25 rad / \sec

. Its kinetic energy is

EASY

Physics>Mechanics>Systems of Particles and Rotational Motion>Energy in Rotation

A solid sphere is in rolling motion. In rolling motion a body possesses translational kinetic energy

(K_{t})

as well as rotational kinetic energy

(K_{r})

simultaneously. The ratio

K_{t} : (K_{t} + K_{r})

for the sphere is

MEDIUM

Physics>Mechanics>Systems of Particles and Rotational Motion>Energy in Rotation

A uniform circular disc of mass

400 g

and radius

4.0 cm

is rotated about one of its diameter at an angular speed of

10 rad s^{- 1}

. The kinetic energy of the disc is

MEDIUM

Physics>Mechanics>Systems of Particles and Rotational Motion>Energy in Rotation

A wheel is at rest in horizontal position. Its

M . I .

about vertical axis passing through its centre is

I

. A constant torque

τ

acts on it for

t

second. The change in rotational kinetic energy is

EASY

Physics>Mechanics>Systems of Particles and Rotational Motion>Energy in Rotation

Energy of

1000 J

is spent to increase the angular speed of a wheel from

20 rad / s

30 rad / s

. Calculate the moment of inertia of the wheel.

EASY

Physics>Mechanics>Systems of Particles and Rotational Motion>Energy in Rotation

Point masses $m_{1}$ and $m_{2}$ are placed at the opposite ends of rigid rod of length $L$ , and negligible mass. The rod is to be set rotating about an axis perpendicular to it. The position of point $L$ on this rod through which the axis should pass so that the work required to set the rod rotating with angular velocity $ω_{0}$ is minimum, is given by:
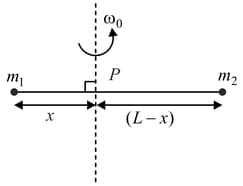
Question Image

EASY

Physics>Mechanics>Systems of Particles and Rotational Motion>Energy in Rotation

A uniform circular disc of radius

50 cm

at rest is free to rotate about an axis which is perpendicular to its plane and passes through its centre. It is subjected to a torque which produces a constant angular acceleration of

2.0 r a d s^{- 2}

. Its net acceleration in

m s^{- 2}

at the end of

2.0 s

is approximately:

EASY

Physics>Mechanics>Systems of Particles and Rotational Motion>Energy in Rotation

Two discs of same moment of inertia

(I)

are rotating in same sense about their regular axis passing through centre and perpendicular to the plane of disc with angular velocities

ω_{1}

and

ω_{2}

. They are brought into contact face to face coinciding the axis of rotation. The expression for loss in energy during this process is

EASY

Physics>Mechanics>Systems of Particles and Rotational Motion>Energy in Rotation

Three objects,

A

: (a solid sphere),

B

: (a thin circular disk) and

C

: (a circular ring), each have the same mass

M

and radius

R

. They all spin with the same angular speed

ω

about their own symmetric axes. The amounts of work

(W)

required to bring them to rest, would satisfy the relation

EASY

Physics>Mechanics>Systems of Particles and Rotational Motion>Energy in Rotation

A disc of radius

2 m

and mass

100 k g

rolls on a horizontal floor. Its centre of mass has speed of

20 c m s^{- 1} .

How much work is needed to stop it?

Derive an expression for kinetic energy of a rotating body with uniform angular velocity.

Important Questions on Systems of Particles and Rotational Motion

Learn and Prepare for any exam you want !