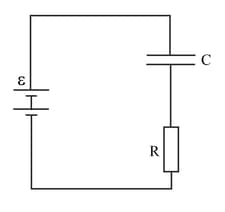
Derive the expression of current as a function of time during the charging of the capacitor in the $RC$ circuit.

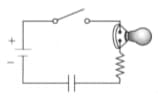
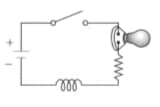
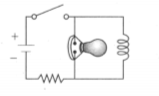
Consider the four circuits shown in the figure, each consisting of a battery, a switch, a light bulb, a resistor and either a capacitor or an inductor. Assume the capacitor has a large capacitance and the inductor has a large inductance but no resistance. The light bulb has high efficiency and glows whenever electric current passes through it.

(i) Describe what the light bulb does in each of circuits (a) through (d) after the switch is closed.

(ii) Describe what the light bulb does in each of circuits (a) through (d) when the switch has been closed for a long time interval and when the switch is opened.

In the circuit shows a capacitor that is initially uncharged is being charged by a battery.

Which of the following is a correct graph of the variation of the potential difference $\mathrm{V}$ across the plates with charge $\mathrm{q}$ on one of the capacitor plates?

A circuit consists of a source of a constant emf $\xi$ and a resistance $R$ and a capacitor with capacitance $C$ connected in series. The internal resistance of the source is negligible. At a moment $t=0$, the capacitance of the capacitor is abruptly decreased $\eta$-fold. Find the current flowing through the circuit as a function of time $t$.

The charge on each of the capacitors, $0.20 \mathrm{ms}$ after the switch $S$ is closed in figure is

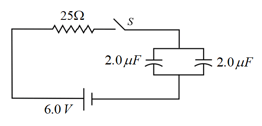
The capacitor is discharging through a resistor of resistance $2.5 \mathrm{M\Omega }$.  What is the current through the resistor when the charge on one of the plates has been reduced to $8.0 \mathrm{nC}$? Here capacitor attached with $6 \mathrm{V}$ Ideal voltmeter. And initial charge in capacitor Is $12 \mathrm{nC}$.

Find how the voltage across the capacitor $C$ varies with time $t$ after the shorting of the switch $S_w$ at the moment $t=0$.

The graph shows the variation with time t of the current I for a discharging capacitor.

What is the time constant of this system?(in sec)

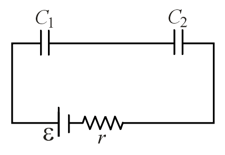
The switch is closed at $t=0$ when the capacitors are uncharged. Find the charge on the capacitor $C_1$ as a function of time $t$.

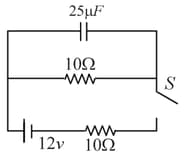
A capacitor of $4 \mathrm{\mu F}$ is connected as shown in the circuit. The internal resistance of the battery is $0.5 \mathrm{\Omega }$. The amount of charge on the capacitor plates will be

Two capacitors of equal capacitance $\left(C_1=C_2\right)$ are as shown in the figure. Initially, while the switch is open (as shown) one of the capacitors is uncharged and the other carries charge $Q_0$. The energy stored in the charged capacitor is $U_0$. Sometime after the switch is closed, the capacitors $C_1$ and $C_2$ carry charges $Q_1$ and $Q_2$, respectively. The energies stored in the capacitors are $U_1$ and $U_2$ respectively. Which of the following expression is correct?

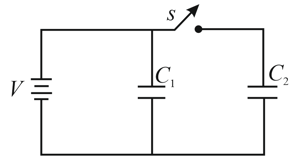
The magnitude of the charge in steady-state on either of the plates of condenser $C$ in the adjoining circuit is 

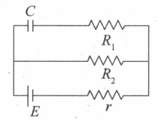
What will the initial current be in the below the instant the switch is closed, and what will it be eventually a long time after the switch is closed? (answer in order)

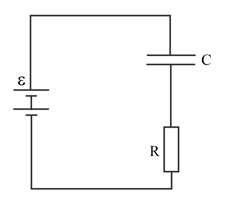
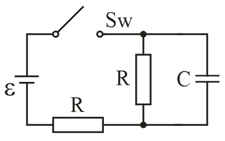
The switch $S$ is kept closed for a long time and is then opened at $t=0$. Find the current in the middle $\left(10 \mathrm{\Omega }\right)$ resistor at $t=1.0 \mathrm{ms}$.