Determine the radius of an incircle by drawing an inscribing circle of a regular hexagon of side $8 \mathrm{cm}$.

Use ruler and compass only for answering the question.

Draw a circle of radius $4 \mathrm{cm}$. Mark the centre as $\mathrm{O}$. Mark a point $\mathrm{P}$ outside the circle at a distance of $7 \mathrm{cm}$ from the centre. Construct two tangents to the circle from the external point $\mathrm{P}$.

Measure and write down the length of any one tangent.

Which of the following steps is INCORRECT to construct a circle of the radius $2 \mathrm{cm}$ with centre $O$ and then drawing two tangents to the circle from $P$ where $P$ is a point outside the circle such that $OP=4.5 \mathrm{cm}.$
Steps of construction

Step $\mathrm{I}$ : Draw a circle with $O$ as center and radius $2 \mathrm{cm}.$

Step $\mathrm{I}\mathrm{I}$: Mark a point $P$ outside the circle such that $OP=2.25 \mathrm{cm},$

Step $\mathrm{I}\mathrm{I}\mathrm{I}$ : Join $OP=4.5 \mathrm{cm}$ and bisect it at $M$.

Step $\mathrm{I}\mathrm{V}$ : Draw a circle with $M$ as centre and radius equal to $MP$ to intersect the given circle at the points $T$ and $T$’.

Step $\mathrm{V}$ : Joint $PT$ and $PT\text{'}$. Then, $PT$ and $PT\text{'}$ are the required tangents.

Given, a $△$$ABC$ with $BC=6.5 \mathrm{cm}, AB=5.5 \mathrm{cm}$ and $AC=5 \mathrm{cm}$ with incircle in the figure below:

The distance from centre of the circle to vertex $B$ of $△ABC$ is

Arrange the following steps of construction while constructing a pair of tangents to a circle, which are inclined to each other at an angle of $60°$ to a circle of radius $3 \mathrm{cm}$
Steps of Construction

Step $\mathrm{I}$ : Draw any diameter $AOB$ of this circle.

Step $\mathrm{I}\mathrm{l}$: Draw $AM\perp AB$ and $CN\perp OC$. Let $AM$ and $CN$ intersect each other at $P$. Then $PA$ and $PC$ are the desired tangents to the given circle, inclined at an angle of $60°.$

Step $\mathrm{I}\mathrm{l}\mathrm{l}$: Draw a circle with $O$ as Centre and radius $3 \mathrm{cm}$.

Step $\mathrm{I}\mathrm{V}$ : Construct $\angle AOC=120°$ such that radius $OC$ meets the circle at $C.$

The construction steps to draw a pair of tangents to the circle of radius $6 \mathrm{cm}$ from a point $10 \mathrm{cm}$ away from its centre, are given below but not in correct order, select the correct order from the options below.

A pair of tangents to the given circle can be constructed as follows.

Step 1: Taking M as centre and MO as radius, draw a circle.
Step 2: Let this circle intersect the previous circle at point Q and R.
Step 3: Taking any point O of the given plane as centre, draw a circle of $6 \mathrm{cm}$ radius. Locate a point P, $10 \mathrm{cm}$ away from O. Join OP.
Step 4: Bisect OP. Let M be the mid-point of PO.
Step 5: Join PQ and PR. PQ and PR are the required tangents.

Arrange the following steps of construction while constructing a pair of tangents to a circle of radius $3 \mathrm{cm}$ from a point $10 \mathrm{cm}$ away from the center of the circle.
Steps of Construction

Step $\mathrm{I}$ : Bisect the line segment $OP$ and let the point of bisection be $M.$

Step $\mathrm{I}\mathrm{l}$: Taking $M$ as centre and $0M$ as radius, draw a circle. Let it intersect the given circle at the point $Q$ and $R.$

Step $\mathrm{I}\mathrm{I}\mathrm{I}$ : Draw a circle of radius $3 \mathrm{cm}$

Step $\mathrm{I}\mathrm{V}$ : Join $PQ$ and $PR.$

Step $\mathrm{V}$: Take an external point $P$ which is $10$$\mathrm{cm}$ away from its centre. Join $OP.$

Draw a circle of radius $6 \mathrm{cm}$. From a point $8 \mathrm{cm}$ away from its centre, construct the pair of tangents to the circle. Below given are the steps for construction. Arrange them in order 

A. Taking $\mathrm{M}$ as centre and $\mathrm{MO}$ as radius draw a circle 

B. Join $\mathrm{PQ}$ and $\mathrm{PR}.$

C. Taking any point $\mathrm{O}$ as centre draw a  circle of $6 \mathrm{cm}$ radius-locate a point P, $8 \mathrm{cm}$ away from $\mathrm{O}.$

D. Bisect $\mathrm{PO},$ and let $\mathrm{M}$ be the midpoint of $\mathrm{PO}.$

E. The circle interests the previous circle at points $\mathrm{Q}$ and $\mathrm{R}.$

Which of the following steps is INCORRECT to construct a tangent to the circle of radius $5 \mathrm{cm}$ at the point $P$ on it without using the center of the circle.
Steps of Construction

Step $\mathrm{I}$: Draw a circle of radius $5 \mathrm{cm}$.

Step $\mathrm{I}\mathrm{l}$: Mark a point $P$ on it.

Step $\mathrm{I}\mathrm{I}\mathrm{I}$: Draw any chord $PQ.$

Step $\mathrm{I}\mathrm{V}$: Take a point $R$ in the minor arc $QP.$

Step $\mathrm{V}$: Join $PR$ and $RQ.$

Step $\mathrm{V}\mathrm{I}$: Make $\angle QPT=$ $\angle PRQ$.

Step $\mathrm{V}\mathrm{I}\mathrm{I}$: Produce $TP$ to $T\text{'}$. Then, $PT$ is the required tangent at $P$.

Given, a $△$$\mathrm{ABC}$ with $\mathrm{BC}=6.5 \mathrm{cm}, \mathrm{AB}=5.5 \mathrm{cm}$ and $\mathrm{AC}=5 \mathrm{cm}$ with incircle in the figure below:

The radius of incircle is

Which of the following steps of construction is INCORRECT while drawing a tangent to a circle of radius $5 \mathrm{cm}$ and making an angle of $30°$ with a line passing through the center.
Steps of Construction

Step $\mathrm{I}$ : Draw a circle with Centre $O$ and radius $2.5 \mathrm{cm}$.

Step $\mathrm{I}\mathrm{l}$: Draw a radius $OA$ of this circle and produce it to $B$.

Step $\mathrm{I}\mathrm{I}\mathrm{I}$ : Construct an angle $\angle AOP$ equal to the complement of $30°$ i.e. equal to $150°.$

Step $\mathrm{I}\mathrm{V}$: Draw a perpendicular to $OP$ at $P$ which intersects $OA$ produced at $Q.$

Clearly, $PQ$ is the desired tangent such that $\angle OQP=30°$.

Given, a $△$$\mathrm{ABC}$ with $\mathrm{BC}=6.5 \mathrm{cm}, \mathrm{AB}=5.5 \mathrm{cm}$ and $\mathrm{AC}=5 \mathrm{cm}$ with incircle in the figure below.

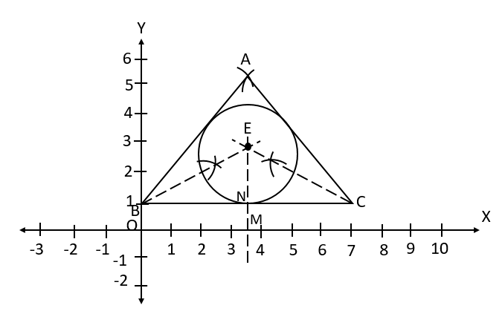
The distance from the centre $E=\left(3.5 \mathrm{cm}, 2.5 \mathrm{cm}\right)$ of the circle to vertex $\mathrm{C}$ of $△\mathrm{ABC}$ is _____ units rounded off to two decimal places.

Let $ABC$ be a right triangle in which $AB=3 \mathrm{cm},BC=4 \mathrm{cm}$ and $\angle B=90°. BD$ is the perpendicular from $B$ on $AC$. The circle through $B,C,D$ is drawn. Given below are the steps of constructions of a pair of tangents from $A$ to this circle. Which of the following steps is INCORRECT?
Steps of Construction

Step $\mathrm{I}$: Draw $∆ABC$ and perpendicular $BD$ from $B$ on $AC$.

Step $\mathrm{I}\mathrm{l}$: Draw a circle with $BC$ as a diameter. This circle will pass through $D.$

Step $\mathrm{I}\mathrm{l}\mathrm{l}$: Let $O$ be the mid-point of $BC$. Join $AO.$

Step $\mathrm{I}\mathrm{V}$: Draw a circle with $AO$ as diameter. This circle cuts the circle drawn in step $\mathrm{I}\mathrm{l}$ at $B$ and $P. AO, AP$ and $AB$ are desired tangents drawn from $A$ to the circle passing through $B,C$ and $D.$

Arrange the steps of construction while constructing a pair of tangents to a circle of radius $5 \mathrm{cm}$ from a point $12 \mathrm{cm}$ away from its Centre.
Steps of Construction

Step $\mathrm{I}$: Join $OA$ and bisect it. Let $P$ is the mid-point of $OA$.

Step $\mathrm{I}\mathrm{l}$: Join $AB$ and $AC$. $AB$ and $AC$ are the required tangents. Length of tangents $=11 \mathrm{cm}.$

Step $\mathrm{I}\mathrm{l}\mathrm{l}$ : With $O$ as centre, draw a circle of radius $5 \mathrm{cm}.$

Step $\mathrm{I}\mathrm{V}$: Taking $P$ as centre and $PO$ as radius, draw a circle intersecting the given circle at the points $B$ and $C.$

Step $\mathrm{V}$: Take a point $A$ at a distance of $12 \mathrm{cm}$ from $O$.