Find the value of $\cos \left({\cos }^{-1}\left(\frac{4}{5}\right)+{\sin }^{-1}\left(\frac{4}{5}\right)\right)$.

1. Domain and range of trigonometric functions

$\begin{array}{|ccccccc|}\hline \text{ Trigonometric function }& \sin x& \cos x& \tan x& cosecx& \sec x& \cot x\\ \text{ Domain }& \mathrm{ℝ}& \mathrm{ℝ}& \mathrm{ℝ}\backslash \left\{(2n+1)\frac{\pi }{2},n\in \mathrm{\mathbb{Z}}\right\}& \mathrm{ℝ}\backslash \{n\pi ,n\in \mathrm{\mathbb{Z}}\}& \mathrm{ℝ}\backslash \left\{(2n+1)\frac{\pi }{2},n\in \mathrm{\mathbb{Z}}\right\}& \mathrm{ℝ}\backslash \{n\pi ,n\in \mathrm{\mathbb{Z}}\}\\ \text{ Range }& [-1,1]& [-1,1]& \mathrm{ℝ}& \mathrm{ℝ}\backslash (-1,1)& \mathrm{ℝ}\backslash (-1,1)& \mathrm{ℝ}\\ \hline\end{array}$

2. Amplitude and Period of a graph

(i) The amplitude is the maximum distance of the graph from the x -axis. Thus, the amplitude of a function is the height from the x -axis to its maximum or minimum. 

(ii) The period is the distance required for the function to complete one full cycle.

3. Graphs of trigonometric and inverse trigonometric function:

(i) Graphs of trigonometric function

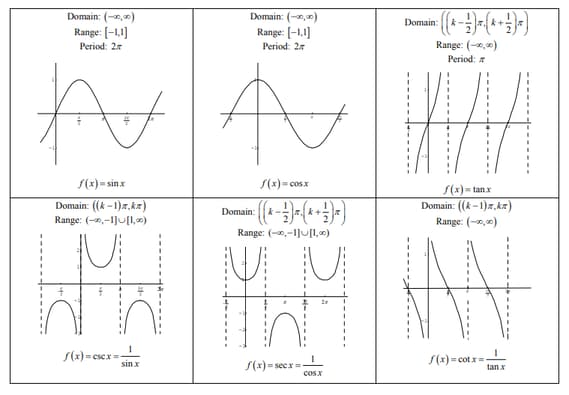
(ii) Graphs of inverse trigonometric functions:

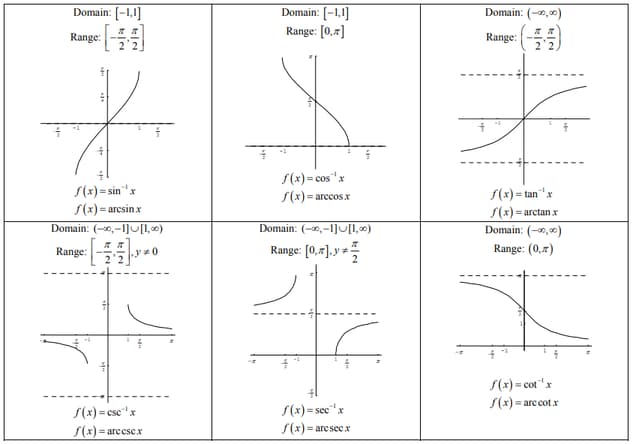
4. Domain and range of inverse trigonometric functions:

    $\begin{array}{|lll|}\hline \begin{array}{c}\text{ Inverse }\\ \text{ Function }\end{array}& \text{ Domain }& \begin{array}{c}\text{ Range of }\\ \text{ Principal }\\ \text{ value branch }\end{array}\\ {\sin }^{-1}& [-1,1]& \left[-\frac{\pi }{2},\frac{\pi }{2}\right]\\ {\cos }^{-1}& [-1,1]& [0,\pi ]\\ {\tan }^{-1}& \mathrm{ℝ}& \left(-\frac{\pi }{2},\frac{\pi }{2}\right)\\ {cosec}^{-1}& \mathrm{ℝ}\backslash (-1,1)& \left[-\frac{\pi }{2},\frac{\pi }{2}\right]\backslash \left\{0\right\}\\ {\sec }^{-1}& \mathrm{ℝ}\backslash (-1,1)& [0,\pi ]\backslash \left\{\frac{\pi }{2}\right\}\\ {\cot }^{-1}& \mathrm{ℝ}& (0,\pi )\\ \hline\end{array}$

5. Properties of Inverse Trigonometric functions of the form ${\mathbf{f}}^{\mathbf{-}\mathbf{1}}\mathbf{\left(}\mathbf{f}\mathbf{\right(}\mathbf{x}\mathbf{\left)}\mathbf{\right)}$:

(i) ${\sin }^{-1}\left(\sin x\right)=x,$ for all $x\in \left[-\frac{\pi }{2},\frac{\pi }{2}\right]$

(ii) ${\cos }^{-1}\left(\cos x\right)=x,$ for all $x\in \left[0,\pi \right]$

(iii) ${\tan }^{-1}\left(\tan x\right)=x,$ for all $x\in \left(-\frac{\pi }{2},\frac{\pi }{2}\right)$

(iv) ${\mathrm{cosec}}^{-1}\left(\mathrm{cosec}x\right)=x,$ for all $x\in \left[-\frac{\pi }{2},\frac{\pi }{2}\right]-\left\{0\right\}$

(v) ${\sec }^{-1}\left(\sec x\right)=x,$ for all $x\in \left[0,\pi \right]-\left\{\frac{\pi }{2}\right\}$

(vi) ${\cot }^{-1}\left(\cot x\right)=x,$ for all $x\in \left(0,\pi \right)$

6. Properties of Inverse Trigonometric functions of the form $\mathbf{f}\left({\mathbf{f}}^{\mathbf{-}\mathbf{1}}\mathbf{\left(}\mathbf{x}\mathbf{\right)}\right)$:

(i) $\sin \left({\sin }^{-1}x\right)=x,$ for all $x\in \left[-1,1\right]$

(ii) $\cos \left({\cos }^{-1}x\right)=x,$ for all $x\in \left[-1,1\right]$

(iii) $\tan \left({\tan }^{-1}x\right)=x,$ for all $x\in R$

(iv) $\mathrm{cosec}\left({\mathrm{cosec}}^{-1}x\right)=x,$ for all $x\in \left(-\infty ,\hspace{0.17em}-1\right]\cup \left[1,\hspace{0.17em}\infty \right)$

(v) $\sec \left({\sec }^{-1}x\right)=x,$ for all $x\in \left(-\infty ,\hspace{0.17em}-1\right]\cup \left[1,\hspace{0.17em}\infty \right)$

(vi) $\cot \left({\cot }^{-1}x\right)=x,$ for all $x\in R$

7. Property-III

(i) ${\sin }^{-1}\left(\sin x\right)=\left\{\begin{array}{c}-\pi -x, \text{if} x\in \left[-\frac{3\pi }{2},-\frac{\pi }{2}\right]\\ x, \text{if} x\in \left[-\frac{\pi }{2},\frac{\pi }{2}\right]\\ \pi -x, \text{if} x\in \left[\frac{\pi }{2},\frac{3\pi }{2}\right]\end{array}\right.$

(ii) ${\cos }^{-1}\left(\cos x\right)=\left\{\begin{array}{c}-x, \text{if} x\in \left[-\pi ,0\right]\\ x, \text{if} x\in \left[0,\pi \right]\\ 2\pi -x, \text{if} x\in \left[\pi ,2\pi \right]\\ -2\pi +x, \text{if} x\in \left[2\pi ,3\pi \right]\end{array}\right.$

(iii) $\mathrm{t}\mathrm{a}{\mathrm{n}}^{-1}\left(\mathrm{t}\mathrm{a}\mathrm{nx}\right)=\left\{\begin{array}{ccc}\pi -x,& \text{if} x\in \left(\frac{-3\pi }{2},\frac{-\pi }{2}\right)& \\ x,& \text{if} x\in \left(\frac{-\pi }{2},\frac{\pi }{2}\right)& \\ x-\pi ,& \text{if} x\in \left(\frac{\pi }{2},\frac{3\pi }{2}\right)& \\ x-2\pi ,& \text{if} x\in \left(\frac{3\pi }{2},\frac{5\pi }{2}\right)& \end{array}\right.$

8. Reflection Identities:

(i) ${\sin }^{-1}\left(-x\right)=-{\sin }^{-1}x,$ for all $x\in \left[-1,1\right]$

(ii) ${\cos }^{-1}\left(-x\right)=\pi -{\cos }^{-1}x,$ for all $x\in \left[-1,1\right]$

(iii) ${\tan }^{-1}\left(-x\right)=-{\tan }^{-1}x,$ for all $x\in R$

(iv) ${\mathrm{cosec}}^{-1}\left(-x\right)=-{\mathrm{cosec}}^{-1}x,$ for all $x\in \left(-\infty ,-1\right]\cup \left[1,\infty \right)$

(v) ${\sec }^{-1}\left(-x\right)=\pi -{\sec }^{-1}x,$ for all $x\in \left(-\infty ,-1\right]\cup \left[1,\infty \right)$

(vi) ${\cot }^{-1}\left(-x\right)=\pi -{\cot }^{-1}x,$ for all $x \in R$

9. Reciprocal Inverse Identities:

(i) sin-11x=cosec-1x, for all $x\in (–\infty ,–1]\cup [1,\infty )$

(ii) cos-11x=sec-1x, for all $x\in (–\infty ,–1]\cup [1,\infty )$

(iii) $\mathrm{t}\mathrm{a}{\mathrm{n}}^{-1}\left(\frac{1}{x}\right)-\left\{\begin{array}{cc}\mathrm{c}\mathrm{o}{\mathrm{t}}^{-1}x,& x>0\\ -\pi +\mathrm{c}\mathrm{o}{\mathrm{t}}^{-1}x,& x<0\end{array}\right.$

10. Cofunction Inverse Identities:

(i) $\mathrm{s}\mathrm{i}{\mathrm{n}}^{-1}x+\mathrm{c}\mathrm{o}{\mathrm{s}}^{-1}x=\frac{\mathrm{\pi }}{2}$, for all $x \in [–1,1]$

(ii) $\mathrm{t}\mathrm{a}{\mathrm{n}}^{-1}x+\mathrm{c}\mathrm{o}{\mathrm{t}}^{-1}x=\frac{\mathrm{\pi }}{2}$, for all $x\in R$

(iii) $\mathrm{s}\mathrm{e}{\mathrm{c}}^{-1}x+{\text{cosec}}^{-1}x=\frac{\mathrm{\pi }}{2}$, for all $x\in (–\infty ,–1]\cup [1,\infty )$

11. Formulae to find sum or difference of ${\mathbf{tan}}^{\mathbf{-}\mathbf{1}}\mathbf{x}$ and ${\mathbf{tan}}^{\mathbf{-}\mathbf{1}}\mathbf{y}$:

(i) $\mathrm{t}\mathrm{a}{\mathrm{n}}^{-1}x+\mathrm{t}\mathrm{a}{\mathrm{n}}^{-1}y=\left\{\begin{array}{cc}\mathrm{t}\mathrm{a}{\mathrm{n}}^{-1}\left(\frac{x+y}{1-xy}\right),& \text{\hspace{0.17em}\hspace{0.17em}if\hspace{0.17em}}xy<1\\ \pi +\mathrm{t}\mathrm{a}{\mathrm{n}}^{-1}\left(\frac{x+y}{1-xy}\right),& \text{if} x>0,y>0\text{\hspace{0.17em}\hspace{0.17em}and\hspace{0.17em}\hspace{0.17em}}xy>1\\ -\pi +\mathrm{t}\mathrm{a}{\mathrm{n}}^{-1}\left(\frac{x+y}{1-xy}\right),& \text{\hspace{0.17em}\hspace{0.17em}if} x<0,y<0\text{\hspace{0.17em}\hspace{0.17em}and\hspace{0.17em}\hspace{0.17em}}xy>1\end{array}\right.$

(ii) $\mathrm{t}\mathrm{a}{\mathrm{n}}^{-1}x-\mathrm{t}\mathrm{a}{\mathrm{n}}^{-1}y=\left\{\begin{array}{cc}\mathrm{t}\mathrm{a}{\mathrm{n}}^{-1}\left(\frac{x-y}{1+xy}\right),& \text{\hspace{0.17em}\hspace{0.17em}if} xy>-1\\ \pi +\mathrm{t}\mathrm{a}{\mathrm{n}}^{-1}\left(\frac{x-y}{1+xy}\right),& \text{\hspace{0.17em}if\hspace{0.17em}\hspace{0.17em}}x>0,y<0 \text{and} xy<-1\\ -\pi +\mathrm{t}\mathrm{a}{\mathrm{n}}^{-1}\left(\frac{x-y}{1+xy}\right),& \text{\hspace{0.17em}if\hspace{0.17em}\hspace{0.17em}}x<0,y>0 \text{and} xy<-1\end{array}\right.$

12. Formulae to find sum or difference of ${\mathbf{sin}}^{\mathbf{-}\mathbf{1}}\mathbf{x}$ and ${\mathbf{sin}}^{\mathbf{-}\mathbf{1}}\mathbf{y}$:

(i) ${\sin }^{-1}x+{\sin }^{-1}y=\left\{\begin{array}{cc}\mathrm{s}\mathrm{i}{\mathrm{n}}^{-1}\left\{x\sqrt{1-y^2}+y\sqrt{1-x^2}\right\},& \begin{array}{c}\text{if} -1\le x,y\le 1 \text{and} x^2+y^2\le 1\\ \text{or}\\ \text{if} xy<0 \text{and} x^2+y^2>1\end{array}\\ \pi -\mathrm{s}\mathrm{i}{\mathrm{n}}^{-1}\left\{x\sqrt{1-y^2}+y\sqrt{1-x^2}\right\},& \text{if} 0<x,y\le 1\text{ and }{x}^2+y^2>1\\ -\pi -\mathrm{s}\mathrm{i}{\mathrm{n}}^{-1}\left\{x\sqrt{1-y^2}+y\sqrt{1-x^2}\right\},& \text{if }-1\le x,y<0\text{ and }{x}^2+y^2>1\end{array}\right.$

(ii) ${\sin }^{-1}x-{\sin }^{-1}y=\left\{\begin{array}{cc}{\sin }^{-1}\left\{x\sqrt{1-y^2}-y\sqrt{1-x^2}\right\},& \begin{array}{c}\text{if} -1\le x,y\le 1 \text{and} x^2+y^2\le 1\\ \text{or}\\ \text{if} xy<0 \text{and} x^2+y^2>1\end{array}\\ \pi -{\sin }^{-1}\left\{x\sqrt{1-y^2}-y\sqrt{1-x^2}\right\},& \text{if} 0 < x\le 1,-1\le y\le 0\text{ and }{x}^2+y^2>1\\ -\pi -{\sin }^{-1}\left\{x\sqrt{1-y^2}-y\sqrt{1-x^2}\right\}& \text{if }-1\le x<0, 0<y\le 1\text{ and }{x}^2+y^2>1\end{array}\right.$

13. Formulae to find sum or difference of ${\mathbf{cos}}^{\mathbf{-}\mathbf{1}}\mathbf{x}$ and ${\mathbf{cos}}^{\mathbf{-}\mathbf{1}}\mathbf{y}$:

(i) ${\cos }^{-1}x+{\cos }^{-1}y=\left\{\begin{array}{cc}{\cos }^{-1}\left\{xy-\sqrt{1-x^2}\sqrt{1-y^2}\right\},& \text{if }-1\le x,y\le 1\text{ and }x+y\ge 0\\ 2\pi -{\cos }^{-1}\left\{xy-\sqrt{1-x^2}\sqrt{1-y^2}\right\},& \text{if }-1\le x,y\le 1\text{ and }x+y\le 0\end{array}\right.$

(ii) ${\cos }^{-1}x-{\cos }^{-1}y=\left\{\begin{array}{cc}{\cos }^{-1}\left\{xy+\sqrt{1-x^2}\sqrt{1-y^2}\right\},& \text{if }-1\le x,y\le 1\text{ and }x\le y\\ -{\cos }^{-1}\left\{xy+\sqrt{1-x^2}\sqrt{1-y^2}\right\},& \text{if }-1\le y\le 0,0<x\le 1\text{ and }x\ge y\end{array}\right.$

14. Formula of $\mathbf{2}{\mathbf{sin}}^{\mathbf{-}\mathbf{1}}\mathbf{x}$:

$2\mathrm{s}\mathrm{i}{\mathrm{n}}^{-1}x=\left\{\begin{array}{cc}\mathrm{s}\mathrm{i}{\mathrm{n}}^{-1}\left(2x\sqrt{1-x^2}\right),& \text{if }-\frac{1}{\sqrt{2}}\le x\le \frac{1}{\sqrt{2}}\\ \pi -\mathrm{s}\mathrm{i}{\mathrm{n}}^{-1}\left(2x\sqrt{1-x^2}\right),& \text{if }\frac{1}{\sqrt{2}}\le x\le 1\\ -\pi -\mathrm{s}\mathrm{i}\mathrm{n}\left(2x\sqrt{1-x^2}\right),& \text{if }-1\le x\le -\frac{1}{\sqrt{2}}\end{array}\right.$

15. Formula of $\mathbf{3}{\mathbf{sin}}^{\mathbf{-}\mathbf{1}}\mathbf{x}$:

$3\mathrm{s}\mathrm{i}{\mathrm{n}}^{-1}x=\left\{\begin{array}{cc}\mathrm{s}\mathrm{i}{\mathrm{n}}^{-1}\left(3x-4x^3\right),& \text{if }-\frac{1}{2}\le x\le \frac{1}{2}\\ \pi -\mathrm{s}\mathrm{i}{\mathrm{n}}^{-1}\left(3x-4x^3\right),& \text{if }\frac{1}{2}<x\le 1\\ -\pi -\mathrm{s}\mathrm{i}{\mathrm{n}}^{-1}\left(3x-4x^3\right),& \text{if }-1\le x<-\frac{1}{2}\end{array}\right.$

16. Formula of $\mathbf{2}{\mathbf{cos}}^{\mathbf{-}\mathbf{1}}\mathbf{x}$

$2\mathrm{c}\mathrm{o}{\mathrm{s}}^{-1}x=\left\{\begin{array}{cc}\mathrm{c}\mathrm{o}{\mathrm{s}}^{-1}\left(2x^2-1\right),& \text{if }0\le x\le 1\\ 2\pi -\mathrm{c}\mathrm{o}{\mathrm{s}}^{-1}\left(2x^2-1\right),& \text{if }-1\le x\le 0\end{array}\right.$

17. Formula of $\mathbf{3}{\mathbf{cos}}^{\mathbf{-}\mathbf{1}}\mathbf{x}$:

$3\mathrm{c}\mathrm{o}{\mathrm{s}}^{-1}x=\left\{\begin{array}{cc}\mathrm{c}\mathrm{o}{\mathrm{s}}^{-1}\left(4x^3-3x\right),& \text{if }\frac{1}{2}\le x\le 1\\ 2\pi -\mathrm{c}\mathrm{o}{\mathrm{s}}^{-1}\left(4x^3-3x\right),& \text{if }-\frac{1}{2}\le x\le \frac{1}{2}\\ 2\pi +\mathrm{c}\mathrm{o}{\mathrm{s}}^{-1}\left(4x^3-3x\right),& \text{if }-1\le x\le -\frac{1}{2}\end{array}\right.$

18. Formula of $\mathbf{2}{\mathbf{tan}}^{\mathbf{-}\mathbf{1}}\mathbf{x}$ in terms of ${\mathbf{tan}}^{\mathbf{-}\mathbf{1}}\mathbf{x}$:

$2\mathrm{t}\mathrm{a}{\mathrm{n}}^{-1}x=\left\{\begin{array}{cc}\mathrm{t}\mathrm{a}{\mathrm{n}}^{-1}\left(\frac{2x}{1-x^2}\right),& \text{i}\text{f }-1<x<1\\ \pi +\mathrm{t}\mathrm{a}{\mathrm{n}}^{-1}\left(\frac{2x}{1-x^2}\right),& \text{if }x>1\\ -\pi +\mathrm{t}\mathrm{a}{\mathrm{n}}^{-1}\left(\frac{2x}{1-x^2}\right),& \text{if }x<-1\end{array}\right.$

19. Formula of $\mathbf{3}{\mathbf{tan}}^{\mathbf{-}\mathbf{1}}\mathbf{x}$:

$3\mathrm{t}\mathrm{a}{\mathrm{n}}^{-1}x=\left\{\begin{array}{cc}\mathrm{t}\mathrm{a}{\mathrm{n}}^{-1}\left(\frac{3x-x^3}{1-3x^2}\right),& \text{if }-\frac{1}{\sqrt{3}}<x<\frac{1}{\sqrt{3}}\\ \pi +\mathrm{t}\mathrm{a}{\mathrm{n}}^{-1}\left(\frac{3x-x^3}{1-3x^2}\right),& \text{if }x>\frac{1}{\sqrt{3}}\\ -\pi +\mathrm{t}\mathrm{a}{\mathrm{n}}^{-1}\left(\frac{3x-x^3}{1-3x^2}\right),& \text{if }x<-\frac{1}{\sqrt{3}}\end{array}\right.$

20. Formula of $\mathbf{2}{\mathbf{tan}}^{\mathbf{-}\mathbf{1}}\mathbf{x}$ in terms of ${\mathbf{sin}}^{\mathbf{-}\mathbf{1}}\mathbf{x}$ and ${\mathbf{cos}}^{\mathbf{-}\mathbf{1}}\mathbf{x}$

(i) $2\mathrm{t}\mathrm{a}{\mathrm{n}}^{-1}x=\left\{\begin{array}{cc}\mathrm{s}\mathrm{i}{\mathrm{n}}^{-1}\left(\frac{2x}{1+x^2}\right),& \text{if} -1\le x\le 1\\ \pi -\mathrm{s}\mathrm{i}{\mathrm{n}}^{-1}\left(\frac{2x}{1+x^2}\right),& \text{if} x>1\\ -\pi -\mathrm{s}\mathrm{i}{\mathrm{n}}^{-1}\left(\frac{2x}{1+x^2}\right),& \text{if} x<-1\end{array}\right.$

(ii) $2\mathrm{t}\mathrm{a}{\mathrm{n}}^{-1}x=\left\{\begin{array}{cc}\mathrm{c}\mathrm{o}{\mathrm{s}}^{-1}\left(\frac{1-x^2}{1+x^2}\right)& ,\text{\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}if\hspace{0.17em}\hspace{0.17em}}0\le x<\mathrm{\infty }\\ -\mathrm{c}\mathrm{o}{\mathrm{s}}^{-1}\left(\frac{1-x^2}{1+x^2}\right)& ,\text{\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}if\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}}-\mathrm{\infty }<x\le 0\end{array}\right.$

21. Relation between different inverse trigonometric functions:

(i) sin-1x=cos-11-x2=tan-1x1-x2$=\mathrm{c}\mathrm{o}{\mathrm{t}}^{-1}\frac{\sqrt{1-x^2}}{x}=\mathrm{s}\mathrm{e}{\mathrm{c}}^{-1}\left(\frac{1}{\sqrt{1-x^2}}\right)={\mathrm{cosec}}^{-1}\left(\frac{1}{x}\right)$ $, (0<x<1)$

(ii) cos-1x=sin-11-x2=tan-11-x2x$=\mathrm{c}\mathrm{o}{\mathrm{t}}^{-1}\left(\frac{x}{\sqrt{1-x}}\right)=\mathrm{s}\mathrm{e}{\mathrm{c}}^{-1}\frac{1}{x}={\mathrm{cosec}}^{-1}\left(\frac{1}{\sqrt{1-x^2}}\right)$ $, (0<x<1)$

(iii) $\mathrm{t}\mathrm{a}{\mathrm{n}}^{-1}x=\mathrm{s}\mathrm{i}{\mathrm{n}}^{-1}\left(\frac{x}{\sqrt{1+x^2}}\right)=\mathrm{c}\mathrm{o}{\mathrm{s}}^{-1}\left(\frac{1}{\sqrt{1+x^2}}\right)$$=\mathrm{c}\mathrm{o}{\mathrm{t}}^{-1}\left(\frac{1}{x}\right)=\mathrm{s}\mathrm{e}{\mathrm{c}}^{-1}\sqrt{1+x^2}={\mathrm{cosec}}^{-1}\left(\frac{\sqrt{1+x^2}}{x}\right)$ $, (x>0)$