Find two different ways of describing the combination of transformations that maps the graph of $f\left(x\right)=\sqrt{x}$ onto the graph $g\left(x\right)=\sqrt{-x-2}$ and sketch the graphs of $y=\mathrm{f}\left(x\right)$ and $y=\mathrm{g}\left(x\right)$.

Functions $\mathrm{f}$ and $\mathrm{g}$ are defined for $x\in \mathrm{ℝ}$ by:

$\mathrm{f}:x\mapsto 3x-1$

$\mathrm{g}:x\mapsto 5x-x^2$

Express $\mathrm{g}f\left(x\right)$ in the form $a-b(x-c{)}^2$, where $a,b \& c$ are constants.

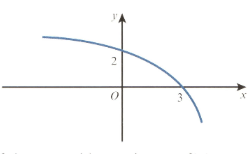
The diagram shows a sketch of the curve with equation $y=\mathrm{f}\left(x\right)$.

(b) Describe fully a sequence of two transformations that maps the graph of $y=f\left(x\right)$ onto the graph of $y=f(3-x)$

A curve has equation $y=x^2+6x+8$.

Sketch the curve, showing the coordinates of any axes crossing points.

The function $f:x\mapsto x^2-2$ is defined for the domain $x⩾0$.

(a) Find $f^{-1}\left(x\right)$ and state the domain of $f^{-1}$.

The function $f:x\mapsto x^2-2$ is defined for the domain $x⩾0$.

On the same diagram, sketch the graphs of $\mathrm{f}$(x) and ${\mathrm{f}}^{-1}$(x).