If at $t=0$, a travelling wave pulse on a string is described by the function, $y=\frac{10}{\left(x^2+2\right)}$ where $x$ and $y$ are in meters and $t$ in seconds, what will be the wave function representing the pulse at time $t$ if the pulse is propagating along the positive $x$-axis with speed $2 \mathrm{m} {\mathrm{s}}^{-1}$?

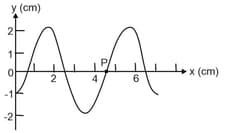
Consider a sinusoidal travelling wave shown in the figure. The wave velocity is $+40 \mathrm{cm} {\mathrm{s}}^{-1}$.

Find,

(a) the frequency
(b) the phase difference between points which are $2.5 \mathrm{cm}$ apart.
(c) how long it takes for the phase at a given position to change by $60°$.
(d) the velocity of a particle at point $P$ at the instant shown.

The equation of a travelling wave is, $y(x,t)=0.02\sin \left(\frac{x}{0.05}+\frac{t}{0.01}\right)\mathrm{m}$.
Find,
(a) the wave velocity and
(b) the particle velocity at $x=0.2 \mathrm{m}$ and $t=0.3 \mathrm{s}$.
Given, $\cos \left(\mathrm{\theta }\right)=-0.85$ where, $\mathrm{\theta }=34 \mathrm{rad}$.

Transverse waves on a string have wave speed $12.0 \mathrm{m} {\mathrm{s}}^{-1}$, amplitude $0.05 \mathrm{m}$ and wavelength $0.4 \mathrm{m}$. The waves travel in the $X-$direction and at $t=0$, the $x=0$ end of the string has zero displacement and is moving upwards.

(a) Write a wave function describing the wave.
(b) Find the transverse displacement of a point at $x=0.25 \mathrm{m}$ at time, $t=0.15 \mathrm{s}$.
(c) How much time must elapse from the instant in part (b) until the point at $x=0.25$ $\mathrm{m}$ has zero displacement?

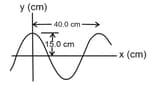
A sinusoidal wave traveling in the positive $x$-direction has an amplitude of $15.0 \mathrm{cm}$, a wavelength of $40.0 \mathrm{cm}$ and a frequency of $8.00 \mathrm{Hz}$. The vertical displacement of the medium at $t=0$ and $x=0$ is also $15.0 \mathrm{cm}$ as shown in figure.

(a) Find the angular wave number $k$, period $T$, angular frequency $\mathrm{\omega }$ and speed $v$ of the wave.
(b) Write a general expression for the wave function.

A loop of rope is whirled at a high angular velocity $\omega$ so that it becomes a taut circle of radius $R$. A kink develops in the whirling rope.

(a) Show that the speed of the kink in the rope is, $v=\omega R$.
(b) Under what conditions does the kink remain stationary relative to an observer on the ground?