Identify the correct labels of A , B and C in the following graph from the options given below: Root mean square speed V r m s ; most proable speed V m p ; Average speed V a v

A - V mp ; B - V av ; C - V rms

A - V mp ; B - V rms ; C - V av

A - V av ; B - V rms ; C - V mp

A - V rms ; B - V mp ; C - V av

For gaseous state, if most probable speed is denoted by C * , average speed by C ¯ and root mean square speed by C , then for many molecules, what is the ratios of these speeds?

C * : C - : C = 1 : 1 .128 : 1 .225

C * : C - : C = 1 : 1 .225 : 1 .128

C * : C - : C = 1 .225 : 1 .128 : 1

C * : C - : C = 1 .128 : 1 .225 : 1

MEDIUM

Earn 100

If the temperature of ${SO}_{2}$ is one fourth that of ${CH}_{4}$ , then what will be the ratio of rms speed of ${CH}_{4}$ and ${SO}_{2}$ ?

50% studentsanswered this correctly

Important Questions on States of Matter: Gases and Liquids

HARD

Chemistry>Physical Chemistry>States of Matter: Gases and Liquids>Kinetic Theory of Gases and Molecular Speeds

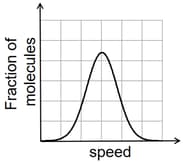
If the distribution of molecular speeds of a gas is as per the figure shown below, then the ratio of the most probable, the average, and the root mean square speeds, respectively, is

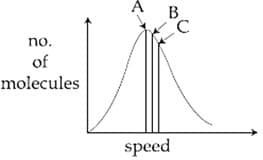
Question Image

MEDIUM

Chemistry>Physical Chemistry>States of Matter: Gases and Liquids>Kinetic Theory of Gases and Molecular Speeds

Points

I, I I

and

I I I

in the following plot respectively correspond to (

V_{m p} :

most probable velocity)

Question Image

MEDIUM

Chemistry>Physical Chemistry>States of Matter: Gases and Liquids>Kinetic Theory of Gases and Molecular Speeds

Identify the correct labels of

A, B

and

C

in the following graph from the options given below:
Question Image

Root mean square speed

(V_{r m s});

most proable speed

(V_{m p});

Average speed

(V_{a v})

MEDIUM

Chemistry>Physical Chemistry>States of Matter: Gases and Liquids>Kinetic Theory of Gases and Molecular Speeds

The kinetic energy in

J

1

mole of

N_{2}

27 ° C

is:

(R = 8.314 J {mol}^{- 1} K^{- 1})

EASY

Chemistry>Physical Chemistry>States of Matter: Gases and Liquids>Kinetic Theory of Gases and Molecular Speeds

At room temperature, the average speed of Helium is higher than that of Oxygen by a factor of:

EASY

Chemistry>Physical Chemistry>States of Matter: Gases and Liquids>Kinetic Theory of Gases and Molecular Speeds

Predict the correct order of rate of diffusion of the following molecules.

EASY

Chemistry>Physical Chemistry>States of Matter: Gases and Liquids>Kinetic Theory of Gases and Molecular Speeds

The number density of molecules of a gas depends on their distance r from the origin as,

n (r) = n_{0} e^{- α r^{4}} .

Then the numer of molecules is proportional to:

EASY

Chemistry>Physical Chemistry>States of Matter: Gases and Liquids>Kinetic Theory of Gases and Molecular Speeds

The quantity

h v / K_{B}

corresponds to

MEDIUM

Chemistry>Physical Chemistry>States of Matter: Gases and Liquids>Kinetic Theory of Gases and Molecular Speeds

The temperature at which oxygen molecules have the same root mean square speed as helium atoms have at 300 K is : (Atomic masses : He = 4 u, O = 16 u)

EASY

Chemistry>Physical Chemistry>States of Matter: Gases and Liquids>Kinetic Theory of Gases and Molecular Speeds

Initially, the root-mean-square (RMS) velocity of

N_{2}

molecules at certain temperature is

u

. If this temperature is doubled and all the nitrogen molecules dissociate into nitrogen atoms, then the new RMS velocity will be:

MEDIUM

Chemistry>Physical Chemistry>States of Matter: Gases and Liquids>Kinetic Theory of Gases and Molecular Speeds

What is the kinetic energy (in

ergs

) of the nitrogen molecule at

- 73 ° C

MEDIUM

Chemistry>Physical Chemistry>States of Matter: Gases and Liquids>Kinetic Theory of Gases and Molecular Speeds

Which of the following is not an assumption of the kinetic theory of gases?

MEDIUM

Chemistry>Physical Chemistry>States of Matter: Gases and Liquids>Kinetic Theory of Gases and Molecular Speeds

At what temperature

(

K)

, the most probable velocity of ozone gas is equal to RMS velocity of oxygen gas at

t ° C ?

EASY

Chemistry>Physical Chemistry>States of Matter: Gases and Liquids>Kinetic Theory of Gases and Molecular Speeds

The internal energy of one mole of an ideal gas is given by the expression:

MEDIUM

Chemistry>Physical Chemistry>States of Matter: Gases and Liquids>Kinetic Theory of Gases and Molecular Speeds

If the kinetic energy and

RMS

speed of a gas at a certain temperature are

4.0 kJ {mol}^{- 1}

and

5.0 \times 10^{4} cm s^{- 1}

, respectively. The molecular weight of the gas is

MEDIUM

Chemistry>Physical Chemistry>States of Matter: Gases and Liquids>Kinetic Theory of Gases and Molecular Speeds

Among the following, the plot that shows the correct marking of most probable velocity

(V_{MP}),

average velocity

(\bar{V}),

and root mean square velocity

(V_{RMS})

is :

EASY

Chemistry>Physical Chemistry>States of Matter: Gases and Liquids>Kinetic Theory of Gases and Molecular Speeds

If the r.m.s. speed of a gaseous molecule is

x m / \sec

at a pressure

p atm

, then what will be the r.m.s. speed at a pressure

2 p atm

and constant temperature ?

MEDIUM

Chemistry>Physical Chemistry>States of Matter: Gases and Liquids>Kinetic Theory of Gases and Molecular Speeds

Which of the following statement(s) (are) correct regarding the root-mean-square speed

(U_{r m s})

and average translational kinetic energy

(E_{av})

of a molecule in a gas at equilibrium?

EASY

Chemistry>Physical Chemistry>States of Matter: Gases and Liquids>Kinetic Theory of Gases and Molecular Speeds

The ratio of root-mean-square velocity of hydrogen at

50 K

to that of nitrogen at

500 K

is closest to

EASY

Chemistry>Physical Chemistry>States of Matter: Gases and Liquids>Kinetic Theory of Gases and Molecular Speeds

For gaseous state, if most probable speed is denoted by

C^{*}

, average speed by

\bar{C}

and root mean square speed by

C

, then for many molecules, what is the ratios of these speeds?

If the temperature of SO2 is one fourth that of CH4, then what will be the ratio of rms speed of CH4 and SO2?

Important Questions on States of Matter: Gases and Liquids

Learn and Prepare for any exam you want !

If the temperature of ${SO}_{2}$ is one fourth that of ${CH}_{4}$ , then what will be the ratio of rms speed of ${CH}_{4}$ and ${SO}_{2}$ ?