EASY

Earn 100

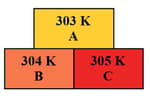
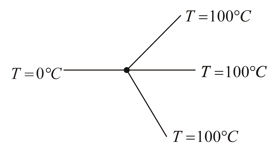
In the given diagram, the possible direction of heat energy transformation is

50% studentsanswered this correctly

Important Questions on Thermal Properties of Matter

HARD

Physics>Heat and Thermodynamics>Thermal Properties of Matter>Heat Transfer

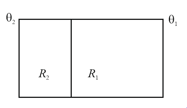
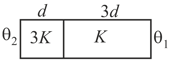
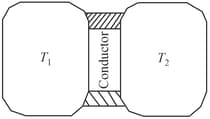
Consider a pair of insulating blocks with thermal resistances $R_{1}$ , and $R_{2}$ as shown in the figure. The temperature $θ$ at the boundary between the two blocks is

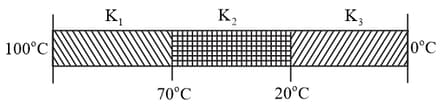
Question Image

EASY

Physics>Heat and Thermodynamics>Thermal Properties of Matter>Heat Transfer

The unit of thermal conductivity is

MEDIUM

Physics>Heat and Thermodynamics>Thermal Properties of Matter>Heat Transfer

Three rods of identical cross-section and length are made of three different materials of thermal conductivity $K_{1}, K_{2}$ and $K_{3}$ , respectively. They are joined together at their ends to make a long rod (see figure). One end of the long rod is maintained at $100 ° C$ and the other at $0 ° C$ (see figure). If the joints of the rod are at $70 ° C$ and $20 ° C$ in steady and there is no loss of energy from the surface of the rod, the correct relationship between $K_{1}, K_{2}$ and $K_{3}$ is :

Question Image

MEDIUM

Physics>Heat and Thermodynamics>Thermal Properties of Matter>Heat Transfer

Consider a ball of mass $100 g$ attached to one end of a spring $(k = 800 N m^{- 1})$ and immersed in $0.5 kg$ water. Assume the complete system is in thermal equilibrium. The spring is now stretched to $20 cm$ and the mass is released so that it vibrates up and down. Estimate the change in temperature of water before the vibrations stop.

(Specific heat of the material of the ball $= 400 J {kg}^{- 1} K^{- 1}$ and Specific heat of water $= 4200 J {kg}^{- 1} K^{- 1}$ )

HARD

Physics>Heat and Thermodynamics>Thermal Properties of Matter>Heat Transfer

Two rectangular blocks, having identical dimensions, can be arranged either in configuration

I

or in configuration

I I

as shown in the figure. One of the blocks has thermal conductivity

K

and the other

2 K

. The temperature difference between the ends along the x-axis is the same in both the configurations. It takes

9 s

to transport a certain amount of heat from the hot end to cold end in the configuration

I

. The time to transport the same amount of heat in the configuration

II

EASY

Physics>Heat and Thermodynamics>Thermal Properties of Matter>Heat Transfer

Three rods of identical cross-sectional area and made from the same metal, form the sides of an isosceles triangle

A B C

right angled at

B

as shown in figure. The point

A

and

B

are maintained at temperature

T

and

\sqrt{2} T

respectively, in the steady state. Now, assuming that only heat conduction takes place. The temperature of point

C

will be

MEDIUM

Physics>Heat and Thermodynamics>Thermal Properties of Matter>Heat Transfer

Two materials having coefficients of thermal conductivity

3 K

and

K

and thickness

d

and

3 d

respectively, are joined to form a slab as shown in the figure. The temperatures of the outer surfaces are

θ_{2}

and

θ_{1}

respectively,

(θ_{2} > θ_{1})

. The temperature at the interface is
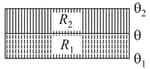
Question Image

EASY

Physics>Heat and Thermodynamics>Thermal Properties of Matter>Heat Transfer

The temperature $θ$ at the junction of two insulating sheets, having thermal resistances $R_{1}$ and $R_{2}$ as well as top and bottom temperatures $θ_{1}$ and $θ_{2}$ (as shown in figure) is given by :

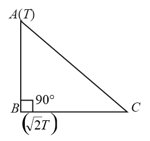
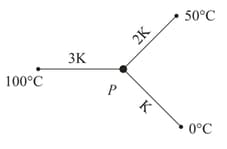
Question Image

MEDIUM

Physics>Heat and Thermodynamics>Thermal Properties of Matter>Heat Transfer

A metallic prong consists of $4$ rods made of the same material, cross-section, and same lengths as shown.

Question Image

The three forked ends are kept at $100^{o} C$ and the handle end is at $0^{o} C .$ The temperature of the junction is

EASY

Physics>Heat and Thermodynamics>Thermal Properties of Matter>Heat Transfer

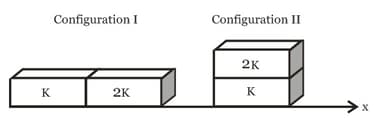
Two rods A and B of different materials are welded together as shown in figure. Their thermal conductivities are $K_{1}$ and $K_{2}$ . The thermal conductivity of the composite rod will be

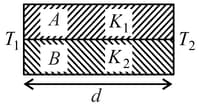
Question Image

EASY

Physics>Heat and Thermodynamics>Thermal Properties of Matter>Heat Transfer

A heat source at

T = 10^{3} K

is connected to another heat reservoir at

T = 10^{2} K

by a copper slab which is

1 m

thick. Given that the thermal conductivity of copper is

0 .1 W K^{- 1} m^{- 1}

, the energy flux through it in the steady-state is:

HARD

Physics>Heat and Thermodynamics>Thermal Properties of Matter>Heat Transfer

A thin piece of thermal conductor of constant thermal conductivity insulated on the lateral sides connects two reservoirs which are maintained at temperatures, $T_{1}$ and, $T_{2}$ as shown. Assuming that the system is in steady-state, which of the following plots best represents the dependence of the rate of change of entropy on the ratio of temperatures, $\frac{T_{1}}{T_{2}}$

Question Image

MEDIUM

Physics>Heat and Thermodynamics>Thermal Properties of Matter>Heat Transfer

Three rods of same dimensions have thermal conductivities $3 K, 2 K$ and $K$ . They are arranged as shown in the figure below. Then in the steady state the temperature of the junction $' P'$ is

Question Image

MEDIUM

Physics>Heat and Thermodynamics>Thermal Properties of Matter>Heat Transfer

Two conducting cylinders of equal length but different radii are connected in series between two heat baths kept at temperatures

T_{1} = 300 K a n d T_{2} = 100 K

as shown in the figure. The radius of the bigger cylinder is twice that of the smaller one and the thermal conductivities of the materials of the smaller and the larger cylinders are

K_{1} a n d K_{2}

, respectively. If the temperature at the junction of the two cylinders in the steady state is

200 K

, then

K_{1} / K_{2}

HARD

Physics>Heat and Thermodynamics>Thermal Properties of Matter>Heat Transfer

In steady state heat conduction, the equations that determine the heat current

j (r)

[heat flowing per unit time per unit area] and temperature

T (r)

in space are exactly the same as those governing the electric field

E (r)

and electrostatic potential

V (r)

with the equivalence given in the table below.

Heat flow	Electrostatics
$T (r)$	$V (r)$
$j (r)$	$E (r)$

We exploit this equivalence to predict the rate

Q

of total heat flowing by conduction from the surfaces of spheres of varying radii, all maintained at the same temperature. If

Q \propto R^{n},

where

R

is the radius, then the value of

n

MEDIUM

Physics>Heat and Thermodynamics>Thermal Properties of Matter>Heat Transfer

A uniform copper rod of

50 cm

length is insulated on the sides and has its ends exposed to ice and steam respectively. If there is a layer of water

1 mm

thick at each end, the temperature gradient

(in ° C m^{- 1})

in the bar is (assume that the thermal conductivity of copper is

400 W m^{- 1} K^{- 1}

and water is

0 .4 W m^{- 1} K^{- 1}

)

EASY

Physics>Heat and Thermodynamics>Thermal Properties of Matter>Heat Transfer

Two identical metal wires of thermal conductivities

K_{1}

and

K_{2}

respectively are connected in series. The effective thermal conductivity of the combination is:

HARD

Physics>Heat and Thermodynamics>Thermal Properties of Matter>Heat Transfer

Three rods of Copper, Brass and Steel are welded together to form a Y-shaped structure. Area of cross-section of each rod is

4 {cm}^{2}

. End of copper rod is maintained at

100 ° C

. Where as ends of brass and steel are kept at

0 ° C

. Lengths of the copper, brass and steel rods are

46, 13

and

12 cms

respectively. The rods are thermally insulated from surroundings except at ends. Thermal conductivities of copper, brass and steel are

0.92, 0.26

and

0.12

CGS units respectively. Rate of heat flow through copper rod is :

EASY

Physics>Heat and Thermodynamics>Thermal Properties of Matter>Heat Transfer

The two ends of a metal rod are maintained at temperatures

100 ° C

and

110 ° C

. The rate of heat flow in the rod is found to be

4.0 J s^{- 1}

. If the ends are maintained at temperatures

200 ° C

and

210 ° C

, the rate of heat flow will be:

MEDIUM

Physics>Heat and Thermodynamics>Thermal Properties of Matter>Heat Transfer

A cylinder of radius

R

is surrounded by a cylindrical shell of inner radius

R

and outer radius

2 R

. The thermal conductivity of the material of the inner cylinder is

K_{1}

and that of the outer cylinder is

K_{2}

. Assuming no loss of heat, the effective thermal conductivity of the system for heat flowing along the length of the cylinder is:

In the given diagram, the possible direction of heat energy transformation is

Important Questions on Thermal Properties of Matter

Learn and Prepare for any exam you want !