In the given figure at point . what is the direction of magnetic field.

Important Questions on Magnetic Effect of Current
[Take ]
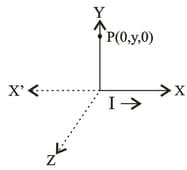
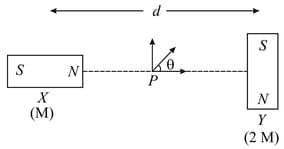
Two magnetic dipoles and are placed at a separation , with their axes perpendicular to each other. The dipole moment of is twice that of . A particle of charge is passing through their mid-point , at angle with the horizontal line, as shown in figure. What would be the magnitude of force on the particle at that instant? ( is much larger than the dimension of the dipole)
An electron enters a magnetic field of with a velocity of The acceleration produced is
( of electron )
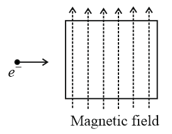
During its motion inside the chamber
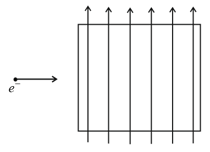
An electric field of appropriate magnitude is also applied so that the electron travels un-deviated without any change in its speed through the chamber. We are ignoring gravity. Then, the direction of the electric field is,
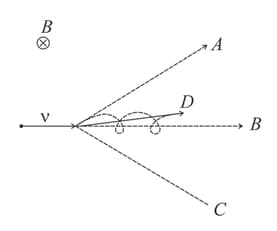
Consider a negatively charged particle moving with a velocity in a magnetic field applied perpendicular to the plane of the paper (into the paper). The particle follows the path or or or (shown in the figure)