EASY

Earn 100

Point out the differences between the free oscillation and forced oscillation.

Important Questions on Sound

HARD

Science>Physics>Sound>Free, Damped and Forced Vibrations

A simple harmonic oscillator of angular frequency

2 rad s^{- 1}

is acted upon by an external force

F = \sin t N .

If the oscillator is at rest in its equilibrium position at

t = 0,

its position at later times is proportional to:

EASY

Science>Physics>Sound>Free, Damped and Forced Vibrations

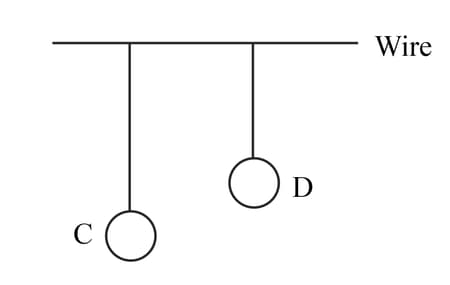
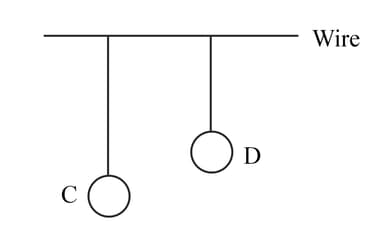
Two pendulums $C$ and $D$ are suspended from a wire as shown in the given figure. Pendulum $C$ is made to oscillate by displacing it from its mean position. It is seen that $D$ also starts oscillating. If the length of $D$ is made equal to $C$ , then what difference will you notice in the oscillations of $D$ ?

Question Image

EASY

Science>Physics>Sound>Free, Damped and Forced Vibrations

Why does the amplitude of a vibrating body continuously decrease during damped vibrations?

EASY

Science>Physics>Sound>Free, Damped and Forced Vibrations

Question Image

EASY

Science>Physics>Sound>Free, Damped and Forced Vibrations

What do you understand by free vibrations of a body?

EASY

Science>Physics>Sound>Free, Damped and Forced Vibrations

Two pendulums

C

and

D

are suspended from a wire as shown in the given figure. Pendulum

C

is made to oscillate by displacing it from its mean position. It is seen that

D

also starts oscillating. Name the type of oscillation,

C

will execute.
Question Image

MEDIUM

Science>Physics>Sound>Free, Damped and Forced Vibrations

The vibrations taking place in the diaphragm of a microphone will be

EASY

Science>Physics>Sound>Free, Damped and Forced Vibrations

If the differential equation given by

\frac{d^{2} y}{d t^{2}} + 2 k \frac{d y}{d t} + ω^{2} y = F_{0} \sin p t

Describes the oscillatory motion of body in a dissipative medium under the influence of a periodic force, then the state of maximum amplitude of the oscillation is a phenomena of

MEDIUM

Science>Physics>Sound>Free, Damped and Forced Vibrations

The amplitude of vibrations remains constant in the case of

(i)

free vibrations

(ii)

damped vibrations

(iii)

maintained vibrations

(iv)

forced vibrations

HARD

Science>Physics>Sound>Free, Damped and Forced Vibrations

A body of mass

600 g

is attached to a spring of spring constant,

k = 100 N m^{- 1}

and it is performing damped oscillations. If damping constant is

0.2

and driving force is,

F = F_{0} \cos (ω t)

, where

F_{0} = 20 N

, find the amplitude of oscillation at resonance.

EASY

Science>Physics>Sound>Free, Damped and Forced Vibrations

Vibrations of constant amplitude are possible only in _____.(Medium/Vacuum)

EASY

Science>Physics>Sound>Free, Damped and Forced Vibrations

Two springs of force constants

K_{1}

and

K_{2}

, respectively, are connected to a mass

m

, as shown. The frequency of oscillation of the mass is

f

. If both

K_{1}

and

K_{2}

are made four times their original values, the frequency of oscillation becomes

Question Image

HARD

Science>Physics>Sound>Free, Damped and Forced Vibrations

A particle, with restoring force proportional to displacement and resisting force proportional to velocity is subjected to a force.

F = F_{0} \sin ω t

If the amplitude of the particle is maximum for

ω = ω_{1}

and the energy of the particle is maximum for

ω = ω_{2}

then

EASY

Science>Physics>Sound>Free, Damped and Forced Vibrations

In case of a forced oscillation, when does the resonance peak becomes very sharp ?

MEDIUM

Science>Physics>Sound>Free, Damped and Forced Vibrations

In the case of sustained forced oscillations the amplitude of oscillations:-

HARD

Science>Physics>Sound>Free, Damped and Forced Vibrations

A light pointer fixed to one prong of a tuning fork touches a vertical plate. The fork is set vibrating at a frequency of 56 Hz and allowed to free fall. Calculate how many complete oscillation are counted when plate falls at 10 cm

EASY

Science>Physics>Sound>Free, Damped and Forced Vibrations

In case of a forced vibration, the resonance peak becomes very sharp when the

MEDIUM

Science>Physics>Sound>Free, Damped and Forced Vibrations

A metal wire of linear mass density of

9.8 g m^{- 1}

is stretched with a tension of kg-wt between two rigid supports 1 m apart. The wire passes at its middle point between the poles of a permanent magnet and it vibrates in resonance when carrying an alternating current of frequency n. the frequency n of the alternating sources is

HARD

Science>Physics>Sound>Free, Damped and Forced Vibrations

A particle of mass,

m

is attached to spring having spring constant

K

and natural angular frequency

ω_{0} .

If external force

F (t)

applied to the oscillator, is proportional to

c o s ω t (ω \neq ω_{0})

. The time displacement of the oscillator is then proportional to:

EASY

Science>Physics>Sound>Free, Damped and Forced Vibrations

The prime condition necessary for forced oscillation of a body is

Point out the differences between the free oscillation and forced oscillation.

Important Questions on Sound

Learn and Prepare for any exam you want !