HARD
9th ICSE
IMPORTANT
Earn 100
Prove that equal chords of congruent circles subtend equal angles at their centre.

Important Questions on Circle
MEDIUM
9th ICSE
IMPORTANT
MEDIUM
9th ICSE
IMPORTANT
HARD
9th ICSE
IMPORTANT
Given two equal chords and of a circle, with centre , intersecting each other at point . Prove that .
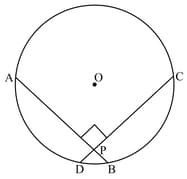
HARD
9th ICSE
IMPORTANT
Given two equal chords and of a circle, with centre , intersecting each other at point . Prove that .
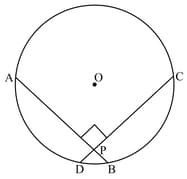
HARD
9th ICSE
IMPORTANT
HARD
9th ICSE
IMPORTANT
MEDIUM
9th ICSE
IMPORTANT
In the given figure, is the centre of the circle with radius and is perpendicular to . . If the length of is , find .
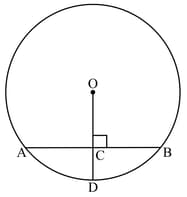
HARD
9th ICSE
IMPORTANT
In the given figure, and are two equal chords of a circle, with centre . is the mid-point of chord is the mid-point of chord and . If , find .

