HARD
10th Meghalaya Board
IMPORTANT
Earn 100
Prove that the area of the equilateral triangle described on the side of a square is half the area of the equilateral triangles described on its diagonal.

Important Questions on Triangles
MEDIUM
10th Meghalaya Board
IMPORTANT
is a triangle and is a straight line meeting in and in . If , prove that area of is one sixteenth of the area of .
MEDIUM
10th Meghalaya Board
IMPORTANT
In the given figure, and are and , respectively. Prove that . Also, find .

HARD
10th Meghalaya Board
IMPORTANT
In the given figure, in and divides the into two regions such that . Determine .

EASY
10th Meghalaya Board
IMPORTANT
Give two different examples of pair of similar figures.
HARD
10th Meghalaya Board
IMPORTANT
In the given figure, is a right-angled triangle with . Find the ratio of the area of to the area of .

HARD
10th Meghalaya Board
IMPORTANT
In the given figure, if and , then find .
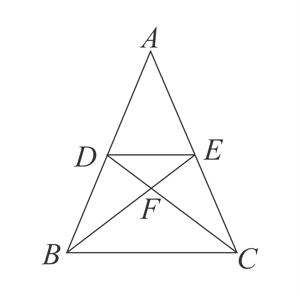
MEDIUM
10th Meghalaya Board
IMPORTANT
In the given figure, and . Find Area of Area of quadrilateral .

MEDIUM
10th Meghalaya Board
IMPORTANT
In the figure, the perpendicular from on side of a , intersects at such that . Prove that .

