HARD

Earn 100

Prove that the ratio of areas of two similar triangles is equal to the square of the ratio of their corresponding medians.

Important Questions on Theorems regarding Similarity

MEDIUM

Mathematics>Geometry>Theorems regarding Similarity>Theorems on Similarity

D

is the midpoint of side

△ A B O

△ ABC

. Point

△ D E P

lies on

AC

such that

CE = \frac{1}{3} AC

BE

and

AD

intersect at

G

. What is

\frac{AG}{GD}

MEDIUM

Mathematics>Geometry>Theorems regarding Similarity>Theorems on Similarity

The area of

△ ABC

44 {cm}^{2}

. If D is the midpoint of BC and E is the midpoint of AB. Then the area in

{cm}^{2}

△ BDE

will be:

MEDIUM

Mathematics>Geometry>Theorems regarding Similarity>Theorems on Similarity

△ PQR

∠ Q

= 85 °

and

∠ R

= 65 °

. Point S and T are on the sides

PQ

and

PR

, respectively such that

∠ STR

= 95 °

, and the ratio of the

QR

and

ST

9 : 5

. If

PQ = 21.6 cm

, then the length of

PT

is:

EASY

Mathematics>Geometry>Theorems regarding Similarity>Theorems on Similarity

[

and

BC = 14 cm . P

is the point on

AB

such that

CP = 11.2 cm

and

∠ ACP = ∠ B

. What is the length (in cm) of

BP

HARD

Mathematics>Geometry>Theorems regarding Similarity>Theorems on Similarity

Let $A B C$ be a triangle and $M$ be a point on side $A C$ closer to vertex $C$ than $A$ . Let $N$ be a point on side $A B$ such that $M N$ is parallel to $B C$ and let $P$ be a point on side $B C$ such that $M P$ is parallel to $A B$ . If the area of the quadrilateral $B N M P$ is equal to $\frac{5}{18}$ of the area of $Δ A B C$ , then the ratio $A M / M C$ equals

EASY

Mathematics>Geometry>Theorems regarding Similarity>Theorems on Similarity

In the given figure, if $D E ∥ B C, A D = 2.5 c m, D B = 3.5 c m$ and $E C = 4.2 c m$ , then the measure of $A C$ is:

Question Image

HARD

Mathematics>Geometry>Theorems regarding Similarity>Theorems on Similarity

In a

△

ABC, MN

∥

BC, the area of quadrilateral MBCN is equal to

130 {cm}^{2}

. If AN

:

NC is

4 : 5

, then the area of

△

AMN is:

EASY

Mathematics>Geometry>Theorems regarding Similarity>Theorems on Similarity

△ A B C ~ △ P Q R

\frac{a r (A B C)}{a r (P Q R)} = \frac{9}{4}

A C = 12 cm, A B = 18 cm

and

B C = 15 cm

then

P R

is equal to _____.

MEDIUM

Mathematics>Geometry>Theorems regarding Similarity>Theorems on Similarity

Suppose

B C

is a given line segment in the plane and

T

is a scalene triangle. The number of points

A

in the plane such that the triangle with vertices

A, B, C

(in same order) is similar to triangle

T

MEDIUM

Mathematics>Geometry>Theorems regarding Similarity>Theorems on Similarity

△ A B C ~ △ R Q P

and

A B = 4 c m, B C = 6 c m

and

A C = 5 c m

. If

a r (△ A B C) : a r (△ P Q R) = 9 : 4

, then

P Q

is equal to:

MEDIUM

Mathematics>Geometry>Theorems regarding Similarity>Theorems on Similarity

Let

△ ABC ~ △ RPQ

and

\frac{ar △ ABC}{ar △ RPQ} = \frac{1}{4}

. If PQ

= 4

cm,QR

= 6

cm and PR

= 7

cm, then AC is equal to_____.

HARD

Mathematics>Geometry>Theorems regarding Similarity>Theorems on Similarity

In a quadrilateral

A B C D,

which is not a trapezium, it is known that

∠ D A B = ∠ A B C = 60 ° .

Moreover,

∠ C A B = ∠ C B D .

Then

EASY

Mathematics>Geometry>Theorems regarding Similarity>Theorems on Similarity

The perimeter of similar triangles

ABC

and

PQR

are

78 cm

and

46.8 cm

, respectively. If

PQ = 11.7

, then the length of

AB

is______.

EASY

Mathematics>Geometry>Theorems regarding Similarity>Theorems on Similarity

∆ P Q R, P Q = 24 c m

and

∠ Q = 58 °

S

and

T

are the points on the side

P Q

and

P R

, respectively such that

∠ S T R = 122 °

. If

P S = 14 c m & P T = 12 c m

, then the length of

R T

is:

MEDIUM

Mathematics>Geometry>Theorems regarding Similarity>Theorems on Similarity

∆ ABC, D

and

E

are the points on sides

AB

and

BC

respectively such that

DE ∥ AC

. If

AD : DB = 5 : 3

, then what is the ratio of the area of

∆ BDE

to that of the trapezium

ACED

EASY

Mathematics>Geometry>Theorems regarding Similarity>Theorems on Similarity

∆ A B C, D

is a point on side

B C

such that

∠ ADC = ∠ BAC

. If

C A = 12 cm, C B = 8 cm

then

C D

is equal to:

HARD

Mathematics>Geometry>Theorems regarding Similarity>Theorems on Similarity

Let

A B C

be a triangle with sides

51, 52, 53 .

Let

Ω

denote the incircle of

∆ A B C .

Draw tangents to

Ω

which are parallel to the sides of

A B C .

Let

r_{1}, r_{2}, r_{3}

be the inradii of the three corner triangles so formed. Find the largest integer that does not exceed

r_{1} + r_{2} + r_{3} .

EASY

Mathematics>Geometry>Theorems regarding Similarity>Theorems on Similarity

Consider the following figure shown below and choose which of the following equation is CORRECT about the similarity of both triangles?

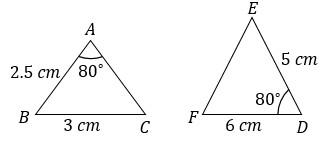
Question Image

HARD

Mathematics>Geometry>Theorems regarding Similarity>Theorems on Similarity

Let

C_{1}, C_{2}

be two circles touching each other externally at the point

A

and let

A B

be the diameter of circle

C_{1}

. Draw a secant

B A_{3}

to circle

C_{2}

, intersecting circle

C_{1}

at a point

A_{1} (\neq A),

and circle

C_{2}

at points

A_{2}

and

A_{3}

. If

B A_{1} = 2, B A_{2} = 3

and

B A_{3} = 4

then the radii of circles

C_{1}

and

C_{2}

are respectively

HARD

Mathematics>Geometry>Theorems regarding Similarity>Theorems on Similarity

In a triangle $A B C$ with $∠ A < ∠ B < ∠ C,$ points $D, E, F$ are on the interior of segments $B C, C A, A B$ respectively. Which of the following triangles cannot be similar to the triangle $A B C$ ?

Prove that the ratio of areas of two similar triangles is equal to the square of the ratio of their corresponding medians.

Important Questions on Theorems regarding Similarity

Learn and Prepare for any exam you want !