EASY
Earn 100
The to ratio in a certain piece of wood is 25% of that in atmosphere. The half life period of is 5580 years. The age of wood piece is
(a)5580 years
(b)2790 years
(c)1395 years
(d)11160 years
50% studentsanswered this correctly
Important Questions on Nuclei
MEDIUM
MEDIUM
[This question was awarded a bonus and proper correction was made to avoid that]
MEDIUM
In the decay sequence:
and are the particles/ radiation emitted by the respective isotopes. The correct option(s) is/are:
HARD
EASY
MEDIUM
MEDIUM
EASY
The variation of decay rate with number of active nuclei is correctly shown in graph
A 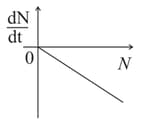
B 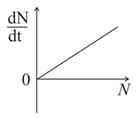
C 
D 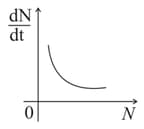
EASY
MEDIUM
MEDIUM
MEDIUM
EASY
MEDIUM
MEDIUM
EASY
EASY
EASY
HARD
MEDIUM

