MEDIUM
Earn 100
The L.P.P. to maximize subject to has
(a)unbounded solutions
(b)no solution
(c)a unique solution
(d)infinite solutions.
16.33% studentsanswered this correctly
Important Questions on Linear Programming
MEDIUM
HARD
EASY
EASY
EASY
EASY
MEDIUM
HARD
A furniture trader deals in only two items - chairs and tables. He has rupees to invest and a space to store at most items. A chair costs him rupees and a table costs him rupees . The trader earns a profit of rupees and rupees on a chair and table, respectively. Formulate the above problem as an LPP to maximise the profit and solve it graphically.
HARD
EASY
HARD
HARD
The maximum value of subject to is
MEDIUM
Minimize Subject to : and
Which one of the following points lies outside the feasible region?
EASY
The feasible region of an is shown in the figure. If , then the minimum value of occurs at
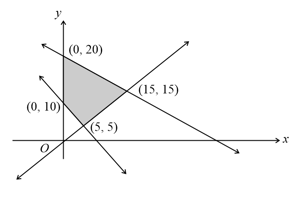
MEDIUM
EASY
Solve graphically the following linear programming problem:
Maximize or minimize subject to constraints .
EASY
Solve graphically the following linear programming problem:
Maximize or minimize subject to constraints .
MEDIUM
EASY
HARD

