The function $f:\left(0,\infty \right)\to R$ defined as $f\left(x\right)=x^2+\ln x$ which of the following option is correct about $f$:

$f:R\to R$ defined by $$\begin{gathered} f\left(x\right)=\left\{\begin{array}{l}2x; x>3 \\ {x}^2; 1<x\le 3\\ 3x; x\le 1\end{array}\right. \end{gathered}$$, then $f(-2)+f\left(3\right)+f\left(4\right)$ is

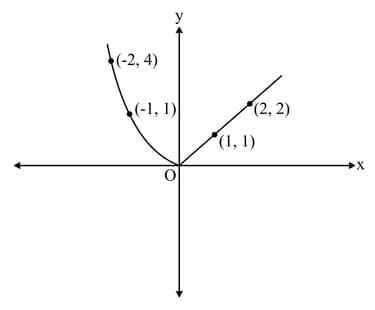
Write the function whose graph is shown below.

Let $R$ be the set of real numbers and $f:R\to R$ be defined by $f\left(x\right)=\frac{\left\{x\right\}}{1+{\left[x\right]}^2},$ where $\left[x\right]$ is the greatest integer less than or equal to $x,$ and $\left\{x\right\}=x-\left[x\right].$ Which of the following statement are true?

I. The range of $f$ is closed interval
II. $f$ is continuous on $R$
III. $f$ is one-one on $R$

The value of function $f\left(x\right)=x^3-3x^2+1$ at $x=0$ will be

A function $f:\left[-7,6\right]\to R$ is defined as follows

$f\left(x\right)=\left\{\begin{array}{ll}{x}^2+2x+1& ; -7\le x<-5\\ x+5& ; -5\le x\le 2\\ x-1& ; 2<x<6\end{array}\right.$

find $\frac{4f\left(-3\right)+2f\left(4\right)}{f\left(-6\right)-3f\left(1\right)}$

A cuboid with a square base and given volume $V$ is shown in the figure.

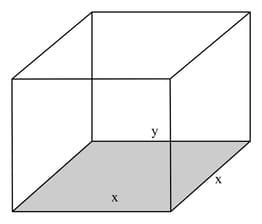
Express the surface area $S$ as a function of $x$.

A function $f:\left[-7,6\right]\to R$ is defined as follows

$f\left(x\right)=\left\{\begin{array}{ll}{x}^2+2x+1& ; -7\le x<-5\\ x+5& ; -5\le x\le 2\\ x-1& ; 2<x<6\end{array}\right.$

find $f\left(-7\right)-f\left(-3\right)$