Given below are two statements. One is labelled as Assertion A and the other is labelled as Reason R . Assertion A : If d Q and d W represent the heat supplied to the system and the work done on the system respectively. Then according to the first law of thermodynamics d Q = d U - d W . Reason R : First law of thermodynamics is based on law of conservation of energy. In the light of the above statements, choose the correct answer from the option given below :

Both A and R are correct and R is the correct explanation of A

A is correct but R is not correct

A is not correct but R is correct

Both A and R are correct but R is not the correct explanation of A

HARD

JEE Main

IMPORTANT

Earn 100

The specific heats, $C_{p}$ and $C_{v}$ of a gas of diatomic molecules, $A,$ are given (in units of $J m o l^{- 1} K^{- 1}$ ) by $29$ and $22,$ respectively. Another gas of diatomic molecules, $B,$ has the corresponding values $30$ and $21 .$ If they are treated as ideal gases, then:

52.73% studentsanswered this correctly

Important Questions on Thermodynamics

EASY

JEE Main

IMPORTANT

EMBIBE CHAPTER WISE PREVIOUS YEAR PAPERS FOR PHYSICS>Chapter 10 - Thermodynamics>JEE Main - 9th January 2019 Shift 1>Q 59

A gas can be taken from

A

B

via two different processes

ACB

and

ADB

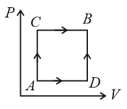
When path

ACB

is used

60 J

of heat flows into the system and

30 J

of work is done by the system. If the path

ADB

is used then work done by the system is

10 J

, the heat flows into the system in the path

ADB

is:

EASY

JEE Main

IMPORTANT

EMBIBE CHAPTER WISE PREVIOUS YEAR PAPERS FOR PHYSICS>Chapter 10 - Thermodynamics>JEE Main - 10th April 2019 Shift 1>Q 62

n

moles of an ideal gas with constant volume heat capacity

C_{v}

undergo an isobaric expansion by certain volume. The ratio of the work done in the process, to the heat supplied is:

MEDIUM

JEE Main

IMPORTANT

EMBIBE CHAPTER WISE PREVIOUS YEAR PAPERS FOR PHYSICS>Chapter 10 - Thermodynamics>JEE Main - 10th April 2019 Shift 1>Q 63

A cylinder with fixed capacity of

67.2

litre contains helium gas at STP. The amount of heat needed to raise the temperature of the gas by

20 ° C

is:
[Given that

R = 8.31 J m o l^{- 1} K^{- 1}]

MEDIUM

JEE Main

IMPORTANT

EMBIBE CHAPTER WISE PREVIOUS YEAR PAPERS FOR PHYSICS>Chapter 10 - Thermodynamics>JEE Main - 10th April 2019 Shift 2>Q 64

When heat

Q

is supplied to a diatomic gas of rigid molecules, at constant volume its temperature increases by

∆ T

. The heat required to produce the same change in temperature, at a constant pressure is:

EASY

JEE Main

IMPORTANT

EMBIBE CHAPTER WISE PREVIOUS YEAR PAPERS FOR PHYSICS>Chapter 10 - Thermodynamics>JEE Main - 10th January 2019 Shift 2>Q 67

Half mole of an ideal monoatomic gas is heated at a constant pressure of

1 atm

from

20 ° C

90 ° C

. Work done by the gas is

(

Gas constant,

R = 8.21 J {mol}^{- 1} K^{- 1}

)

MEDIUM

JEE Main

IMPORTANT

EMBIBE CHAPTER WISE PREVIOUS YEAR PAPERS FOR PHYSICS>Chapter 10 - Thermodynamics>JEE Main - 12th April 2019 Shift 1>Q 68

Two moles of helium gas is mixed with three moles of hydrogen molecules (taken to be rigid). What is the molar specific heat of mixture at constant volume?

(R = 8.3 J / m o l K)

MEDIUM

JEE Main

IMPORTANT

EMBIBE CHAPTER WISE PREVIOUS YEAR PAPERS FOR PHYSICS>Chapter 10 - Thermodynamics>JEE Main - 12th April 2019 Shift 1>Q 69

A sample of an ideal gas is taken through the cyclic process

a b c a

as shown in the figure. The change in the internal energy of the gas along the path

c a

- 180 J .

The gas absorbs

250 J

of heat along the path

a b

and

60 J

along the path

b c

. The work done by the gas along the path

a b c

is:

MEDIUM

JEE Main

IMPORTANT

EMBIBE CHAPTER WISE PREVIOUS YEAR PAPERS FOR PHYSICS>Chapter 10 - Thermodynamics>JEE Main - 12th January 2019 Shift 1>Q 73

An ideal monoatomic gas occupies a volume of

2 m^{3}

at a pressure of

3 \times 10^{6} Pa .

The energy of the gas is:

The specific heats, Cp and Cv of a gas of diatomic molecules, A, are given (in units of J mol-1 K-1 ) by 29 and 22, respectively. Another gas of diatomic molecules, B, has the corresponding values 30 and 21. If they are treated as ideal gases, then:

Important Questions on Thermodynamics

Learn and Prepare for any exam you want !

The specific heats, $C_{p}$ and $C_{v}$ of a gas of diatomic molecules, $A,$ are given (in units of $J m o l^{- 1} K^{- 1}$ ) by $29$ and $22,$ respectively. Another gas of diatomic molecules, $B,$ has the corresponding values $30$ and $21 .$ If they are treated as ideal gases, then: