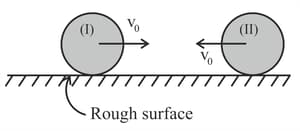
Two identical solid spheres are rolling without slipping with velocity$v_0,$ over a rough horizontal surface collides elastically as shown. The final speed of centre of mass of sphere (ii) after sufficient long time will be

Assertion $\left(A\right) :$ If the ice on the polar caps of the earth melts, then length of the day will increase.

Reason $\left(R\right) :$ Moment of inertia of earth increases, as ice on polar caps melts.

The correct answer is

A thin rod of mass $0.9 \mathrm{kg}$ and length $1 \mathrm{m}$ is suspended, at rest, from one end so that it can freely oscillate in the vertical plane. A particle of mass $0.1 \mathrm{kg}$ moving in a straight line with velocity $80 \mathrm{m} {\mathrm{s}}^{-1}$ hits the rod at its bottom most point and sticks to it (see figure). The angular speed (in $\mathrm{rad} {\mathrm{s}}^{-1}$) of the rod immediately after the collision will be …………

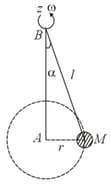
A thin rod of mass $M$ and length $a$ is free to rotate in horizontal plane about a fixed vertical axis passing through point $O$. A thin circular disc of mass $M$ and of radius $\frac{a}{4}$ is pivoted on this rod with its centre at a distance $\frac{a}{4}$ from the free end so that it can rotate freely about its vertical axis, as shown in the figure. Assume that both the rod and the disc have uniform density and they remain horizontal during the motion. An outside stationary observer finds the rod rotating with an angular velocity $\Omega$ and the disc rotating about its vertical axis with angular velocity $4\Omega$. The total angular momentum of the system about the point $O$ is $\left[\left(\frac{M{a}^2\Omega }{48}\right)n\right]$.

The value of $n$ is _________ .

A mass $M$ hangs on a massless rod of length $l$ which rotates at a constant angular frequency. The mass $M$ moves with steady speed in a circular path of constant radius. Assume that the system is in steady circular motion with constant angular velocity $\omega .$ The angular momentum of $M$ about point $A$ is $L_A$ which lies in the positive $z$ direction and the angular momentum of $M$ about $B$ is $L_B.$ The correct statement for this system is:

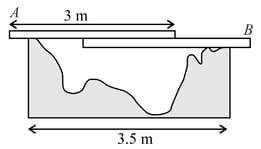
Persons $A$ and $B$ are standing on the opposite sides of a $3.5 \mathrm{m}$ wide water stream that they wish to cross. Each one of them has a rigid wooden plank whose mass can be neglected. However, each plank is only slightly longer than, $3 \mathrm{m}.$ So, they decide to arrange them together as shown in the figure schematically. With $B$ (the mass $17 \mathrm{kg}$ ) standing, the maximum mass of $A$, who can walk over the plank is close to,

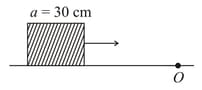
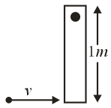
A cubical block of side $30 \mathrm{cm}$ is moving with velocity $2 \mathrm{m} {\mathrm{s}}^{-1}$ on a smooth horizontal surface. The surface has a bump at a point $O$ as shown in the figure. The angular velocity (in rad/s) of the block immediately after it hits the bump, is :